A rod of mass M and length l attached with a small bob of mass m at its end is freely rotating about a vertical axis passing through its other end with a constant angular speed ω. Find the force exerted by the system (rod and bob) on the pivot. Assume that gravity is absent.

 We select a rotating frame of reference with respect to the rod. The centrifugal force experienced by the elementary segment of length dr & mass δ m at a distance r from the axis is given by the expression
We select a rotating frame of reference with respect to the rod. The centrifugal force experienced by the elementary segment of length dr & mass δ m at a distance r from the axis is given by the expression
dF=(δm)rω2⇒ The total centrifugal force experienced by the rod
F=ω2∫r(δm)=ω2∫l0r(M/l).dr⇒F=Mω2l(r22)∫l0=Mω2l2
The centrifugal force exerted by the bob
F=mlω2
∴ The total centrifugal force =F1=F+F.=Mω2l2+mω2l
Ft=(M2+m)ω2l, (centrifugal force is a pseudo force being experienced in a non-inertial plane.)
Hence, the requried force =[M2+m]ω2l
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)14videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSERTION REASONING TYPE1videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - COMPREHENSION - IV2videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type6videos
PHYSICS PART2
- BOOK - FIITJEECHAPTER - SEMICONDUCTOR AND DEVICEEXERCISE - SOLVED PROBLEMS Objective: Level-I20videos
SEMICONDUCTOR AND DEVICE
Similar Questions
A uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA thin uniform rod of length l and mass m is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is ω. Its centre of mass rises to a maximum height of -
View Solution
Knowledge Check
- Question 1 - Select One
A uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A12mω2xB12mω2(1−x2l)C12mω2l(1−x2l2)D12mω2l[1−xl] - Question 2 - Select One
A thin uniform rod of length l and mass m is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is ω. Its centre of mass rises to a maximum height of -
A13l2ω2gB16lωgC12l2ω2gD16l2ω2g - Question 3 - Select One
A thin uniform rod of length l and mass m is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is ω. Its cenre of mass rises to a maximum height of :
A1lω6gB1l2ω2gC1l2ω26gD1l2ω23g
A uniform rod of mass M and length L with two particles m and m2 attached to its ends, is pivoted at its centre. The system rotates in a vertical plane with angular velocity ω.
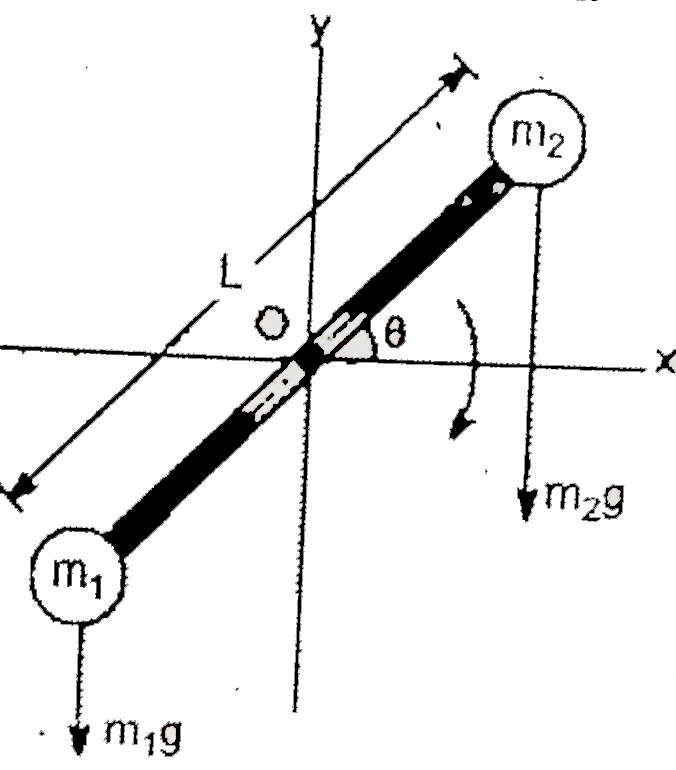 View Solution
View SolutionA uniform rod of mass m and length l0 is rotating with a constant angular speed ω about a vertical axis passing through its point of suspension. Find the moment of inertia of the rod about the axis of rotation if it make an angle θ to the vertical (axis of rotation).
View SolutionA uniform rod of mass m and length l0 is rotating with a constant angular speed ω about a vertical axis passing through its point of suspension. Find the moment of inertia of the rod about the axis of rotation if it make an angle θ to the vertical (axis of rotation).
View SolutionA rod of length l and mass m is capable of rotating freely about an axis passing through a hole at the end. The period of oscillation of this physical pendulum is
View SolutionA thin uniform rod of mass 'M' and ength 'L' is rotating about a perpendicular axis passing through its centre with a constant angular velocity 'ω'. Two objects each of mass M3 are attached gently to the two ends of the rod. The rod will now rotate with an angular velocity of
View Solution

