A rod of length l is constrained to move in a vertical plane containing its length between two smooth perpendicular planes. It is released from the vertical position by pulling its bottom gently, find its angular acceleration & angular velocity as a function of the angle θ made by it with vertical as shown in the figure.

When the rod inclines at an angle θ with vertical, let the contact forces at A & B are NA&NB respectively as shown in the figure.
⇒ Equation of motion of c.m. of the rod can be given by,
Fx=max ⇒ NA=max ...(i)
& Fy=may NB−mg=may ...(ii)
where ax&ay are the horizontal & vertical acceleration of the c.m. of the rod respectively. The moment of NA&NB anticlockwise about G is given by [G is COM of rod]
τ(G)=−NA(l/2)cosθ+NB(l/2)sinθ
⇒ τG=IGα,where IG = M.I. of the rod about G.
IG=ml2/12andα = angular accleration of the rod =d2θdt2
⇒ (−NAcosθ+NBsinθ)l/2=ml2α/12 ...(iii)

Now we have three equations and four unknowns NA,NB,ax&ay. Therefore we need another two equations by using kinematics, x = (l/2) sin θ & y = (l/2) cos θ, by differentiating x and y, we obtain
ax=d2xdt2=l2(αsinθ−ω2cosθ) ...(iv)
ay=d2ydt2=−l2(αsinθ+ω2cosθ) ...(v)
Using (i) & (iv), find NA & using (ii) & (v) find NB, then put NA&NB in equation (iii) to obtain α=(3g/2l)sinθ
Putting α=ωdωdθ we obtain,
ωdω=3g2lsinθdθ⇒∫ω0ωdω=3g2l∫θ0sinθ ⇒ω=√3g(1−cosθ)l
Alter
I=ml212+ml24 (∵y=l/2)
I=ml23
By law of energy conservation,
12Iω2=mgl2(1−cosθ)⇒ω=√3gl(1−cosθ)
α=dωdt=3g2lsinθ

Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)14videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSERTION REASONING TYPE1videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - COMPREHENSION - IV2videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type6videos
PHYSICS PART2
- BOOK - FIITJEECHAPTER - SEMICONDUCTOR AND DEVICEEXERCISE - SOLVED PROBLEMS Objective: Level-I20videos
SEMICONDUCTOR AND DEVICE
Similar Questions
A light rigid rod of length l is constrained to move in a vertical plane, so that its ends are along the x and y axes respectively. Find the instantaneous axis of rotation of the rod when it makes an angle θ with horizontal.
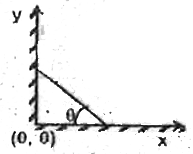 View Solution
View SolutionA light rigid rod of length l is constrained to move in a vertical plane, so that its ends are along the x and y axes respectively. Find the instantaneous axis of rotation of the rod when it makes an angle θ with horizontal.
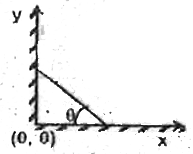 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A slender uniform rod of mass M and length l is pivoted at one ens so that it can rotate in a vertical plane, Fig. There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is
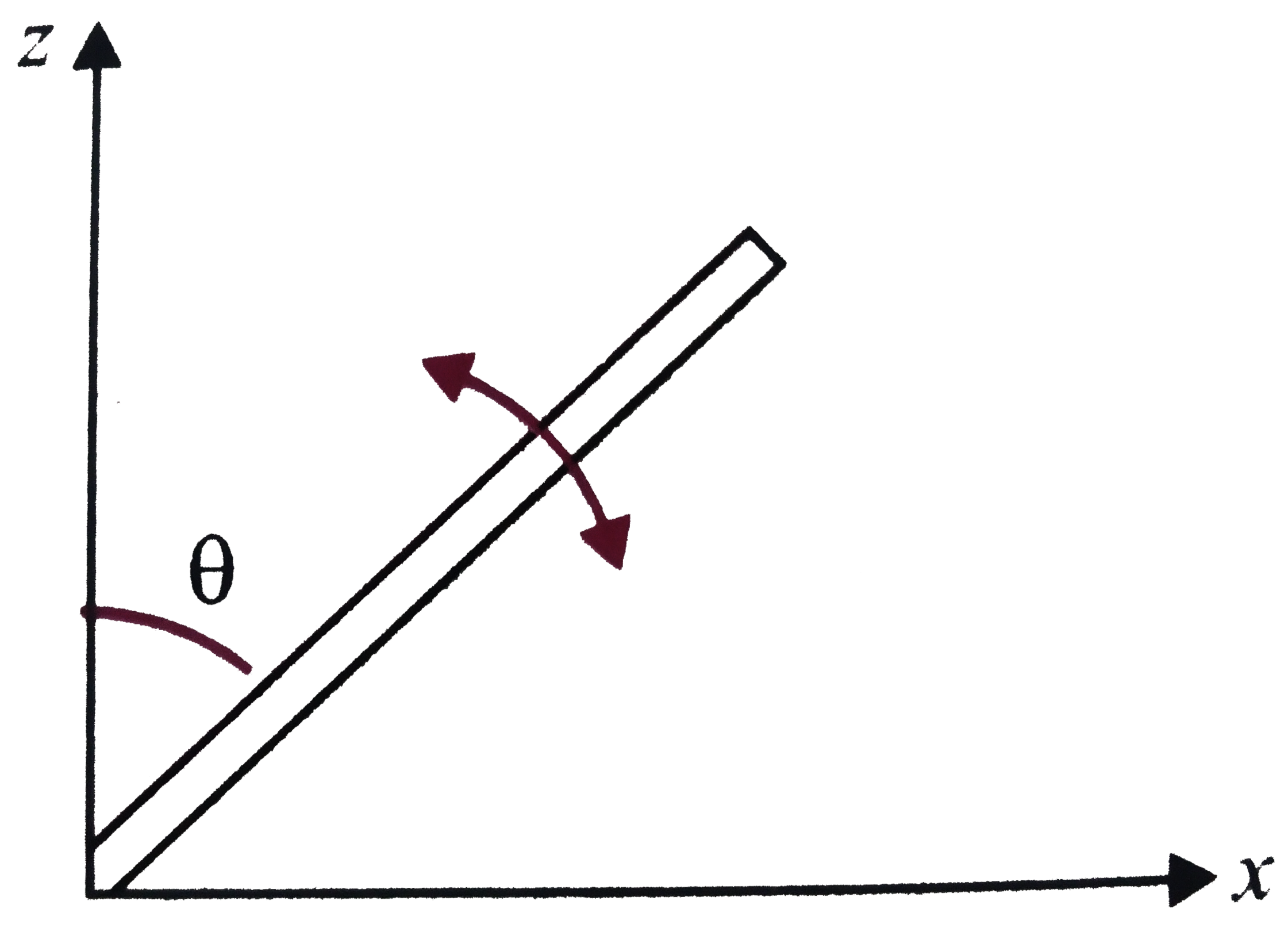 A3g2lcosθB2g3lcosθC3g2lsinθD2g3lsinθ
A3g2lcosθB2g3lcosθC3g2lsinθD2g3lsinθ - Question 2 - Select One
A L shaped rod of mass M is free to rotate in a vertical plane about axis A A as shown in figure. Maximum angular acceleration of rod is
 A3g√10IB9g10lC9g5lD6g5√2I
A3g√10IB9g10lC9g5lD6g5√2I
A rod of length l is released from the vertical position on a smooth horizontal plane. What is the nature of motion of the free end of the rod ?
View SolutionA uniform rod of mass m and length L is free to rotate in the vertical plane about a horizontal axis passing through its end. The rod initially in horizontal position is released. The initial angular acceleration of the rod is:
 View Solution
View SolutionA rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle θ from the vertical.
 View Solution
View SolutionA rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle θ from the vertical.
 View Solution
View SolutionA slender uniform rod of mass M and length l is pivoted at one end so that it can rotate in a vertical plane, Fig. There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is
 View Solution
View SolutionA chain with uniform mass per unit length lies in a vertical plane along the slope of a smooth hill. The two end of the chain are at same height. If the chain is released from this position find its acceleration.
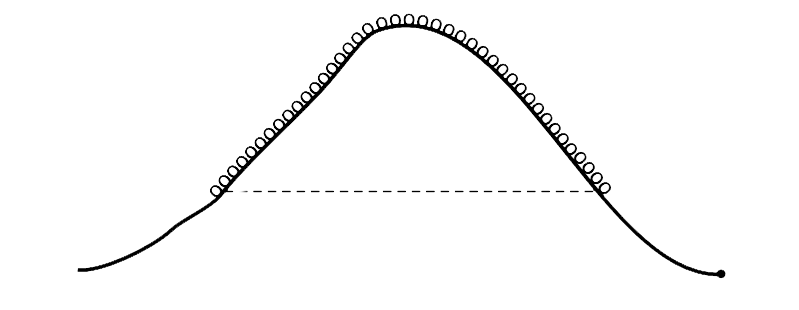 View Solution
View Solution

