A composite rod of mass 2m & length 2l consists of two identical rods joined end to end at P. The composite rd is hinged at one of its ends and is kept horizontal as shown in the figure. If it is released from rest,

(a) find its angular speed when it becomes vertical.
(b) If the lower rod gets detached with the upper rod due to centrifugal effect at their joint P, when the composite rod becomes vertical, find their linear and angular velocities just after their separation.
(a) Suppose that the composite rod acquires an angular speed ω when it reaches its vertical position. Its centre of mass G moves from G1toG2. Therefore the potential energy of the composite rod decreases by 2mgh where h = l.
Applying conservation of energy, 12Iω2=(2m)gl
where I = M.I. of the composite rod about O
=(2m)(2l)2/3=8ml2/3
⇒ω=√32gl

(b) Referring to fig. we can see that, just at the vertical position, during the break, the weights of the component rods 1 & 2, the reaction force R at the pivot & the reaction forces N at the joint of the rods, pass through the pivot O. Therefore these forces can not produce any moment about O, that means the rods do not experience any horizontal force and torque during breaking. At vertical position, the angular momentum of the system about O remains same as just after the breaking.
We can also argue that, the angular momentum of each rod remains constant just before & after breaking. The radial forces cannot produce any moment about the centre of mass of the rods 1 & 2 in the vertical position. The linear velocity of the c.m. of the rods remains constant.
⇒ ω1=ω
v.1=v1=ωl/2 {for rod 1}
ω2=ω,v.2=v2=(3/2)ωl {for rod 2}
⇒ ω1=ω2=√3g2l,v1=12√3gl2glandv2=32√3gl2

Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)
14 Videos
- ROTATIONAL MECHANICSBOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSERTION REASONING TYPE
1 Video
- ROTATIONAL MECHANICSBOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - COMPREHENSION - IV
2 Videos
- PHYSICS PART2BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type
6 Videos
- SEMICONDUCTOR AND DEVICEBOOK - FIITJEECHAPTER - SEMICONDUCTOR AND DEVICEEXERCISE - SOLVED PROBLEMS Objective: Level-I
20 Videos
Similar Questions
A composite rod of mass 2m and length 2l comprises two indentica rods joined end to end at P. The composite rod hinged at one of its ends is kept horizontal as shown in the figure. If it is realeased from rest.
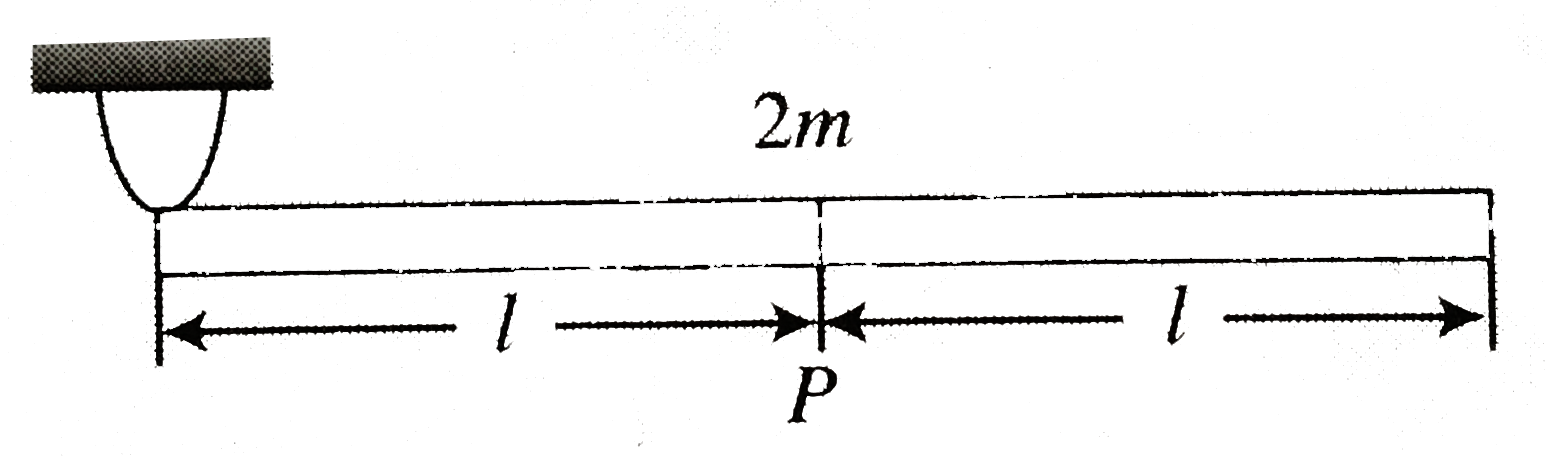
a. find its angular speed when it becomes vertical.
b. If the lower rod gets detached with the upper rod due to centrifugal effect at their joint P, at the vertical position of the composite rod, find their linear and angular velocities just after their separation.
View SolutionA composite rod of mass 2m and length 2l comprises two indentica rods joined end to end at P. The composite rod hinged at one of its ends is kept horizontal as shown in the figure. If it is realeased from rest.
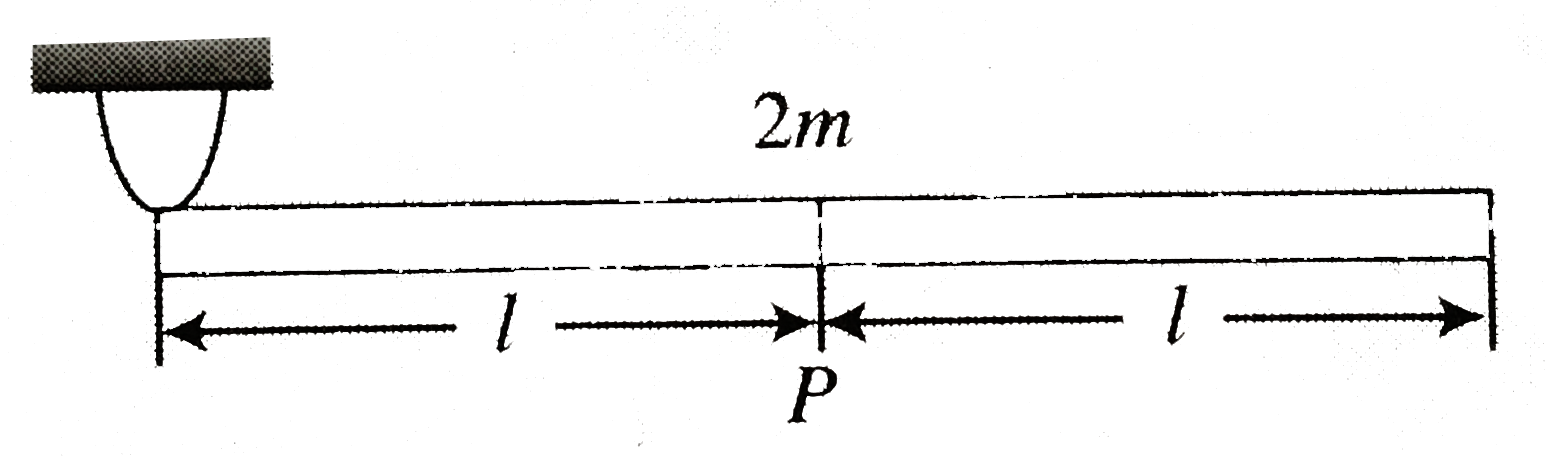
a. find its angular speed when it becomes vertical.
b. If the lower rod gets detached with the upper rod due to centrifugal effect at their joint P, at the vertical position of the composite rod, find their linear and angular velocities just after their separation.
View Solution
Knowledge Check
One end of a uniform rod of length l and mass m is hinged at A. It is released from rest from horizontal position AB as shown in figure. The force exerted by the rod on the hinge when it becomes vertical is
 A32mgB52mgC3mgD5mg
A32mgB52mgC3mgD5mgOne end of a uniform rod of length l and mass m is hinged at A. It is released from the rest from horizontal position AB as shown in figure. The force exerted by the rod on the hinge when it becomes verticle is
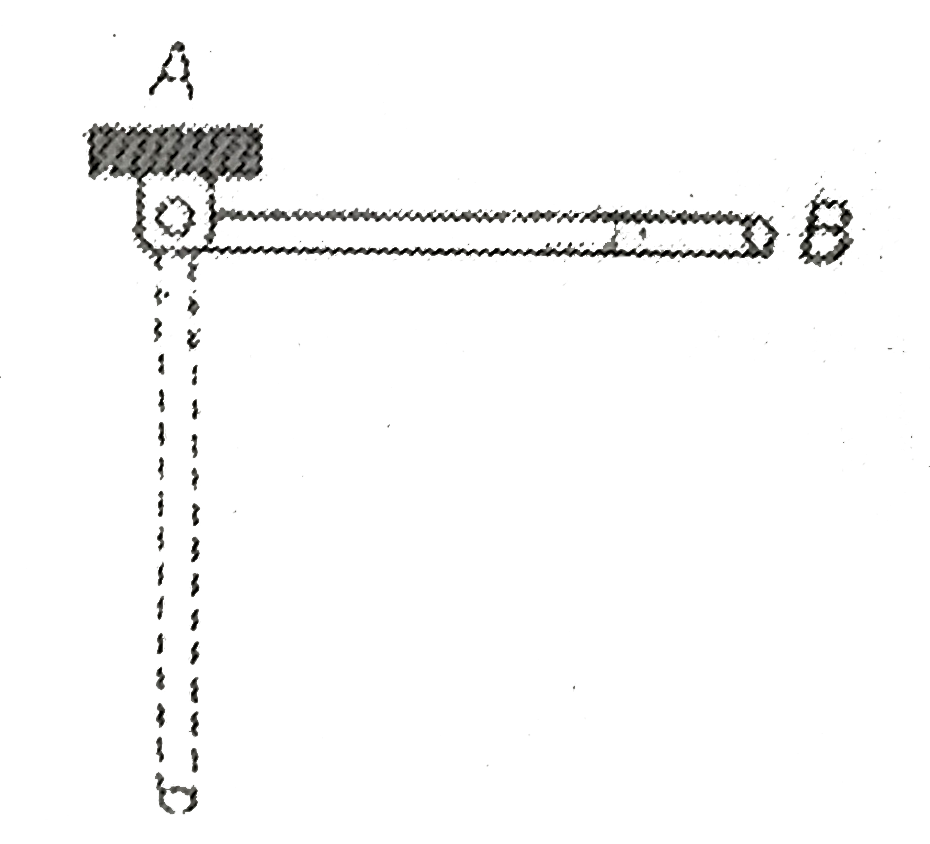 A32mgB52mgC3mgD5mg
A32mgB52mgC3mgD5mgA rod PQ of mass M and length L is hinged at end P. The rod is kept horizontal by a massless string tied to point Q as shown in the figure. When string is cut, the initial angular accleration of the rod is.
 A3g2LBgLC2gLD2g3L
A3g2LBgLC2gLD2g3L
One end of a uniform rod of length l and mass m is hinged at A. It is released from the rest from horizontal position AB as shown in figure. The force exerted by the rod on the hinge when it becomes verticle is

View SolutionA rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
View SolutionA rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
View SolutionA rod PQ of mass M and length L is hinged at end P. The rod is kept horizontal by a massless string tied to point Q as shown in the figure. When string is cut, the initial angular accleration of the rod is.

View SolutionA rod of mass m and length l is himged about one of its ends. The rod is released from horizontal position. When the rod becomes vertical, calculate (i) angular speed of the rod (ii) Hinge reaction.
View Solution
