In figure, if PQ||RS, prove that ΔPOQΔSOR.
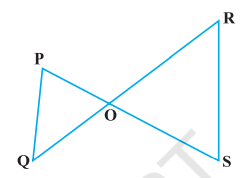
Given: PQ∣∣RS
To Prove: △POQ~△SOR
Let us take △POQ and △SOR
∠OPQ=∠OSR (as PQ∣∣RS, Alternate angles)
∠POQ=∠ROS (vertically opposite angles)
∠OQP=∠ORS (as PQ∣∣RS, Alternate angles)
△POQ~△SOR (by AAA similarity criterion) Hence Prove
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure PQ||LM||RS. Find the vlaue of ∠LRS:
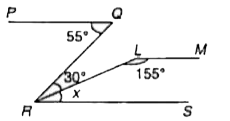 A30∘B25∘C35∘D40∘
A30∘B25∘C35∘D40∘
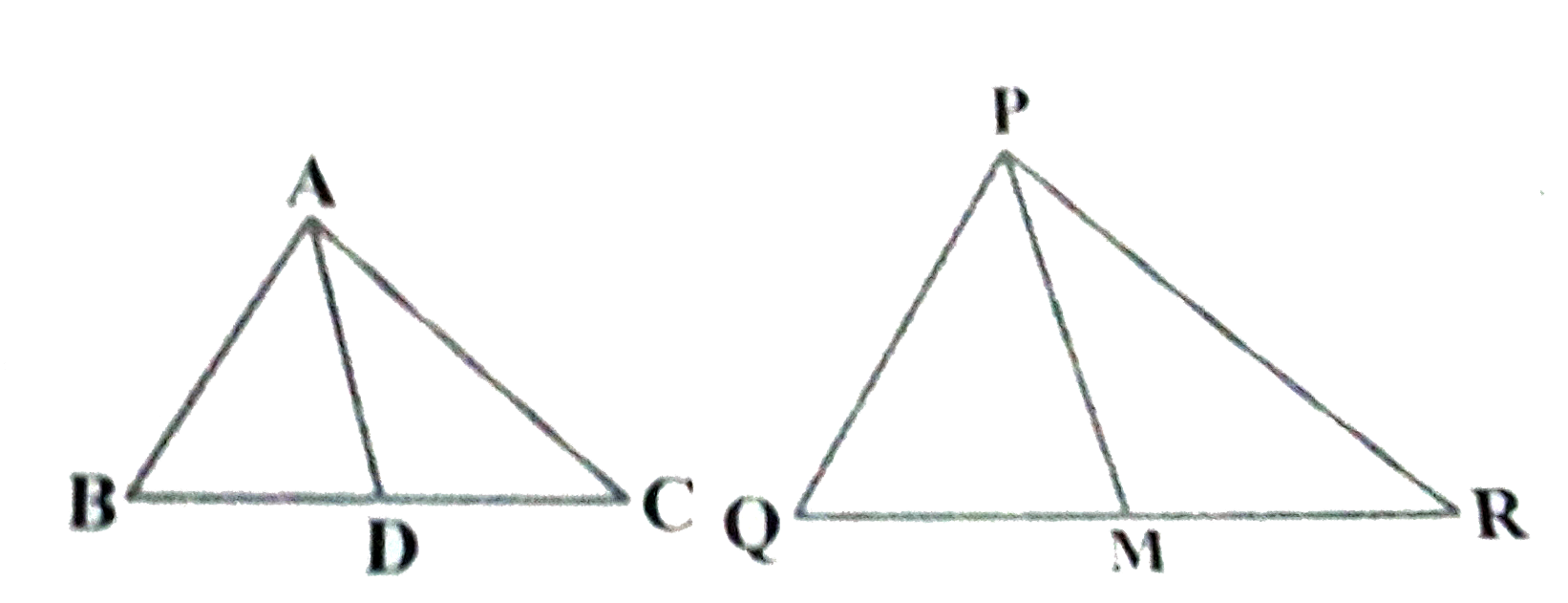
Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of ΔPQR. Show that ΔABC~ΔPQR.
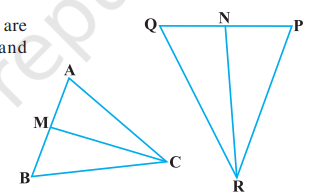
View SolutionIn figure Cm and RN are respectively the medians of ΔABCand ΔPQR. If ΔABC~ΔPQR, prove that:
(i) ΔAMC~ΔPNR
(ii) CMRN=ABPQ
(ii) ΔCMB~ΔRNQ
View SolutionSides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Show that ΔABC~ΔPQR.
View SolutionIn Fig. 4.90, if POS~ROQ , prove that PSQR . (FIGURE)
View SolutionIn figure PS is the bisector of ∠QPRof ΔPQR. Prove that QSSR=PQPR.

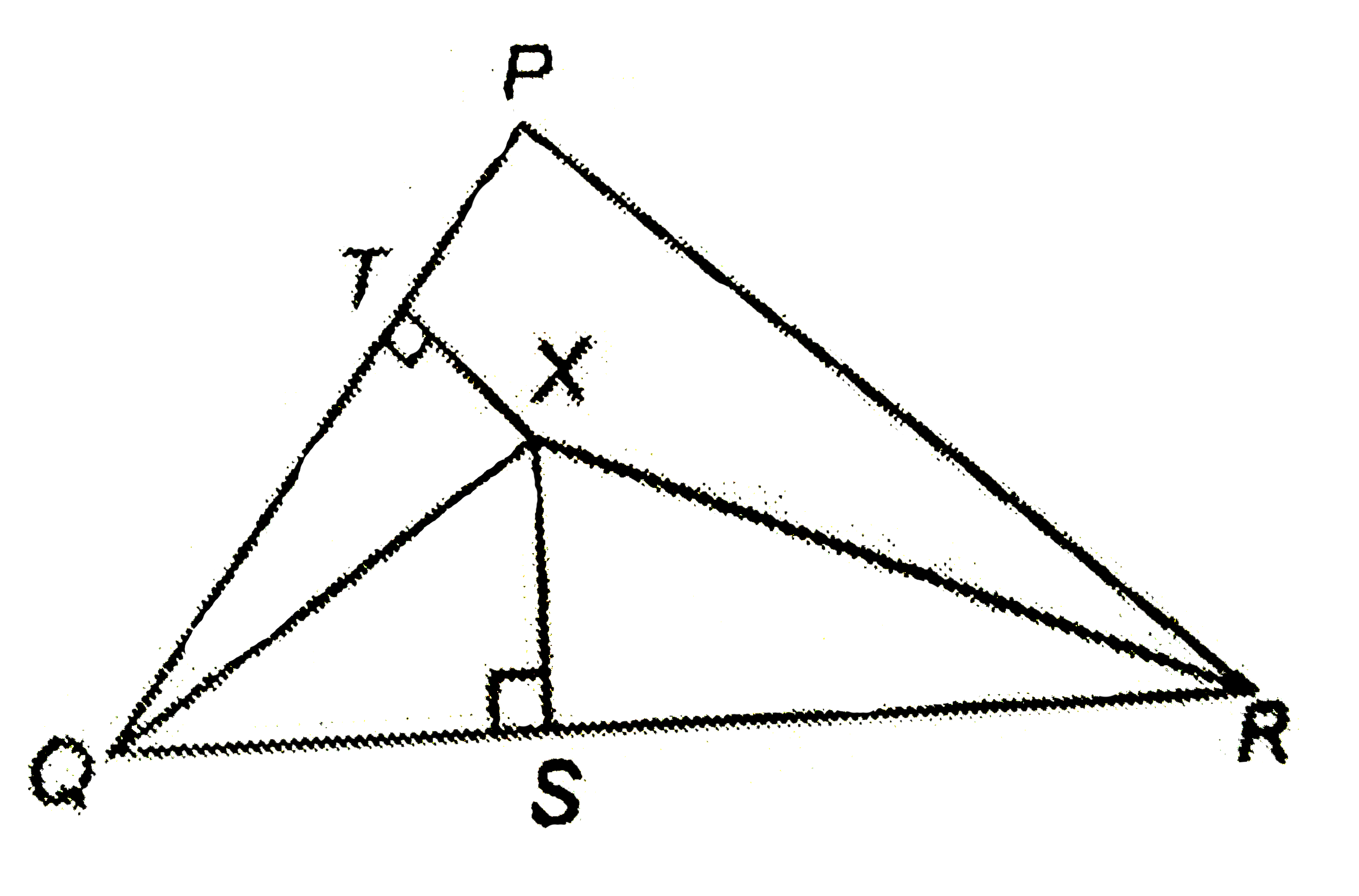
View SolutionIn the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the angles Q and R respectively of the triangle PQR. If XS⊥PQ. Prove that :
(i) ΔXTQ≅ΔXSQ
(ii) PX bisects angle P.
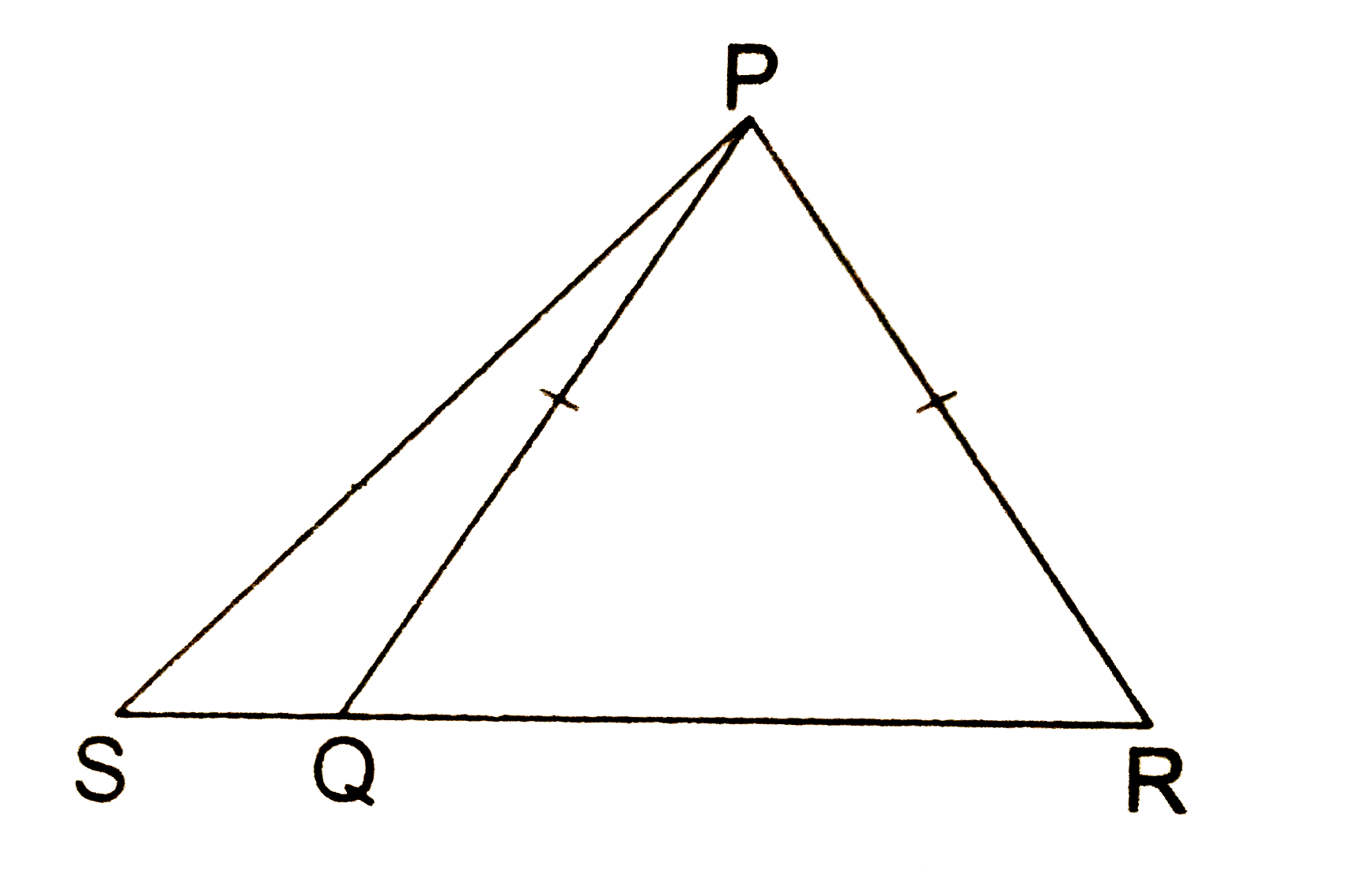
View SolutionIn the given figure, Q is a point on the side SR of ΔPSR such that PQ = PR. Prove that PS>PQ.
View Solution
