(a) State Lens law. Give one example to illustrate this law. '' The Lenz's law is a consequence of the principle of conversation of energy .' Justify this statement.
(b) Deduce an expression for the mutual inductance of two long coaxial solenoids but having different radil and different radil and different number of turns.
(a)Lenz's law:It states that the direction of induced current or in a circuit is always such that it opposes the cause which produces it.
It gives the direction of current or induced in a circuit.
Lenz's law is in accordance with the principles of conservation of energy.In electromagnetic induction, the electrical energy (in the form of induced current or induced ) is obtained at the expense of mechanical energy.
(b)Coefficient of mutual induction:The coefficient of mutual induction or mutual inductance of two coils is numerically equal to the amount of magnetic flux linked with one coil when unit current flows through the neighbouring coil.
It is denoted by .Its unit is weber/ampere.
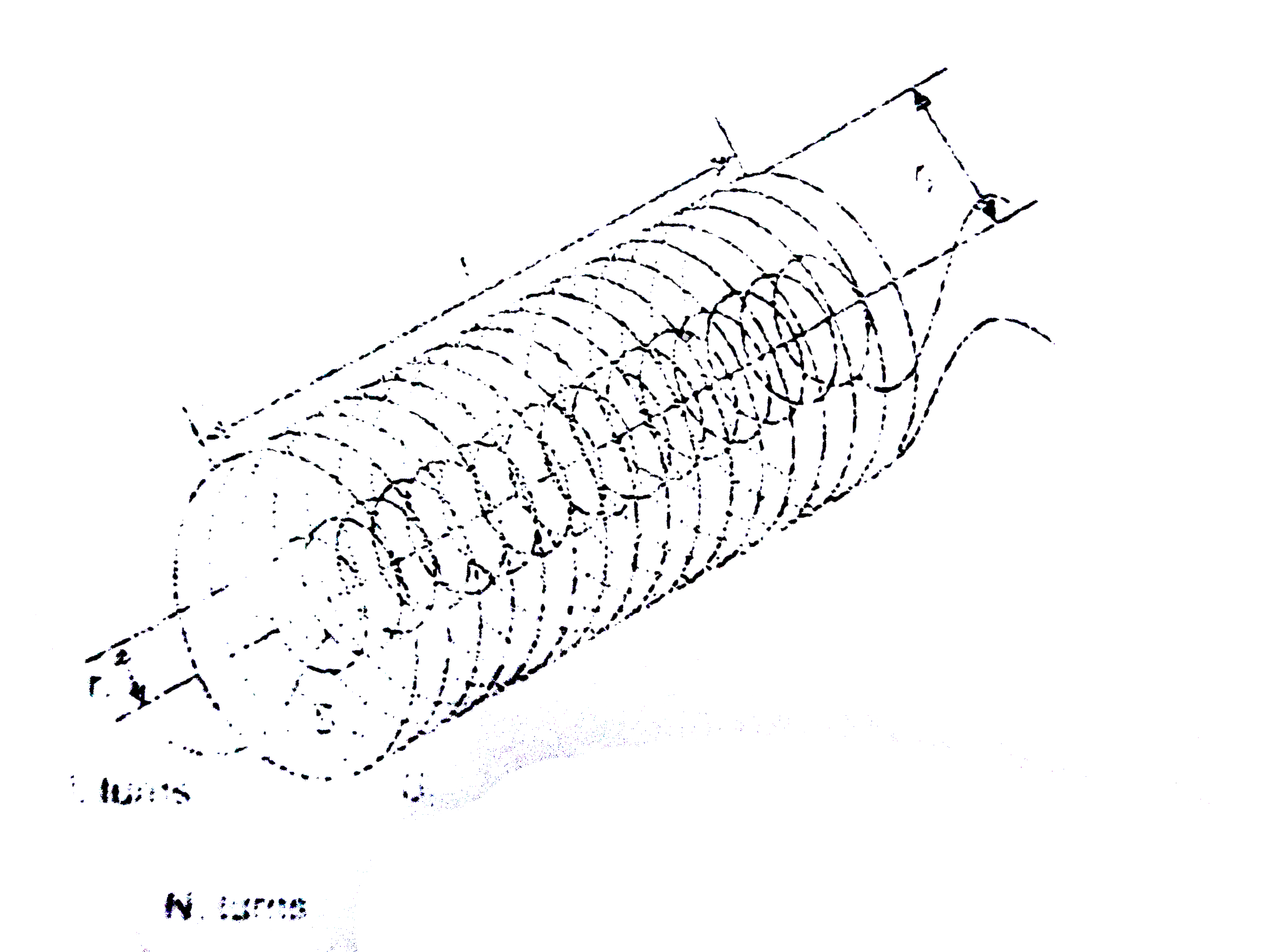
Mutual inductance of two long coaxial solenoids:Consider the following fig.Which shows two long coaxial solenoids each of length let the radius of the inner solenoid be and the number of turns per unit length be
The corresponding quantities for the outer solenoid are and respectively. Let and , be the total number of turns of coils and respectively.
..(1)
where is called the mutual inductance of solenoid with respect to solenoid .The magnetic field due to the current in is .The resulting flux linkage with coil is ..(2)
where is the total number of turns in solenoid From eq.(1) and (2) we get
or ...(3)
Now consider the reverse case a current is passed through the solenoid and the flux linkage with coil is
..(4)
Where ,is called the mutual inductance of solenoid with respect to solenoid .The flux due to the current in can be assumed to be confined only inside , Since the solenoids are very long.Thus, flux linkage with solenoid is
...(5)
Where is the total number of turns of .
From eq.(4) and (5), we get
...(6)
From eq (3) and (4), we get
(say)
Therefore
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-3 PART 2
16 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
(a) State Lanz's law. Give one example to illustrate this law. "The Lenz's law is a consequence of the principle of conservation of energy." Justify this statement.
(b) Deduce a expression for the mutual inductance of two long coaxial solenoids but having different radii and different number of turns.
View SolutionState and explain Lenz's law. Give one exmple to illustrate the law. How will you verify it experimentally ? Does it obey the principle of energy consevartion ?
View SolutionState and explain Lenz's law of electromagnetic induction. Give one example to illustrate the law. How can it be verified experimentally?
View SolutionDeduce an expression for the mutual inductance of two long coaxial solenoids but having different radii and different number of turns.
Or
Obtain the expression for the mutual inductance of two long coaxial solenoids S1 and S2 wound one over the other, each of length I and radii r1andr2, and number of turns per unit length n1andn2 respeetively, when a current I is set up in the outer solenoid S2.
View Solution(a) Explain the meaning of the term mutual inductance. Consider two concentric circular coils, one radius r1 and the other of radius r2(r1<r2) placed coaxially with centres coinciding with each other. Obtain the expression for the mutual inductance of the arrangement.
(b) A rectangular coil of area A, having number of turns N is rotated at 'f' revoluation per second in a uniform magnetic field B, the field being perpendicular to the coil. Prove that maximum emf induced in the coil is 2πfNBA.
View SolutionDefine mutual inductance between two long coaxial solenoids. Find out the expression for the mutual of inner solenoid of length 1 having the radius r1 and the number of turns n1 per unit length due to the second other solenoid of same length and n2 number of turns per unit length.
View SolutionDefine mutual inductance between two long coaxial solenoids. Find out the expression for the mutual of inner solenoid of length 1 having the radius r1 and the number of turns n1 per unit length due to the second other solenoid of same length and n2 number of turns per unit length.
View SolutionA long solenoid of length l=2.0 m , radius r=0.1 m and total number of turns N=1000 is carrying a current i0=20.0A. The axis of the solenoid conicides with the z-axis.
(a) State the expression for the magnetic field of the solenoid and calculate its value ? Magnetic field.
(b) Obtain the expression for the self - inductance (L) of the solenoid. Calcuate its value. Value of L .
( c) Calculate the energy stored (D) when the solenoid carries this current ?
(d) Let the resistance of the solenoid be R. It is connected to a battery of emf e. Obtain the expression for the current (i) in the solenoid.
(e) Let the solenoid with resistance R describe in part (d) be strecthed at a constant speed u ( l is increased but B and γ are constant ). State kirchhoff's second law for this cases. (Note: Do not solve for the current )
(f) Consider a time varying current i=i0cos(ωt) (where i0=20.0A) flowing in the solenoid. Obtain an expression for the electric field due to the current in the solenoid. (Note: Part(e) is not operative, i.e., the solenoid is not being stretched. )
(g) Consider t=π/2ωandω=200/πrad−s−1 in the previous part. Plot the magnitude of the electric field as a function of the radial distance from the solenoid. Also, sketch the electric lines of force.
View SolutionOne of the fundamental laws of physics is that matter is most stable with the lowest possible energy. Thus, the electron in a hydrogen atom usually moves in the n=1 orbit, the orbit in which it has the lowest energy. When the electon is in this lowest energy orbit, the atom is said to be in its ground electronic state. If the atom receives energy from an outside source, it is possible for the electron to move ot an orbit with a higher n value, in which case the atoms is in an excited state with a higher energy.
The law of conservation of energy says that we cannot create or destroy energy. Thus, if a certain amount of external energy is required to excite an electron from one energy level to another, then that same amount of energy will be liberated when the electron returns to its initial state.
Lyman series is observed when the electron returns to the lowest orbit while Balmer series is formed when the electron returns returns to second orbit. Similarly, Paschen, Brackett and Pfund series are formed when electrons returns to the third, fourth and fifth orbits from higher energy orbits respectively.
When electrons return form n2 to n1 state, the number of lines in the spectrum will equal to
(n2−n1)(n2−n1+1)2
If the electon comes back from energy level having energy E2 to energy level having energy E1, then the difference may be expressed in terms of energy of photon as :
E2−E1=ΔE,δE⇒hcλ
Since, h and c are constant, δE corresponds to definite energy. Thus, each transition from one energy level to another will produce a radiatiob of definite wavelength. This is actually Wave number of a spectral line is given by the formula
¯v=R(1n21−1n22).
where R is a Rydberg's constant (R=1.1×107m−1)
The emission spectra is observed by the consequence of transition of electrons from higher energy state to ground state of He+ ion. Six different photons are observed during the emission spectra, then what will be the minimum wavelength during the transition?
View SolutionOne of the fundamental laws of physics is that matter is most stable with the lowest possible energy. Thus, the electron in a hydrogen atom usually moves in the n=1 orbit, the orbit in which it has the lowest energy. When the electon is in this lowest energy orbit, the atom is said to be in its ground electronic state. If the atom receives energy from an outside source, it is possible for the electron to move ot an orbit with a higher n value, in which case the atoms is in an excited state with a higher energy.
The law of conservation of energy says that we cannot create or destroy energy. Thus, if a certain amount of external energy is required to excite an electron from one energy level to another, then that same amount of energy will be liberated when the electron returns to its initial state.
Lyman series is observed when the electron returns to the lowest orbit while Balmer series is formed when the electron returns returns to second orbit. Similarly, Paschen, Brackett and Pfund series are formed when electrons returns to the third, fourth and fifth orbits from higher energy orbits respectively.
When electrons return form n2 to n1 state, the number of lines in the spectrum will equal to
(n2−n1)(n2−n1+1)2
If the electon comes back from energy level having energy E2 to energy level having energy E1, then the difference may be expressed in terms of energy of photon as :
E2−E1=ΔE,δE⇒hcλ
Since, h and c are constant, δE corresponds to definite energy. Thus, each transition from one energy level to another will produce a radiatiob of definite wavelength. This is actually Wave number of a spectral line is given by the formula
¯v=R(1n21−1n22).
where R is a Rydberg's constant (R=1.1×107m−1)
An electron in H-atom in M-shell on de-excitation to ground state gives maximum ........... spectrum lines.
View Solution
