Find the number of roots of the equation $x sin x = 1, x \in [- 2 π, 0) \cup (0, 2 π]$ .

Video Solution

Text Solution

Verified by Experts

We have x sin x = 1
$∴$ $sin x = \frac{1}{x}$
Number of roots of the above equation = number of points (s) of the intersection of the graphs of $y = sin x$ and $y = \frac{1}{x}$ for $x \in [- 2 π, 0) \cup (0, 2 π]$
The graphs of both functions are as shown in the following figure.

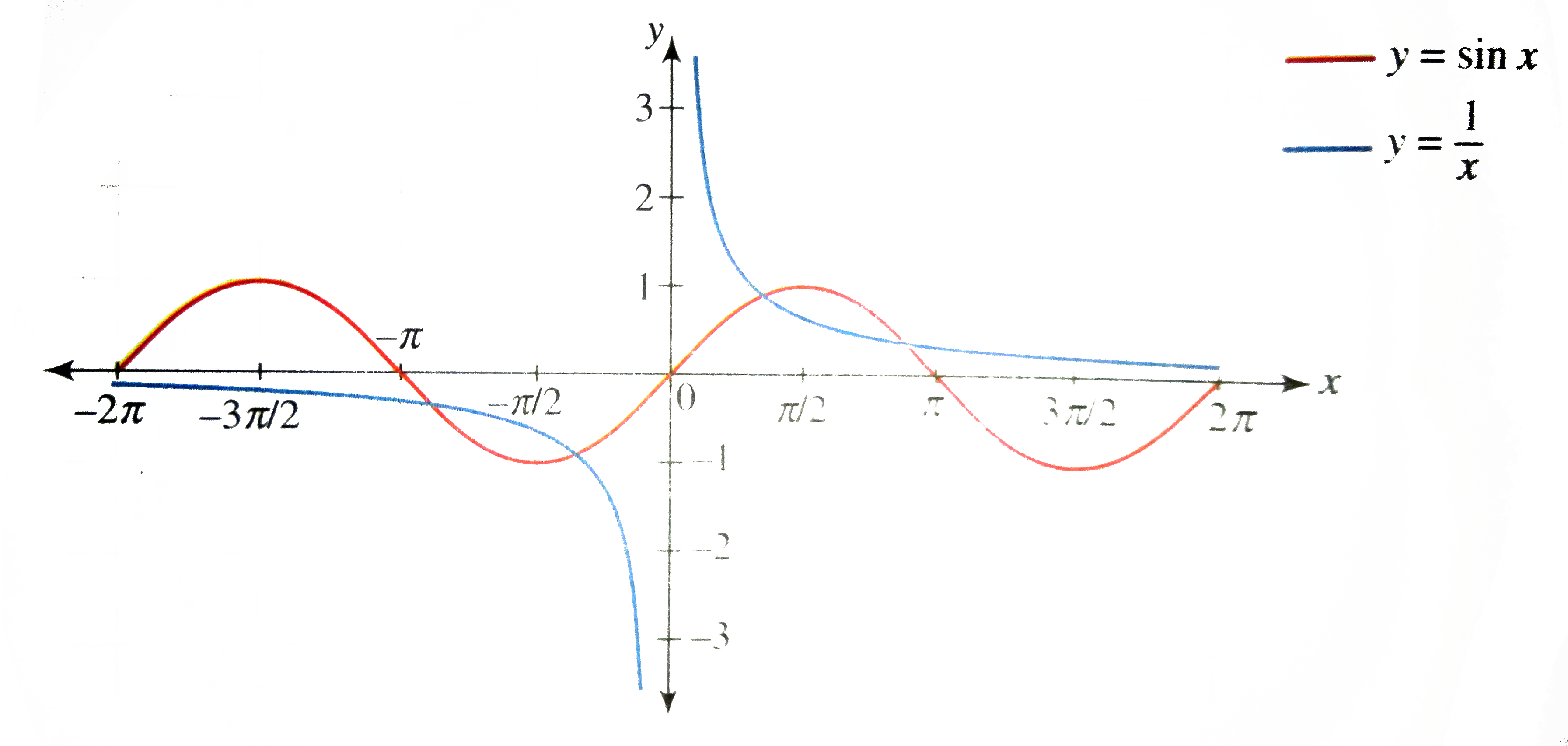
Graphs intersect at four points, hence four roots.

|

Updated on:21/07/2023

Class 12 MATHS GRAPHS OF TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The number of real roots of the equation
$x^{2} + x + 3 + 2 sin x = 0, x \in [- π, π]$ , is
A2
B3
C4
DNone of these
Question 2 - Select One
The number of solutionsof the equation
$1 + sin x {sin}^{2} \frac{x}{2} = 0$ in $[- π, π]$ is
Azero
B1
C2
D3
Question 3 - Select One
The number of solutions of the equation $sin x . Sin 2 x . Sin 3 x = 1$ in $[0, 2 π]$ is
A0
B1
C2
D3

CENGAGE-GRAPHS OF TRIGONOMETRIC FUNCTIONS-Exercise

Home
Ask Doubt
Profile