Draw the graph of
Video Solution
Text Solution
We have
Clearly, the domain of the function is
So the period of is .
We need to draw the graph of the function for .
for
and , when sin 2x = 1 or
Thus, the graph of the function for is as follows.
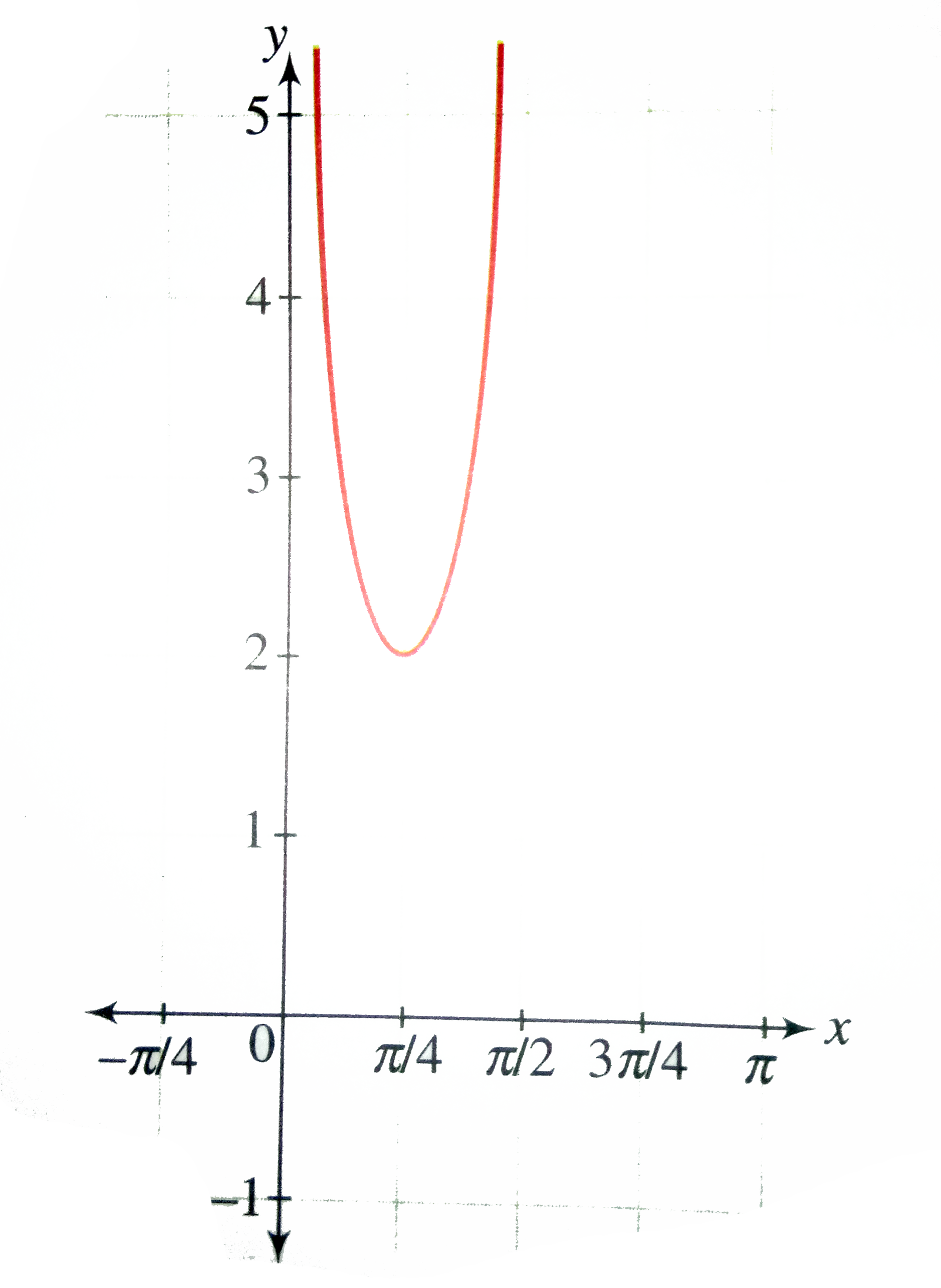
Since the period of is the graph is repeated as shown in the following figure.
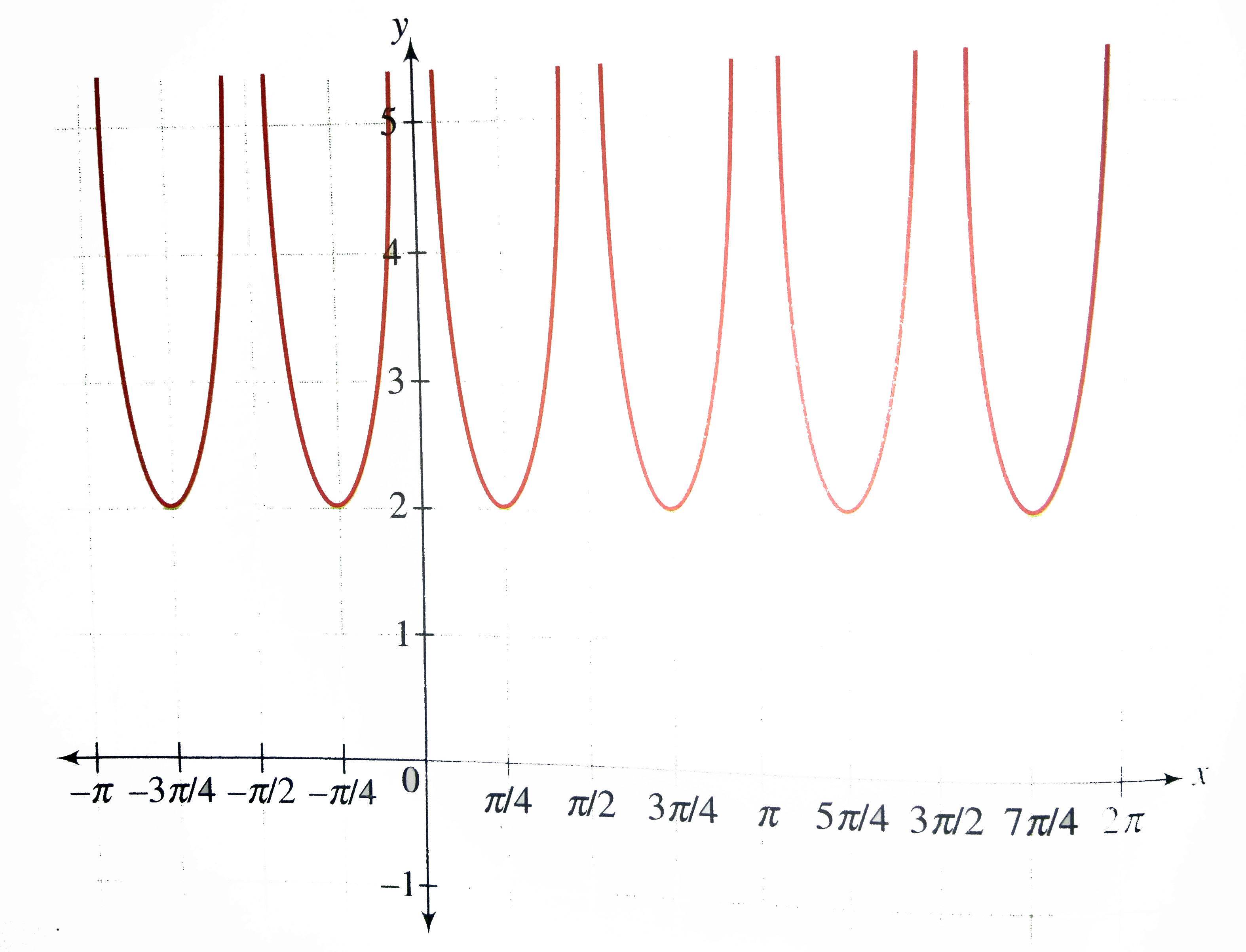
|
Topper's Solved these Questions
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- HIGHT AND DISTANCE BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year
3 Videos
Similar Questions
Draw the graph of f(x)=x|x|.
View SolutionDraw the graph of f(x)=sgn(x−2).
View SolutionDraw the graph of f(x)=|sinx|+|cosx|,x∈R.
View SolutionDraw the graph of f(x)=esinx.
View SolutionDraw the graph of f(x)=√sinx.
View SolutionDraw the graph of y=tan2x.
View SolutionDraw the graph of f(x)=(x+2)|x−1|.
View SolutionDraw the graph of f(x)=x2−3
View SolutionDraw the graph of y=tan(3x).
View SolutionDraw the graph of f(x)=[tanx],0≤x≤5π/12, where [⋅] represents the greatest integer function.
View Solution
