Evaluate : $[lim_{x \to 0} \frac{\tan x}{x}]$ , where $[\cdot]$ represents the greatest integer function.

Video Solution

Text Solution

Verified by Experts

Let us first draw the graph of y = x and y = tan x.
For $y = x, y' = 1$ , i.e. the slope of the line is 1 for all real x.
For $y = \tan x, y' = \sec^{2} x$
Thus, both y = x and y = tan x have slope '1' at x = 0, hence the graphs touch each other.
Moving from '0' to $' π / 2'$ the values of $\sec^{2} x$ increase from '1' to $\infty$ .
Thus, the graph pf y = tan x lies below the graph of y = x.
The graphs of y = x and y = tan x are plotted as follows.

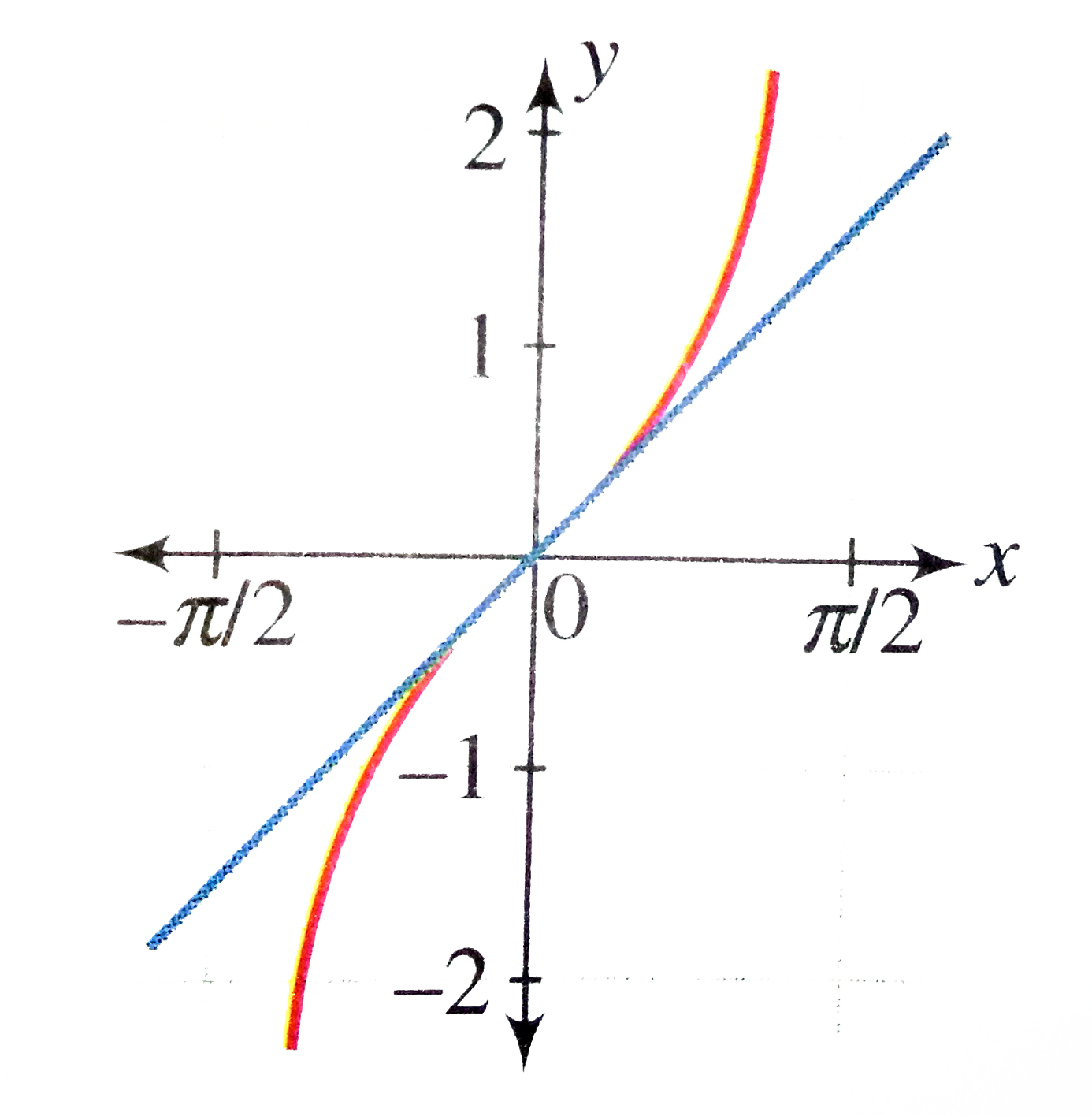
From the graph, when $x \to 0^{+}$ , the graph of y = tan x is above the graph of y = x
or $\tan x > x \Rightarrow \frac{\tan x}{x} > 1 \Rightarrow [lim_{x \to 0^{+}} \frac{\tan x}{x}] = 1$
When $x \to 0^{-}$ , the graph of y = x is above the graph of y = tan x
or $\tan x < x \Rightarrow \frac{\tan x}{x} > 1$ (as x is negative) $\Rightarrow [lim_{x \to 0^{+}} \frac{\tan x}{x}] = 1$
Thus, $[lim_{x \to 0} \frac{\tan x}{x}] = 1$

Updated on:21/07/2023

|

Class 12 MATHS GRAPHS OF TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

The value of $lim x \to 0 [\frac{x}{sin x}]$ , where $[.]$ represents the greatest integer function,is
A1
B0
C-1
Dnone of these
$lim x \to 0 [(1 - e^{x}) \frac{sin x}{| x |}]$ is (where $[.]$ represents the greatest integer function )
A1
B2
C3
Dnone of these

CENGAGE-GRAPHS OF TRIGONOMETRIC FUNCTIONS-Exercise

Home
Ask Doubt
Profile