Draw the graph of y=sin−1(logex). Also find the point of inflection.

Text Solution
Verified by Experts

We have y=f(x)=sin−1(logex)
Heref(x)isdef∈edif−1≤ logex≤1
or e−1≤x≤e
f(x)=sin−1(logex)=0
∴ logex=0
∴ x=1
So the graph meets the x-axis at (1,0).
f'(x)=1x√1−(logex)2>0, hence f(x) is invreasing.
f'(x)=√1−(logex)2−logex√(1−(logex)2)x2(1−(logex)2)
f'(x)=√1−(logex)2−logex√(1−(logex)2)=0
∴ (logex)2+logex−1=0
∴ logex=−1+√52
∴ x=e−1+√52
Hence the graph of the function is as shown in the following figure.
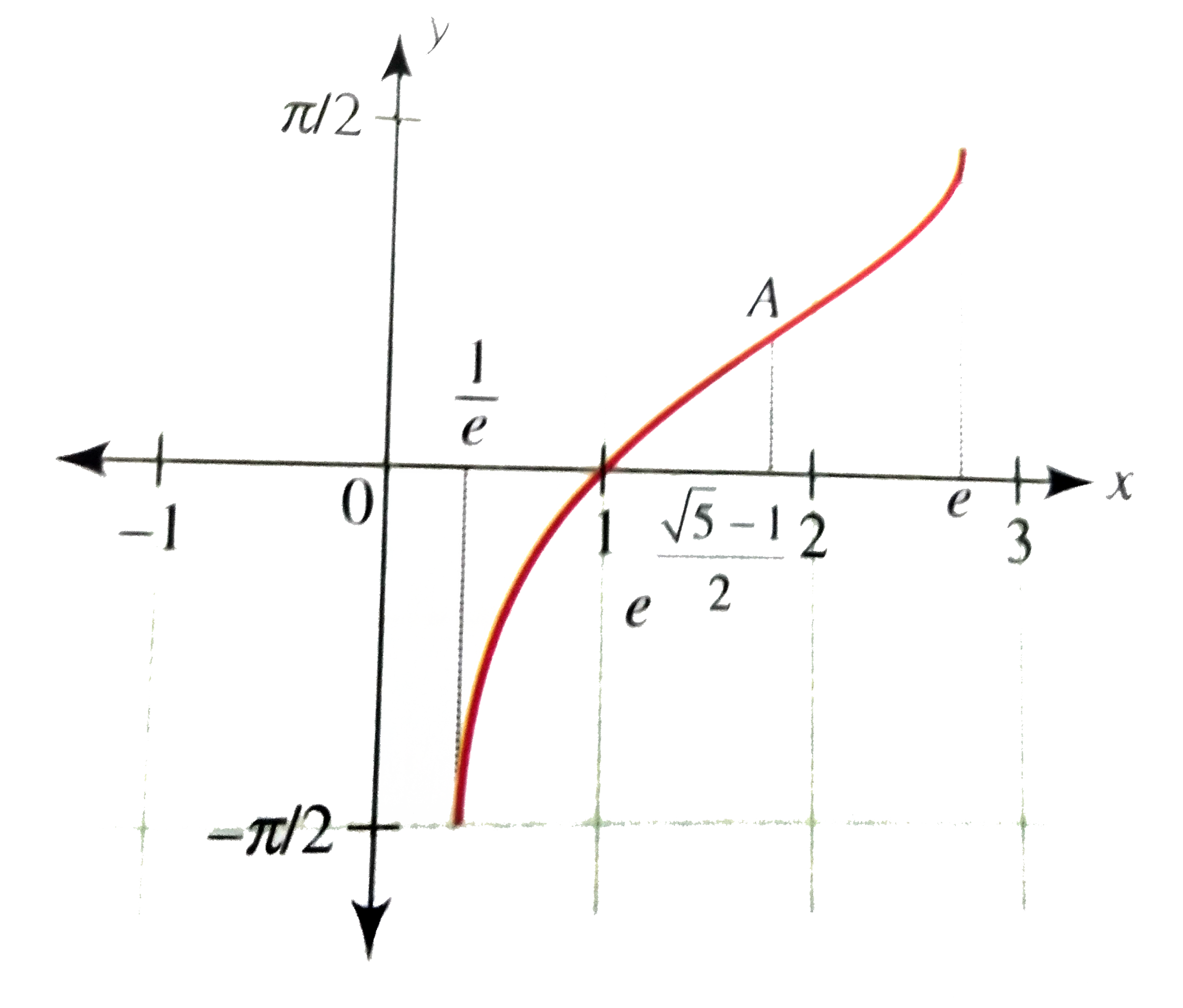
ocr_image
In the figure, the graph has his point of infection at point A.

Updated on:21/07/2023
Doubtnut Promotions Banner
|