Two particles A and B of masses 1 kg and 2 kg respectively are projected in the directions shown in figure with speed uA=200 m/s and uB=50 m/s . Initially they were 90 m apart . Find the maximum height attained by the centre of mass of the particles . Take g=10m/s2

Let h0 be the height of CM initally , Then
h0=(mBmA+mB)×d=(21+2)×90m=60m
Alternativley using mArA=mBrB
or (1) (rA)=(2)(rB)orrA=2rBandrA+rB=90m
Solving these two equations , we get rA=60mandrB=30cm
Hence , CM is at height h0=60m from the gorund at time t = 0 .
Further →aCM=mA→aA+mB→aBmA+mB
Because aA=aB=g
(downwards ) ⇒aCM=(mA+mBmA+mB).g=10m/s2
→uCM=mA→uA+mB→uBmA+mB=(1)(200)+(2)(−50)1+2=1003m/s (upwards )
Let h be the maximum height attained by Cm beyond 60 m . Using equations for constant acceleration ,
v2CM=u2CM+2aCMh
⇒0=(1003)2−(2)(10)h
⇒h=(100)218=55.55m
Therefore , maximum height attained by the centre of mass is
H=h0+h=60+55.55=115.55m
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 1
9 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 4
11 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI
78 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)
19 Videos
Similar Questions
Two particles A and B of mass 1 kg and 2 kg respectively are projected in the direction shown in figure with speeds uA=200ms−1 and uB=50ms−1. Initially they were 90 m apart. Find the maximum height attained by the center of mass of the particles. Assume acceleration due to gravity to be constant. (Take, g=10ms−2.
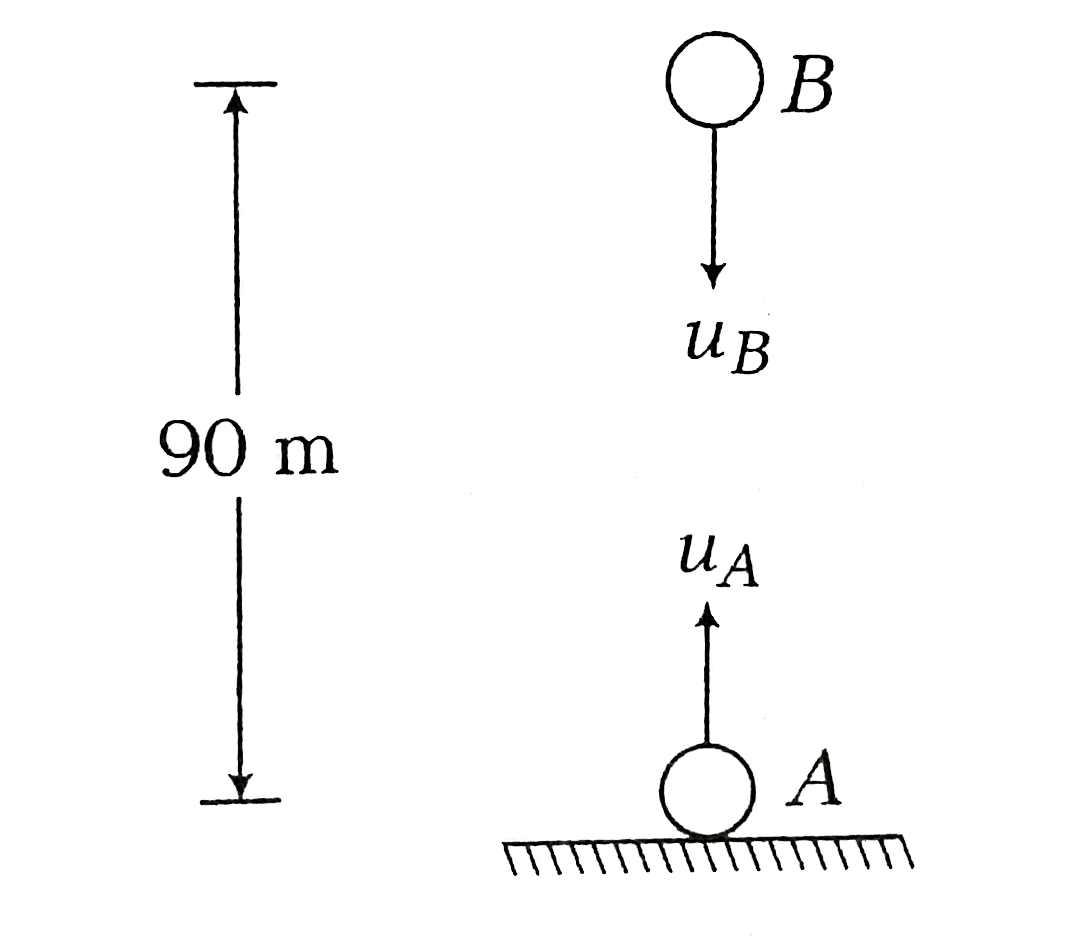
View SolutionTwo particles A and B of mass 1 kg and 2 kg respectively are projected in the direction shown in figure with speeds uA=200ms−1 and uB=50ms−1. Initially they were 90 m apart. Find the maximum height attained by the center of mass of the particles. Assume acceleration due to gravity to be constant. (Take, g=10ms−2.
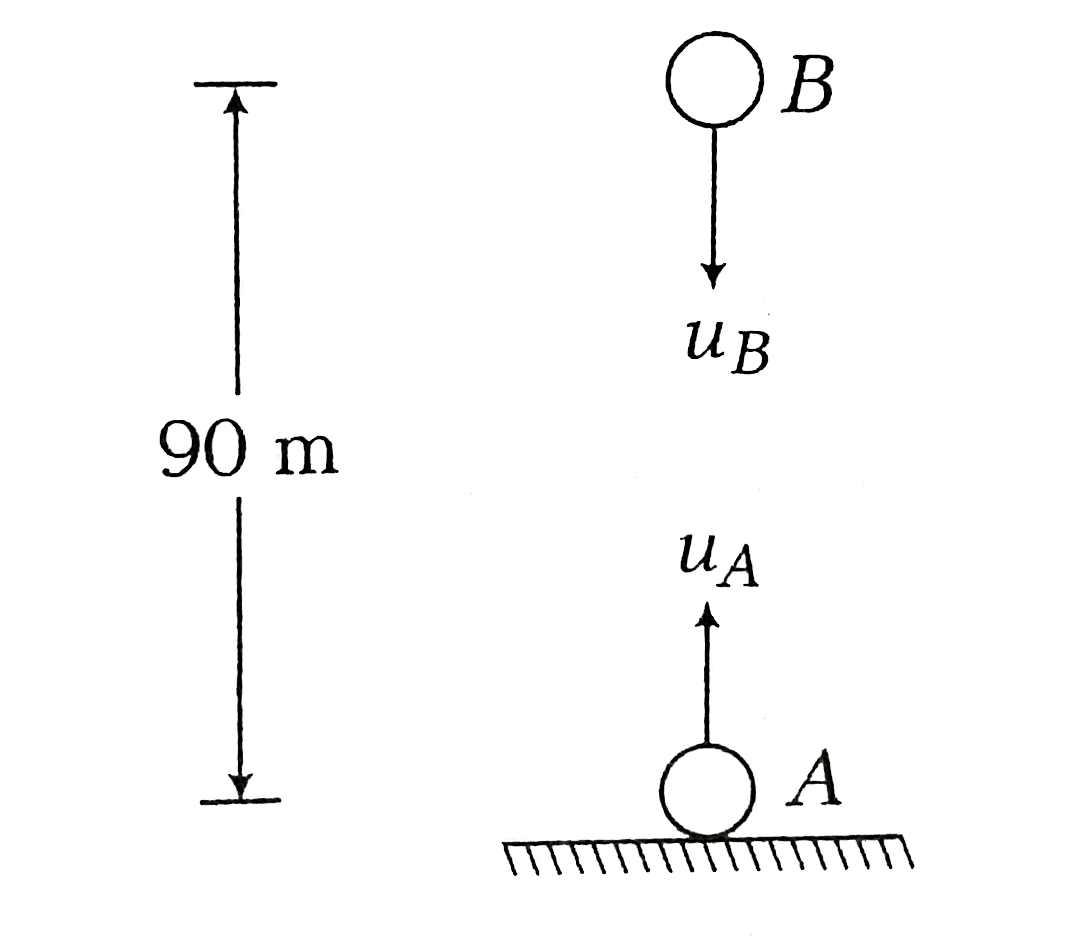
View Solution
Knowledge Check
Two particles A and B masses 1 kg and 2 kg respectivelty are projected in the same vertical line as shown in figure with speeds uA=200m/s and uB=85m/s respectively. Initially they were 90 m apart. Find the maximum height attained by the centre of mass of the system of particles A and B, from the initial position of centre of mass of the system. Assume that none of these particles collides with the ground in that duration Take g=10m/s2
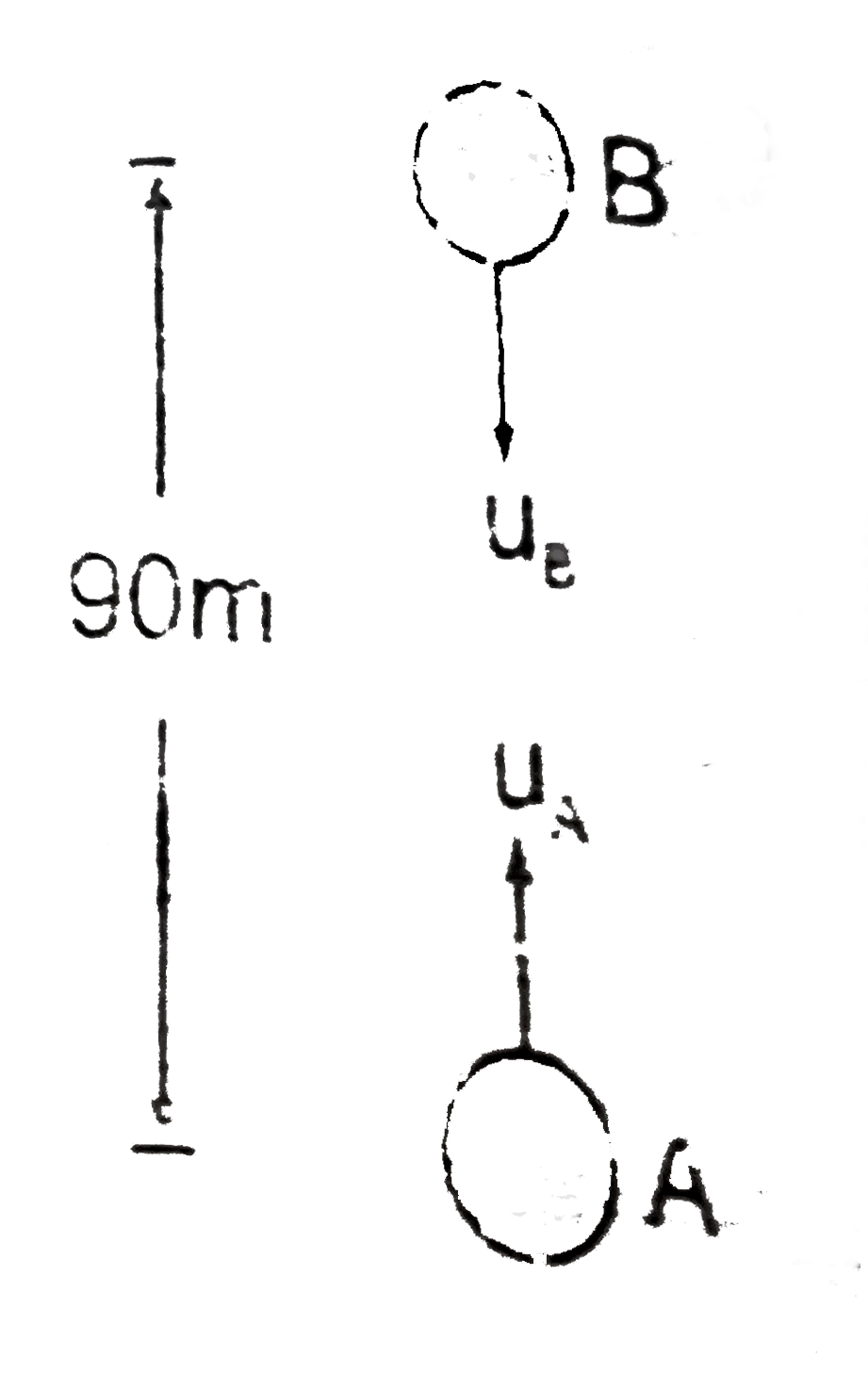 A5 meterB10 meterC25 meterD20 meter
A5 meterB10 meterC25 meterD20 meterTwo block A and B of mass 50kg and 300kg respectively are placed as shown in figure. Coefficient of friction between all surface is 12
Then (take g=10m/s)
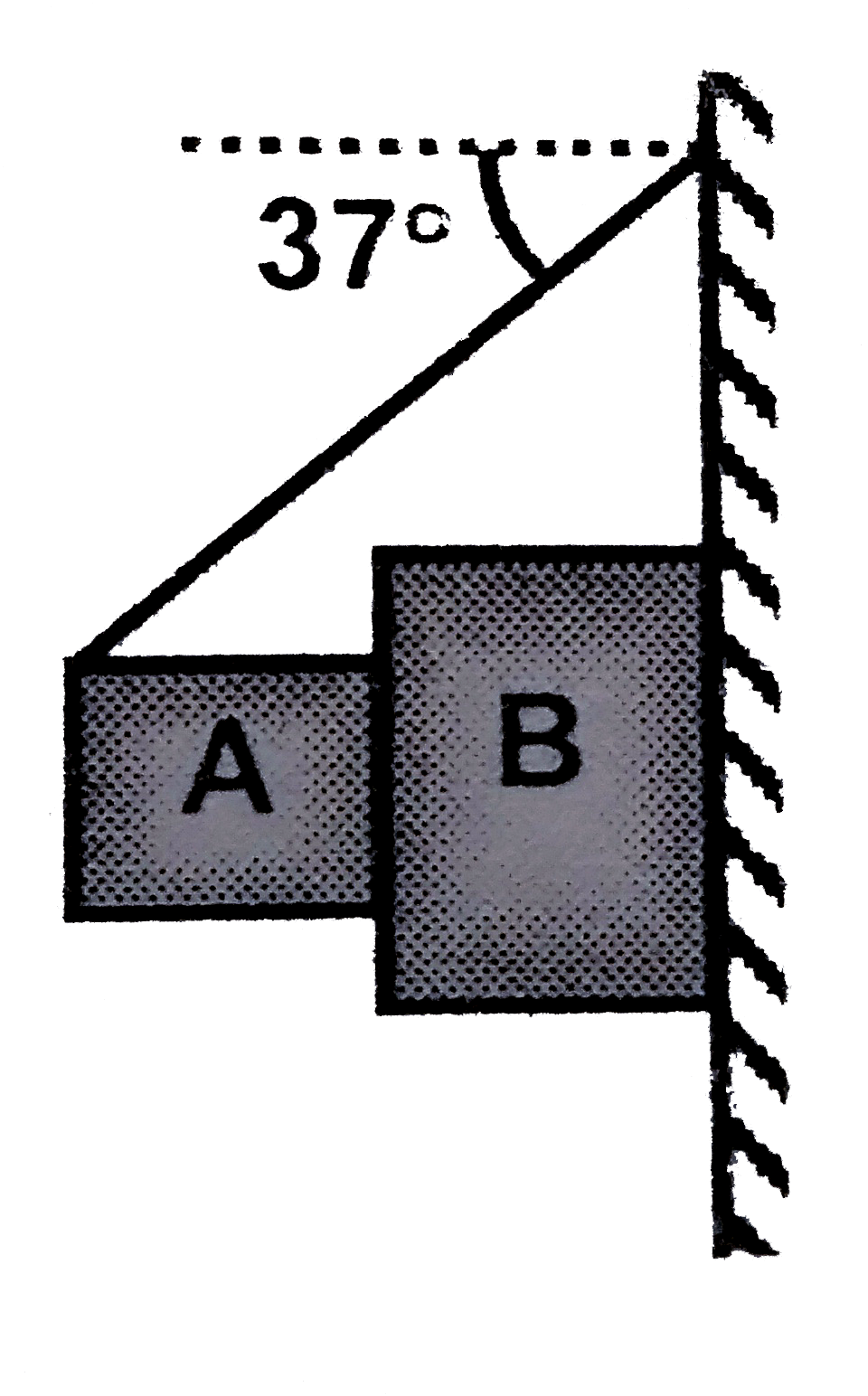 ATension in the string is 2000NBTension in the string is 3000NCAcceleration of block B is 5/3m/s2DAcceleration of block B is 10/3m/s2
ATension in the string is 2000NBTension in the string is 3000NCAcceleration of block B is 5/3m/s2DAcceleration of block B is 10/3m/s2
Two particles A and B of masses 1kg and 2kg respectively are projected in the directions shown in figure with speeds uA=200m/s and uB=50m/s. Initially they were 90m apart. They collide in mid air and stick with each other. Find the maximum height attained by the centre of mass of the particles. Assume acceleration due to gravity to be constant.
(g=10m/s2)

View SolutionTwo particles A and B of masses 1kg and 2kg respectively are projected in the directions shown in figure with speeds uA=200m/s and uB=50m/s. Initially they were 90m apart. They collide in mid air and stick with each other. Find the maximum height attained by the centre of mass of the particles. Assume acceleration due to gravity to be constant.
(g=10m/s2)

View SolutionTwo particles of equal mass are projected simultaneously with speeds 20m/s end 10√3m/s as shown in figure. Find the maximum height reached by the centre of mass of the particles
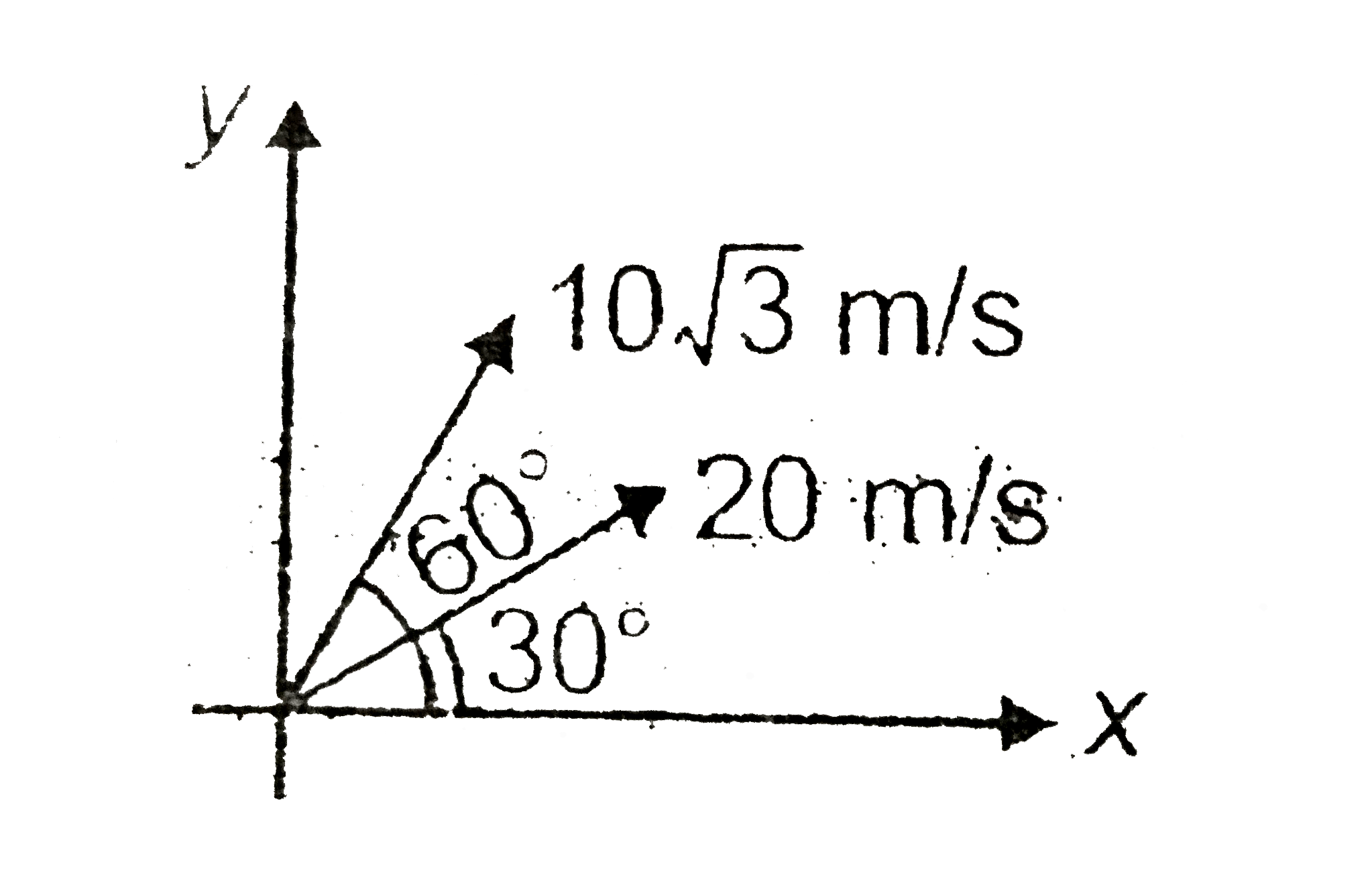
View SolutionTwo particles of equal mass are projected simultaneously with speeds 20m/s end 10√3m/s as shown in figure. Find the maximum height reached by the centre of mass of the particles
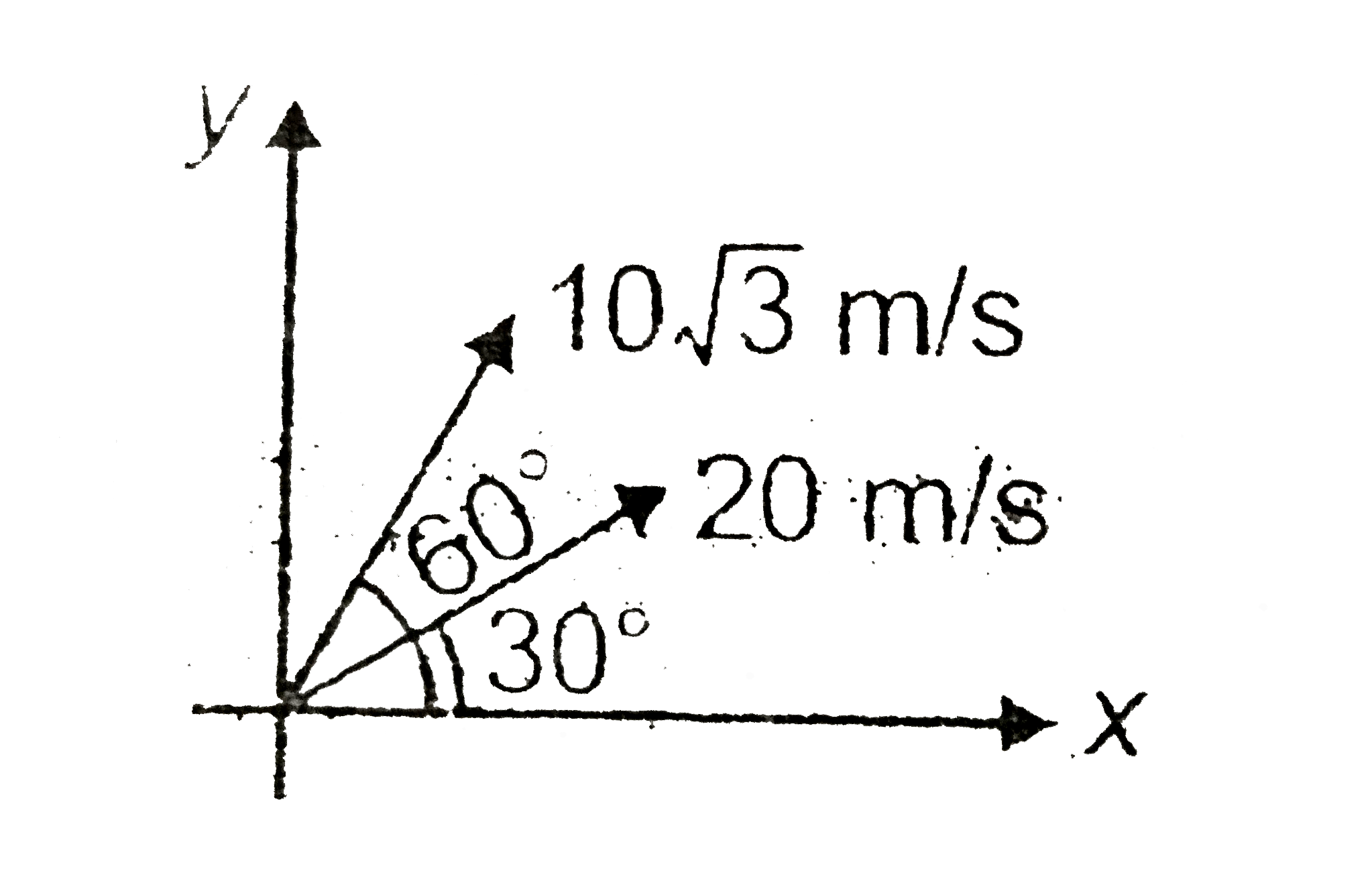
View SolutionTwo particles PandQ have mass 1kgand2kg respectively. They are projected along a vertical line with velocity up=20 m/s and uQ=5 m/s when separation between them was 60 m. P was projected vertically up while Q was projected vertically down. Calculate the maximum height attained by the centre of mass of the system of two particles, measured from the initial position of P. Assume that the particles do not collide and that the ground is far below their point of projection [g=10m/s2]
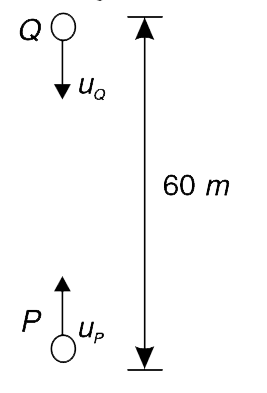
View SolutionTwo block A and B of mass 50kg and 300kg respectively are placed as shown in figure. Coefficient of friction between all surface is 12
Then (take g=10m/s)

View Solution
