A small block of mass 4 kg is attached to a cord passing through a hole in a horizontal frictionless syurface . The block is originally revolving in a circle of radius 0.5 m about the hole , with a tangential velocity of 4 m/s . The cord is then pulled slowly from below , shortening the radius of the circle in which the block revolves . The breaking strength of the cord is 600 N . What will be the radius of the circle when the cord breaks ?
To solve the problem, we need to find the radius of the circle when the cord breaks. We start with the information given:
- Mass of the block (m) = 4 kg
- Initial radius (R1) = 0.5 m
- Initial tangential velocity (V1) = 4 m/s
- Breaking strength of the cord (T_max) = 600 N
Step 1: Understand the relationship between angular momentum and radius
Since the block is revolving in a circle and the cord is being pulled, we can use the principle of conservation of angular momentum. Angular momentum (L) is given by:
L=m⋅v⋅r
Where:
- m is the mass of the block
- v is the tangential velocity
- r is the radius of the circle
Step 2: Set up the equation for angular momentum
Initially, the angular momentum (L1) is:
L1=m⋅V1⋅R1
When the radius changes to R2 and the velocity to V2, the angular momentum (L2) is:
L2=m⋅V2⋅R2
Since angular momentum is conserved, we have:
L1=L2
Thus,
m⋅V1⋅R1=m⋅V2⋅R2
We can cancel the mass m from both sides:
V1⋅R1=V2⋅R2
Step 3: Substitute known values
Substituting the known values:
4m/s⋅0.5m=V2⋅R2
This simplifies to:
2=V2⋅R2
Step 4: Express V2 in terms of R2
From the equation above, we can express V2:
V2=2R2
Step 5: Find the tension in the cord
The tension in the cord when the radius is R2 can be expressed using the centripetal force formula:
T=m⋅V22R2
Substituting V2:
T=m⋅(2R2)2R2
This simplifies to:
T=m⋅4R32
Step 6: Set the tension equal to the breaking strength
The maximum tension before the cord breaks is given as 600 N, so we set up the equation:
4⋅4R32=600
This simplifies to:
16R32=600
Step 7: Solve for R2
Now, we can solve for R32:
R32=16600
Calculating this gives:
R32=16600=275
Taking the cube root:
R2=(275)1/3
Step 8: Calculate the numerical value
Calculating R2:
R2≈0.29m
Final Answer
Thus, the radius of the circle when the cord breaks is approximately:
R2≈0.29m
---
- Initial radius (R1) = 0.5 m
- Initial tangential velocity (V1) = 4 m/s
- Breaking strength of the cord (T_max) = 600 N
Since the block is revolving in a circle and the cord is being pulled, we can use the principle of conservation of angular momentum. Angular momentum (L) is given by:
- m is the mass of the block
- v is the tangential velocity
- r is the radius of the circle
Initially, the angular momentum (L1) is:
Substituting the known values:
From the equation above, we can express V2:
The tension in the cord when the radius is R2 can be expressed using the centripetal force formula:
The maximum tension before the cord breaks is given as 600 N, so we set up the equation:
Now, we can solve for R32:
Calculating R2:
Thus, the radius of the circle when the cord breaks is approximately:
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 1
9 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 4
11 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI
78 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)
19 Videos
Similar Questions
A small block of mass 4kg is attached to a cord passing through a hole in a horizontal frictionless surface. The block is originally revolving in a circle of radius 0.5m about the hole with a tangential velocity of 4m/s. The cord is then pulled slowly from below, shorting the radius of the circle in which the block revolves. The breaking strength of the cord is 600N. What will be radius of the circle when the cord breaks?
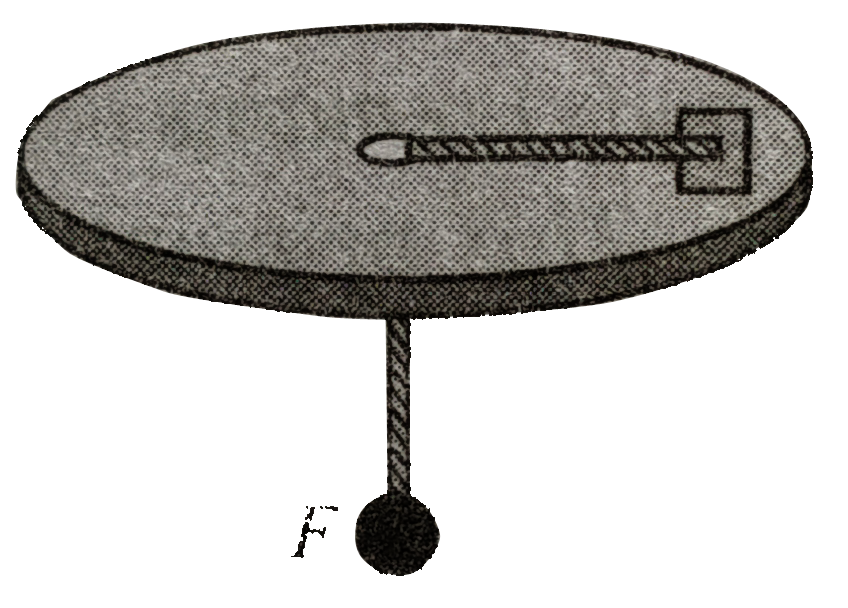
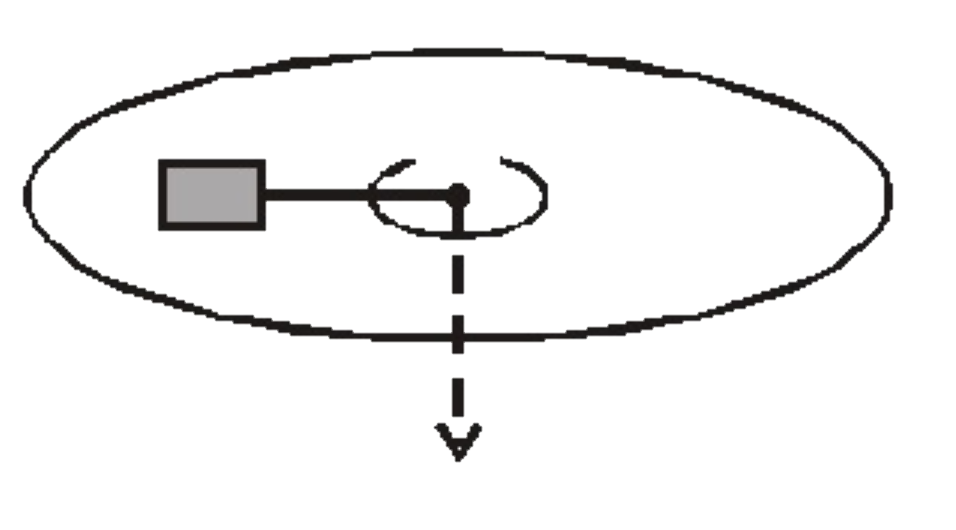
View SolutionA block of mass 0.1 kg is attached to a cord passing through a hole in a horizontal frictionless horizontal surface as shown in the figure. The block is originally revolving at a distance of 0.4 m from the hole, with an angular velocity of 2 rad s−1. The cord is then pulled from below, shortening the radius of the circle in which the block revolves to 0.2 m. Considering the block to be point mass, calculate the new angular velocity (in rad s−1)
View Solution
Knowledge Check
A small block of mass 4kg is attached to a cord passing through a hole in a horizontal frictionless surface. The block is originally revolving in a circle of radius of 5m about the hole, with a tangential velocity of 4m/s. The cord is then pulled slowly from below, shortening the radius of the circle in which the block revolves. The breaking strength of the cord is 200N.
What will be the radius of the circle when the cord breaks?A4.0mB1.0mC3.0mD2.0mA small block of mass 4kg is attached to a cord passing through a hole in a horizontal frictionless surface. The block is originally revolving in a circle of radius of 5m about the hole, with a tangential velocity of 4m/s. The cord is then pulled slowly from below, shortening the radius of the circle in which the block revolves. The breaking strength of the cord is 200N.
Velocity of the block at the time of breaking of the stringA10m/sB20m/sC7.5m/sD12.5m/sA puck of mass m=50.0g is attached to a taut cord passing through a small hole in a frictionless, horizontal surface (Fig.) The puck is initially orbiting with speed vi=1.50m/s in a circle of radius ri=0.30m. The cord is then slowly pulled from below, decreasing the radius of the circle to r=0.10m. The puck's speed at the smaller radius is.
 .A2.25m/sB5.50m/sC4.50m/sD6.0m/s
.A2.25m/sB5.50m/sC4.50m/sD6.0m/s
A small block of mass m on a horizontal smooth table is attached to a light string passing through a hole as shown in the figure. Initially, the block moves in a circle of radius r with speed v and the string is held by a person. The person pulls the strin slowly to decrease the radius of circle to r/2. (a) Find the tension in the string when the block moves in a circle of radius r/2. (b) Calculate the change in the kinetic energy of the block.

View SolutionFigure shows a mass m placed on a frictionless horizontal table and attached toa string passing through a mall hole in the surface. Initially, themas movesin a circle of radius r0 with a speed v0 and the ree end of the string is held by a person. The person pulls on the string slowly to decrease the radius of th circle of r. a. Find the tension in the string when the mass moves in the circle of radius r. b. Calculate the chasnge in the kinetic energy of the mass
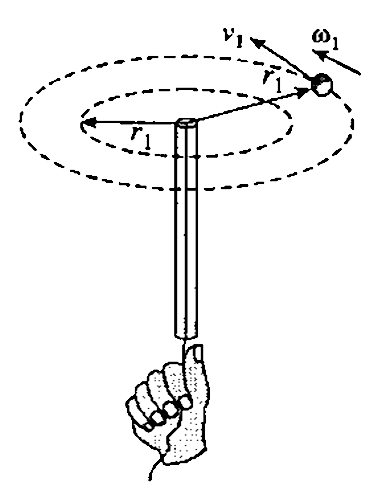
View SolutionA point mass is tied to one end of a cord whose other end passes through a vertical hollow tube , caught in one hand. The point mass is being rotated in a horizontal circle ofradius 2 m with speed of 4m/sec.The cord is then pulled down so that the radius of the circle reduces to 1m . Compute the new linear and angular velocities of the point mass and compute the kinetic energies under the initial and final states.
View SolutionA block of mass m is attached to a pulley disc of equal mass, radius r by means of a slack string as shown. The pulley is hinged about its centre on a horizontal table and the block is projected with an initial velocity of 5m/s. Find the velocity when the string becomes taut.
 .
.
View SolutionA block of mass 2 m is pulled along a horizontal frictionless surface by a rope of mass m as shown in the figure given. What force is exerted by the rope on the block ?

View Solution
