A spring elongates by 2 cm when a piece of cobalt of mass 20g is suspended from it. A water conatiner is placed below the pieces in such a way that the piece is immersed completely in water. Calculate the elongation produced in the spring.
Density of cobalt =8700kg/m3,Useg=10ms2.
To solve the problem step by step, we will follow these calculations:
Step 1: Understand the Forces Acting on the Cobalt Piece
When the cobalt piece is suspended from the spring, two forces act on it:
1. The weight of the cobalt piece (downward force).
2. The spring force (upward force).
Step 2: Calculate the Spring Constant (k)
Given that the spring elongates by 2 cm (0.02 m) when a 20 g (0.02 kg) cobalt piece is suspended, we can use Hooke's Law:
F=kx
Where:
- F is the weight of the cobalt piece, F=mg
- m=20g=0.02kg
- g=10m/s2
- x=2cm=0.02m
Calculating the weight:
F=mg=0.02kg×10m/s2=0.2N
Now, using Hooke's Law:
0.2=k×0.02
Solving for k:
k=0.20.02=10N/m
Step 3: Calculate the Volume of Cobalt
The volume of the cobalt piece can be calculated using its density:
Density=MassVolume⟹Volume=MassDensity
Given:
- Density of cobalt ρc=8700kg/m3
- Mass of cobalt m=0.02kg
Calculating the volume:
V=0.02kg8700kg/m3=0.028700≈2.298×10−6m3
Step 4: Calculate the Buoyant Force
The buoyant force Fb acting on the cobalt piece when it is immersed in water can be calculated using Archimedes' principle:
Fb=ρw⋅V⋅g
Where:
- Density of water ρw=1000kg/m3
- Volume V is the volume of the cobalt piece calculated above
- g=10m/s2
Calculating the buoyant force:
Fb=1000kg/m3⋅2.298×10−6m3⋅10m/s2≈0.023N
Step 5: Calculate the New Equilibrium Condition
When the cobalt piece is fully immersed in water, the forces acting on it are:
1. The weight of the cobalt piece mg (downward).
2. The spring force kx (upward).
3. The buoyant force Fb (upward).
At equilibrium:
mg=kx+Fb
Substituting the known values:
0.2=kx+0.023
We already know k=10N/m:
0.2=10x+0.023
Rearranging gives:
10x=0.2−0.023=0.177
Solving for x:
x=0.17710=0.0177m=1.77cm
Final Answer
The elongation produced in the spring when the cobalt piece is immersed in water is approximately 1.77 cm.
---
When the cobalt piece is suspended from the spring, two forces act on it:
1. The weight of the cobalt piece (downward force).
2. The spring force (upward force).
Given that the spring elongates by 2 cm (0.02 m) when a 20 g (0.02 kg) cobalt piece is suspended, we can use Hooke's Law:
- F is the weight of the cobalt piece, F=mg
- m=20g=0.02kg
- g=10m/s2
- x=2cm=0.02m
The volume of the cobalt piece can be calculated using its density:
- Density of cobalt ρc=8700kg/m3
- Mass of cobalt m=0.02kg
The buoyant force Fb acting on the cobalt piece when it is immersed in water can be calculated using Archimedes' principle:
- Density of water ρw=1000kg/m3
- Volume V is the volume of the cobalt piece calculated above
- g=10m/s2
When the cobalt piece is fully immersed in water, the forces acting on it are:
2. The spring force kx (upward).
3. The buoyant force Fb (upward).
The elongation produced in the spring when the cobalt piece is immersed in water is approximately 1.77 cm.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBook:MODERN PUBLICATIONChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT (Textbook Exercises)
Explore 20 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:MODERN PUBLICATIONChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT (Additional Exercises)
Explore 11 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:MODERN PUBLICATIONChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:Conceptual Questions
Explore 29 Videos
- MATHEMATICAL TOOLSBook:MODERN PUBLICATIONChapter:MATHEMATICAL TOOLSExercise:PRACTICE PROBLEMS (10)
Explore 12 Videos
- MOTION IN A PLANE Book:MODERN PUBLICATIONChapter:MOTION IN A PLANE Exercise:Chapter Practice Test
Explore 15 Videos
Similar Questions
A metal piece of mass 10g is suspended by a vertical spring. The spring elongates 10cm over its natural length to keep the piece in equilibrium. A beaker containing water is now placed below the piece so as to immerse the piece completely in water. Find the elongation of the spring. Density of metal =9000kg/m3. Take g=10m/s2.
View SolutionA copper piece of mas 10 g is suspended by a vertical spring. The sprig elongates 1 cm over its natural lenngth to keep the piece in equilibrium. A beaker containing water is now placed below the piece so as to immerse the piece completely in water. Find the elongation of the spring. Density of copper =9000 kgm^-3. Takeg=10ms−2
View Solution
Knowledge Check
A spring balance reads 10kg when a bucket of water is suspened from it . What will be the reading of the balance when an iron piece of mass 7.2kg suspended by a string is immersed with half its volume inside the water in the bucket? Relative density of iron is 7.2
A10kgB10.5kgC13.6kgD17.2kgIn spring constant of the spring is 100 N/m Extension produced in the spring is:
(g=10m/s2)
 A10 cmB20 cmC30 cmD40 cm
A10 cmB20 cmC30 cmD40 cmA spring is made of material of Young's modulus 1011N/m2. When a weight of 10 kg is suspended vertically the elongation produced in the spring is 4 mm. If the load is reduced to half the elongation in the spring will be
A1 mmB4 mmC2 mmD0.2 mm
A solid of mass 100 g and density 2gcm−3 is immersed in water. Calculate the upthrust acting on it ?
View SolutionA wire gets elongated by 4 mm when a block of mass 2 kg is suspended from it. Now, the block is immersed in water, the wire contracts by 1 mm. Find the density of block when the density of water is 1000kg/m3.
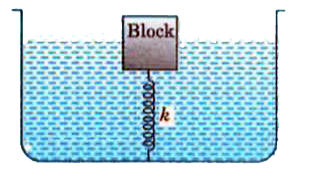
View SolutionA spring is attached to a wooden cubical block of edge 5cm and placed in a water conatiner as shown in figure. Calculate the maximum weight to be placed on the block so that it is just immersed in water completely. Density of wood =700kg/m3, spring constant of spring =70N/m,g=10m/s2. Assume that initially spring is in its normal length.
View SolutionThe wire of a Young's modules appartus is elongated by 2 mm when a brick is suspended from .it When the brick is immersed in water the wire contracts by 0.6mm Calculate the density of the brick given that the density of water is 1000kgm−3
View SolutionA block of bras of mass 0.5 kg and density 8×103kg/m3 is suspended from a string. What will be the tension in the string if the block is completely immersed in water? (g=10ms−2)
View Solution