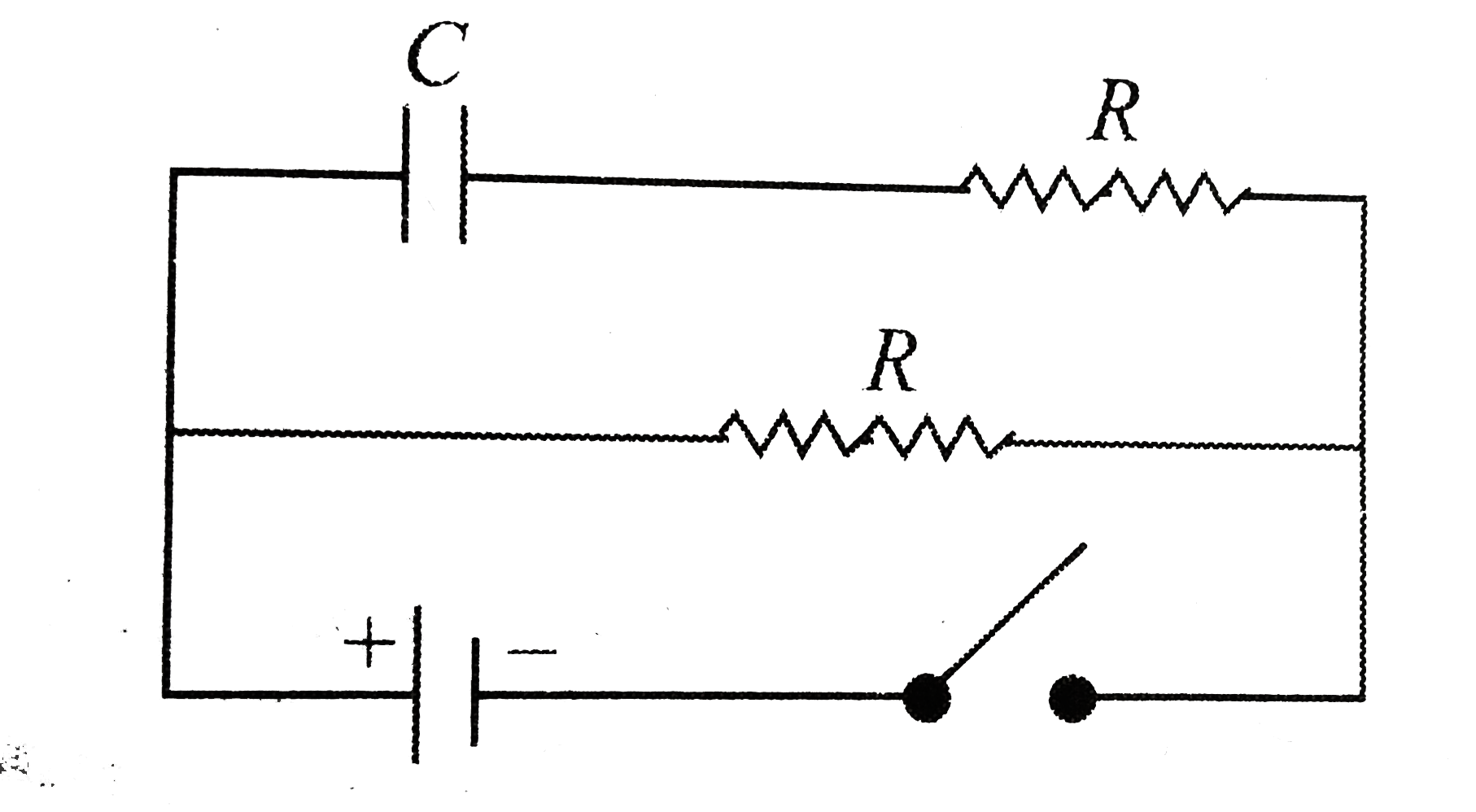
In an LCR circuit as shown in the both switches are open initially. Now switch is closed . kept open (q is charge on the capacitor and = RC is capacitive time constant ). Which of the following statement is correct?

At t = , q = CV/2
At t = , q = CV
At t = , q = CV
Work done by the battery is half of the energy dissipated in the resistor.
The correct Answer is:B
(b) When switch is closed the circuit will behave as RC circuit which starts charging withtime (t) .

Time constant
At
Q .
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - COMPETITION FILE JEE (ADVANCED ) FOR IIT ENTRANCE
4 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - COMPETITION FILE C. MULTIPLE CHOICE QUESTIONS
17 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS) B. MULTIPLE CHOICE QUESTIONS
15 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
Similar Questions
In an LCR circuit as shown below both switches are open initially. Now switch S1 is closed and S2 kept open. (q is charge on the capacitor and τ=RC is Capacitive time constant). Which of the following statement is correct?
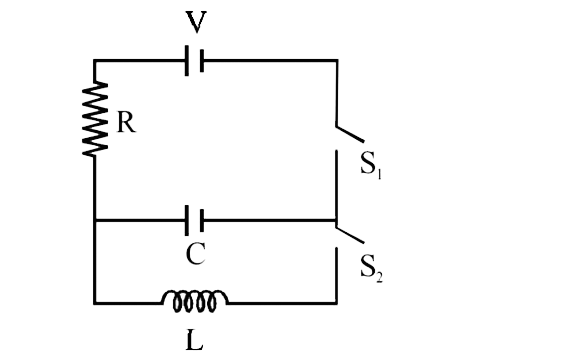
View SolutionIn the given circuit the capacitor (C) may be charged through resistance R by a battery V by closing switch (S1). Also when (S1) is opend and (S2) is closed the capacitor is connected in series with inductor (L).
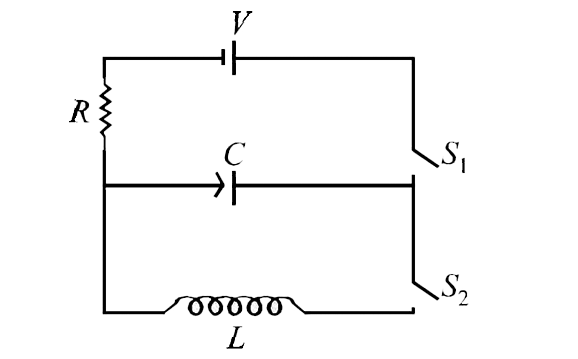
At the start, the capicitor was uncharged. when switch (S1) is closed and (S2) is kept open, the time constant of this circuit is τ. which of the following is correct
View Solution
Knowledge Check
In an LCR circuit as shown below both switches are open initially. Now switch S1 kept open. (q is charge on the capacitor and τ=RC is Capacitive time constant). Which of the following statement is correct?
 AAt t=(τ),q=CV/6BAt t=(τ),q=CV/2CAt t=2(τ),q=CV(1−e−2)DAt t=2(τ),q=CV(1−e−1)
AAt t=(τ),q=CV/6BAt t=(τ),q=CV/2CAt t=2(τ),q=CV(1−e−2)DAt t=2(τ),q=CV(1−e−1)In the given circuit the capacitor (C) may be charged through resistance R by a battery V by closing switch (S1). Also when (S1) is opend and (S2) is closed the capacitor is connected in series with inductor (L).

At the start, the capicitor was uncharged. when switch (S1) is closed and (S2) is kept open, the time constant of this circuit is τ. which of the following is correctAafter interval τ charge on the capcitor is CV2Bafter interval 2τ charge on the capcitor is (CV)(1−e−2)Cthe work done by the voltage source will be half of the heat dissipated when the capacitor is fully chargedDafter time interval 2τ, charge on the capacitor is CV(1−e−1In the circuit shown in the figure, if switches S1 and S2 have been closed for a long time, then charge on the capacitor.
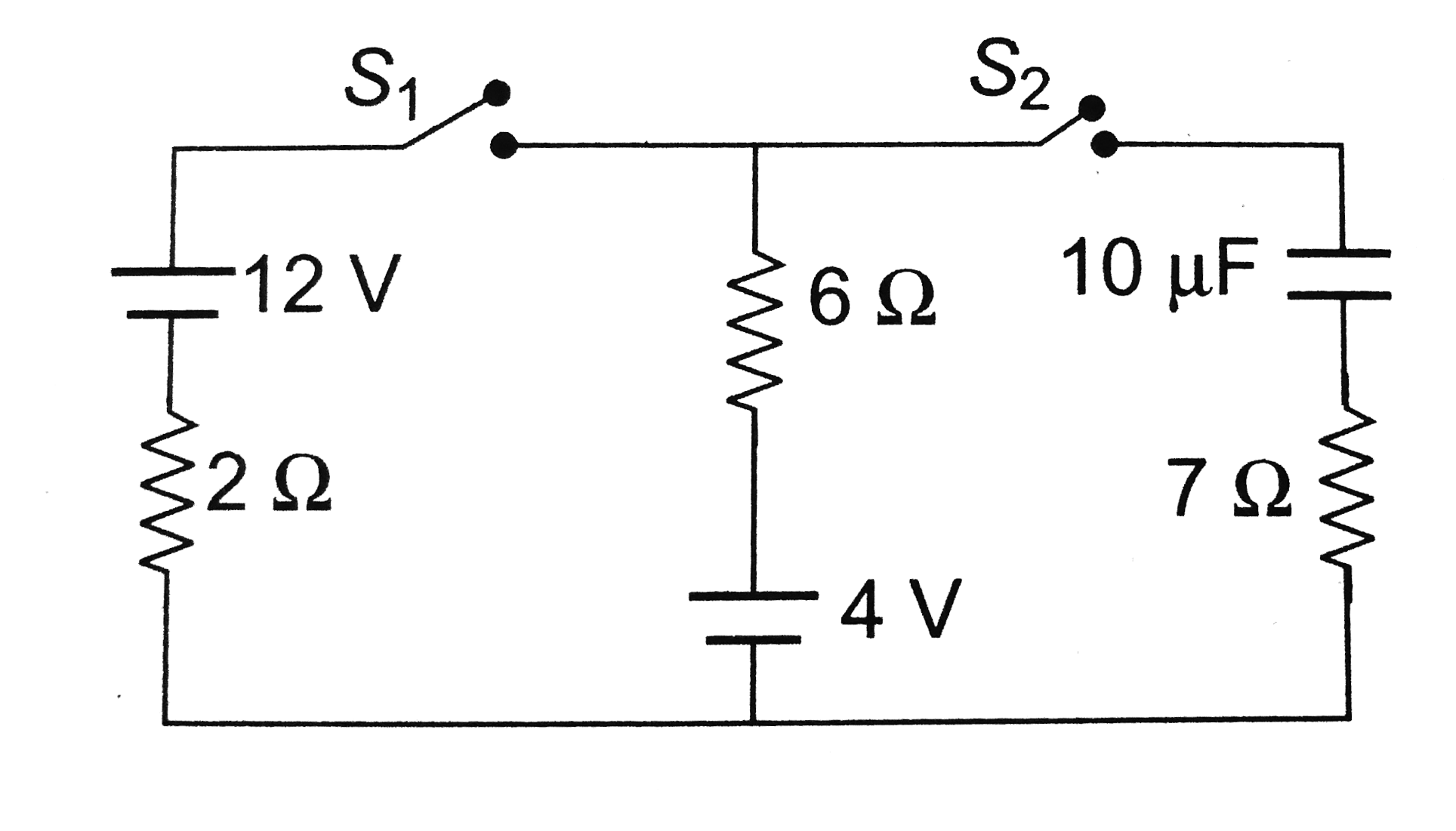 Ais 100μCBincreases to 200μC if one third of the gap of the capacitor's plates is filled with a dielectric (K=2) of same areaCboth (a) and (b) aboveDcharge on the capacitor remains unchanged if one third of the gap of the capacitor's plates is filled with a dielectric (K=2) of same area.
Ais 100μCBincreases to 200μC if one third of the gap of the capacitor's plates is filled with a dielectric (K=2) of same areaCboth (a) and (b) aboveDcharge on the capacitor remains unchanged if one third of the gap of the capacitor's plates is filled with a dielectric (K=2) of same area.
In the circuit shown in the figure, if switches S1 and S2 have been closed for a long time, then charge on the capacitor.
View SolutionThe circuit was in the shown state for a long time. Now if the switch S is closed then the net charge that flows through the switch S, will be

View SolutionIn the circuit shown in fig. when the switch is closed, the capacitor charges with a time constant
View SolutionIn the circuit shown in figure, switch S is initially closed and S is open. Find V-V
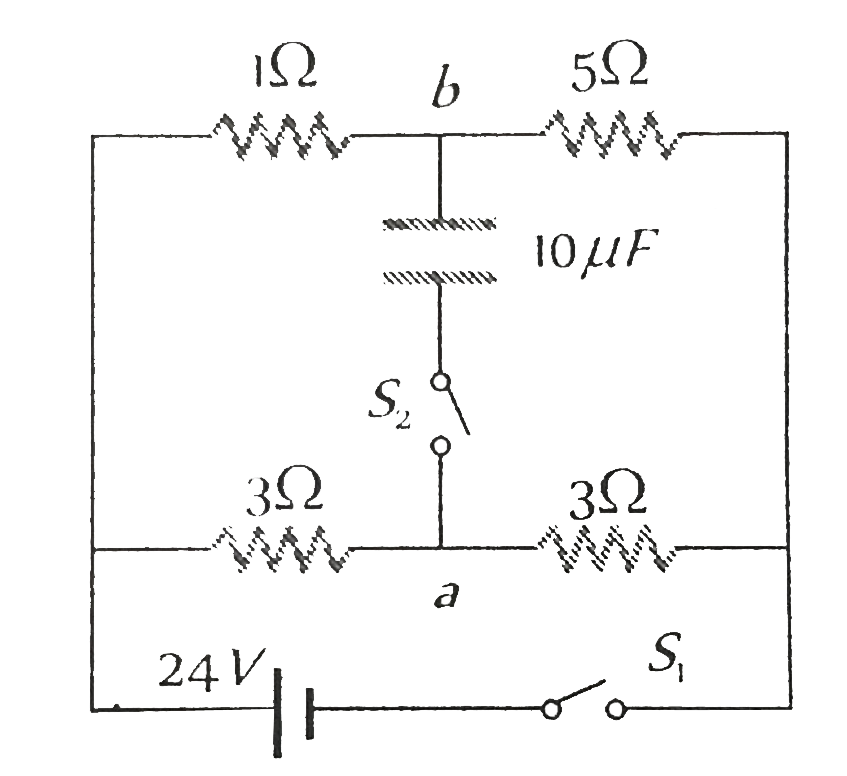
View SolutionThe circuit was in the shown state for a long time. Now if the switch S is closed then the net charge that flows through the switch S, will be

View Solution
