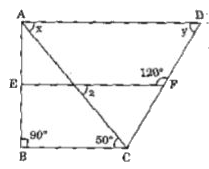
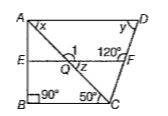
In the figure given above, ABCD is a trepezium. EF is parallel to AD and BC. Then Zy is equal to
The correct Answer is:C
Step by step video, text & image solution for In the figure given above, ABCD is a trepezium. EF is parallel to AD and BC. Then Zy is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.145videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2100videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)52videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 240videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions18videos
HCF AND LCM
Similar Questions
Knowledge Check
- Question 1 - Select One
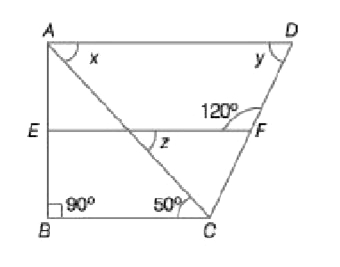
In the figure given below, ABCD is a trapezium, EF is parallel to AD and BC. Then, ∠y equal to
 A30∘B45∘C60∘D65∘
A30∘B45∘C60∘D65∘ - Question 2 - Select One
In the given figure , ABCD is a trapezium in which the parallel sides AB,CD are both perpendicular to BC. Find the area of the trapezium :
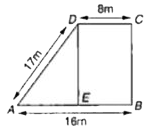 A140m2B168m2C180m2D156.4m2
A140m2B168m2C180m2D156.4m2 - Question 3 - Select One
In the given figure ABCD is trapezium in which the parallel sides AB and CD both are perpendicular to BC. If AB = 16 m, CD = 8 m and AD = 17 M. What is the area of the trapezium ?
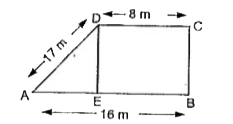 A140 m2B168 m2C180 m2D156.4 m2
A140 m2B168 m2C180 m2D156.4 m2
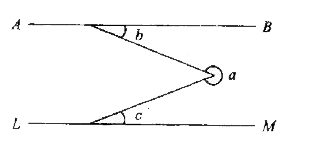
In the figure given above, AB is parallel to LM. What is angle a equal to ?View SolutionIn the figure ABCD is a trapezium in which side AB is parallel to side DC and E is the midpoint of side AD. If F is a point on the side BC such that the line segment EF is parallel to DC.Prove that Fis the mid-point of BC and EF=12(AB+DC)
View Solution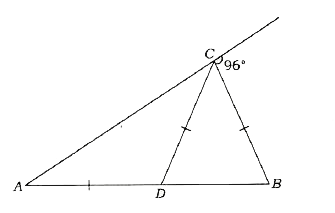
In the figure given above, AD = CD = BC. What is the value of ∠CDB?View Solution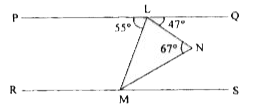
In the figure given above, PQ is parallel to Rs. What is ∠NMS equal toView SolutionIn the given figure , ABCD is a trapezium in which the parallel sides AB,CD are both perpendicular to BC. Find the area of the trapezium :
 View Solution
View Solution