ABCD is a quadrilateral such that BC = BA and CD>AD. Which one of the following is correct ?
∠BAX=∠BCD
∠BAD<∠BCD
∠BAD>∠BCD
2∠BAD=∠BCD
The correct Answer is:C
To solve the problem, we need to analyze the properties of the quadrilateral ABCD based on the given conditions: BC = BA and CD > AD.
1. Draw the Quadrilateral:
- First, sketch the quadrilateral ABCD. Label the points A, B, C, and D appropriately.
2. Identify the Given Conditions:
- We know that BC = BA, which means triangle ABC is isosceles with AB = BC.
- We also know that CD > AD.
3. Analyze Triangle ABC:
- Since AB = BC, the angles opposite these sides must be equal. Therefore, angle BAC = angle BCA.
4. Analyze Triangle ADC:
- In triangle ADC, since CD > AD, the angle opposite to CD (which is angle DAC) must be greater than the angle opposite to AD (which is angle DCA). Thus, angle DAC > angle DCA.
5. Compare Angles:
- We have established that angle BAC = angle BCA from triangle ABC.
- We also know that angle DAC > angle DCA from triangle ADC.
- Since angle DAC is greater than angle DCA, and angle BAC is equal to angle BCA, we can conclude that angle BAD (which is equal to angle BAC) is greater than angle BCD (which is equal to angle DCA).
6. Conclusion:
- Therefore, we conclude that angle BAD > angle BCD.
Final Answer:
The correct statement is that angle BAD is greater than angle BCD.
---
- First, sketch the quadrilateral ABCD. Label the points A, B, C, and D appropriately.
- We know that BC = BA, which means triangle ABC is isosceles with AB = BC.
- We also know that CD > AD.
- Since AB = BC, the angles opposite these sides must be equal. Therefore, angle BAC = angle BCA.
- In triangle ADC, since CD > AD, the angle opposite to CD (which is angle DAC) must be greater than the angle opposite to AD (which is angle DCA). Thus, angle DAC > angle DCA.
- We have established that angle BAC = angle BCA from triangle ABC.
- We also know that angle DAC > angle DCA from triangle ADC.
- Since angle DAC is greater than angle DCA, and angle BAC is equal to angle BCA, we can conclude that angle BAD (which is equal to angle BAC) is greater than angle BCD (which is equal to angle DCA).
- Therefore, we conclude that angle BAD > angle BCD.
The correct statement is that angle BAD is greater than angle BCD.
Topper's Solved these Questions
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.1
45 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2
100 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)
52 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
- HCF AND LCMBOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions
18 Videos
Similar Questions
Knowledge Check
ABCD is a quadrilateral whose diagonals are AC and BD. Which one of the following is correct?
A−−→BA+−−→CD=−−→AC+−−→DBB−−→BA+−−→CD=−−→BD+−−→CAC−−→BA+−−→CD=−−→AC+−−→BDD−−→BA+−−→CD=−−→BC+−−→ADABCD is a quadrilateral whose diagonals are AC and BD. Which one of the following is correct?
AA. −−→BA+−−→CD=−−→AC+−−→DBBB. −−→BA+−−→CD=−−→BD+−−→CACC. −−→BA+−−→CD=−−→AC+−−→BDDD. −−→BA+−−→CD=−−→BC+−−→ADSides BA and DC of quadrilateral ABCD are extended as per diagram then which one of the follwing is true?
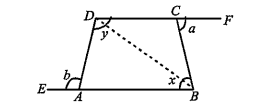Ax+y=a+bBx+y=a-bCx-y=(a+b)/2D(x+y)/2=a-b
ABCD is a quadrilateral. Is AB+BC+CD+DA>AC+BD?
View SolutionThe figure ABCD is a quadrilateral in which AB = CD and BC = AD. Its area is
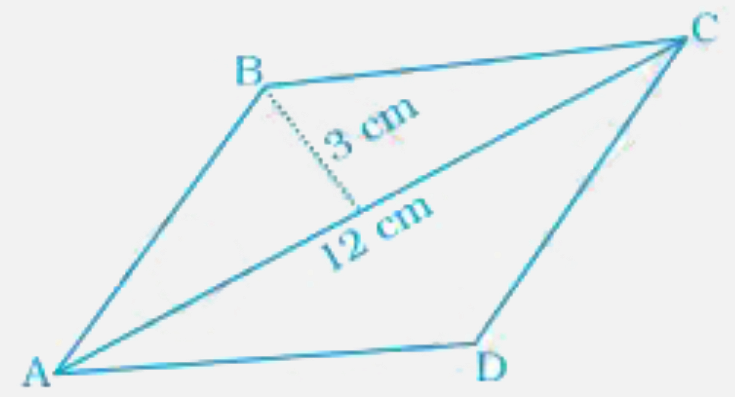
View SolutionIn a quadrilateral ABCD, show that
(AB+BC+CD+DA)>(AC+BD).
View SolutionLet ABCD be a cyclic quadrilateral such that AB=2,BC=3,∠B=120∘ and area of quadrilateral =4√3. Which of the following is/are correct ?
View SolutionIn a quadrilateral ABCD, AC bisects ∠C and BC = CD, then which of the following statement is false?
View Solution