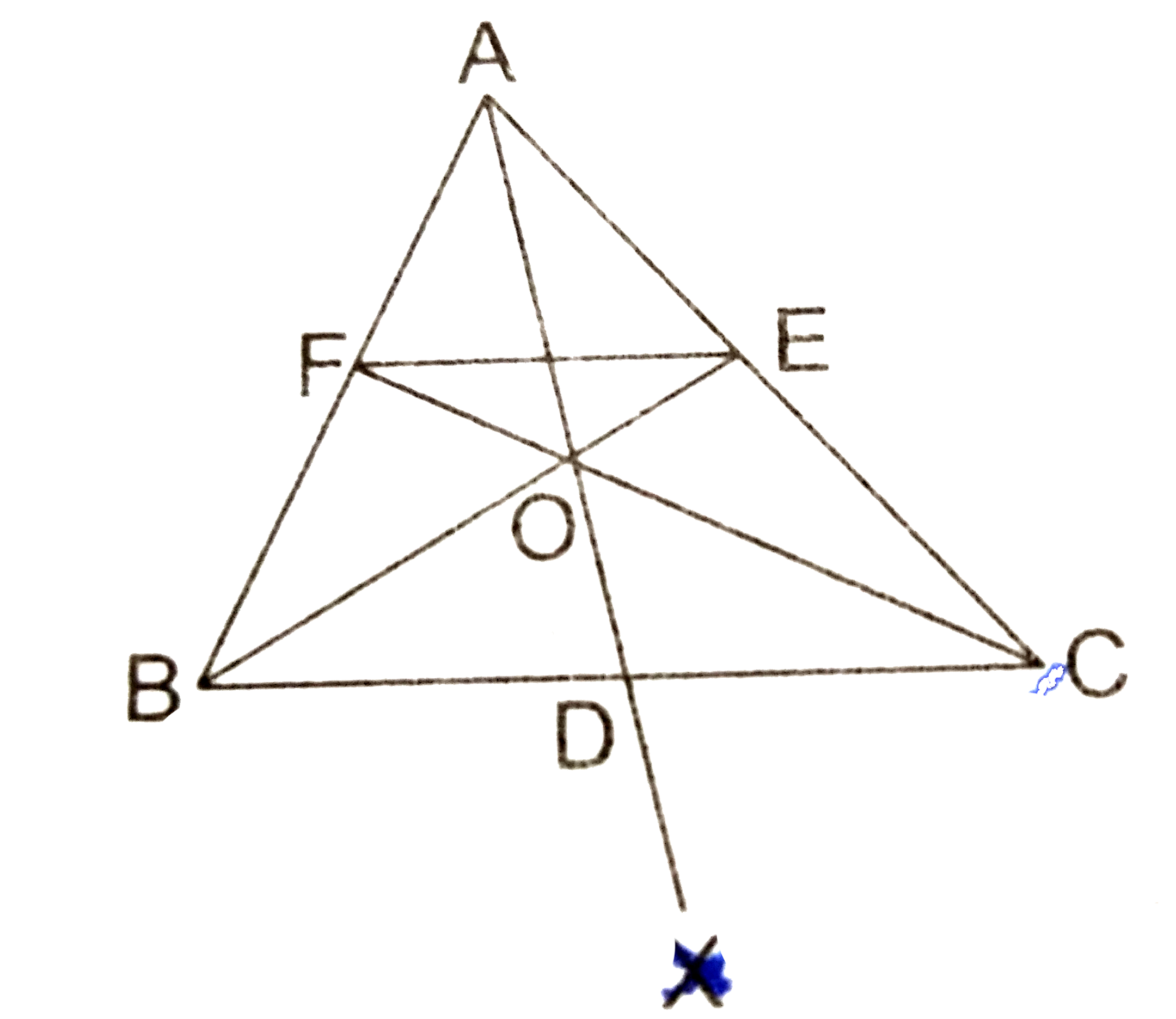
The side BC of a ΔABC is bisected at D. O is any point on AD, BO and CO are produced to meet AC and AB at E and F respectively and AD is produced to G so that D is the midpoint of OG. Prove that FE∣∣BC
Step by step video & image solution for The side BC of a DeltaABC is bisected at D. O is any point on AD, BO and CO are produced to meet AC and AB at E and F respectively and AD is produced to G so that D is the midpoint of OG. Prove that FE|| BC by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Similar Questions
The side BC of a ΔABC is bisected at D. O is any point on AD. BO and CO are produced to meet AC and AB at E & F respectively. AD is produced to C1 so that D is the mid-point of OC1. Prove that FE||BC.
View SolutionThe side BC of a triangle ABC is bisected at D;o is any point in AD,BOandCO produced meet ACandAB in EandF respectively and AD is produced to X so that D is the mid-point of OX. Prove that AO:AX=AF:AB and show that FEBC.
View Solution
Knowledge Check
A circle touches the side BC of ΔABC at D and AB and aC are produced to E and F, respectively. If AB=10cm, AC=8.6 cm and BC=6.4 cm then BE=?
A3.5cmB3.2cmC2.5cmD2.2cm
The side BC of a triangle ABC is bisected at D;O is any point in AD.BOandCO produced meet ACandAB in EandF respectively and AD is produced to X so that D is the mid-point of OX. Prove that AO:AX=AF:AB and show that FE∣∣BC.
View SolutionIn the given figure, side BC of ΔABC is bisected at D and O is any point AD.BO and CO produced meet AC and AB at E and F respectively, and AD is respectively, and AD is produced to X so that D is the midpoint of OX. Prove that AO:AX=AF:AB and show that EF∣∣BC.
View SolutionIn a triangle ABC,D is the mid - point of BC, AD is produced upto E so that DE = AD.
Prove that :
AB=EC
View SolutionAD is a median of ΔABC . The bisector of ∠ADB and ∠ADC meet AB and AC in E and F respectively. Prove that EF||BC
View SolutionAD is a median of △ABC. The bisectors of ∠ADBand∠ADC meets AB and AC in E and F respectively prove that EF||BC.
View SolutionIn ΔABC,D is the mid-pointpoint of BC and E is mid-point of AD.BE is produced to meet the side AC in F.Then BF is equal to
View SolutionD is the mid-point of side BC of a A ABC. AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE : EX = 3:1
View Solution
