In △ABC,∠B=90∘, P,Q and R are the mid-points of ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC and ¯¯¯¯¯¯AC respectively. Then which of the following is true?
A,P,Q and R are concylic points.
B,P,R and Q are concylic points.
C,Q,P and R are concyclic points.
All of these
The correct Answer is:B
i) Draw the figure and mark the points P,Q and R on sides AB, BC and AC respectively.
ii) Angle B=90∘ and ∠PRQ is formed by lines PR and RQ, which are parallel to lines BC and AB (mid-point theorem).
iii) Since BC and AB are perpendicular, PR and RQ are also perpendicular , i.e., ∠PRQ=90∘.
iv) Quadrilateral joining BPRQ is a cyclic quadrilateral.
Topper's Solved these Questions
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - SHORT ANSWER TYPE QUESTIONS18videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - Level 39videos
COORDINATE GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS AND INEQUATIONSEXERCISE - level 310videos
LINEAR EQUATIONS AND INEQUATIONS
Similar Questions
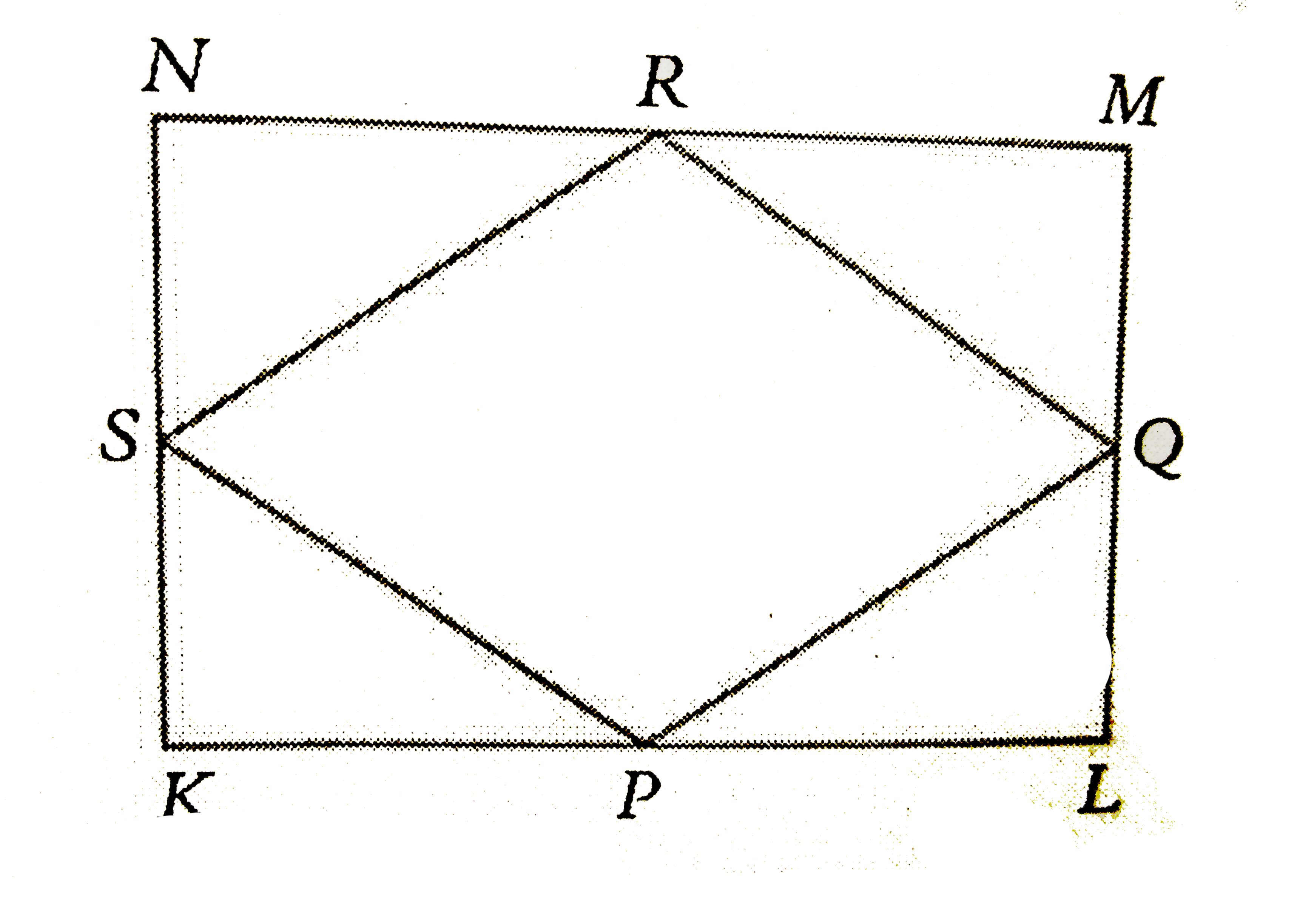
In the figure above, KLMN is a rectangle . P,Q,R and S are the mid-points of ¯¯¯¯¯¯KL,¯¯¯¯¯¯¯LM,¯¯¯¯¯¯¯¯MN,and¯¯¯¯¯¯¯NK, respectively. If ∠KPS=30∘, then find ∠QRS.View Solution-
In the figure above, KLMN is a rectangle . P,Q,R and S are the mid-points of ¯¯¯¯¯¯KL,¯¯¯¯¯¯¯LM,¯¯¯¯¯¯¯¯MN,and¯¯¯¯¯¯¯NK, respectively. If ∠KPS=30∘, then find ∠QRS.View Solution
Knowledge Check
- Question 1 - Select One
In a triangle PQR, PQ=QR, A and B are the mid-points of ¯¯¯¯¯¯QR and ¯¯¯¯¯¯PR respectively. A circle passes through P,Q, A and B. Then which of the following is necessarily true?
A△ is equilateralB△ is right isoscelesCPQ is a diameterDBoth a) and c) - Question 2 - Select One
In right angled ΔABC,∠B=90∘, if P and Q are points on the sides AB and BC respectively, then :
AAQ2+CP2=2(AC2+PQ2)BAQ2+CP2=AC2+PQ2C(AQ2+CP2)=12(AC2+PQ2)D(AQ+CP)=12(AC+PQ) - Question 3 - Select One
In ΔABC,∠B=90∘. If P is the mid-point of side AC, then
PA=PB=AACB3ACC12ACD2AC
In triangle ABC,∠C=90∘,AC=6andBC=8. Points D and E are on ¯¯¯¯¯¯ABand¯¯¯¯¯¯BC , respectively, and ∠BED=90∘. If DE=4, then BD=
View SolutionIf D is the mid -point of side AB of ΔABC, then ¯¯¯¯¯¯AB+¯¯¯¯¯¯BC+¯¯¯¯¯¯AC=
View SolutionTaken on side ¯¯¯¯¯¯AC of a ΔABC, a point M such that ¯¯¯¯¯¯¯AM=13¯¯¯¯¯¯AC. A point N is taken c the side BC such that ¯¯¯¯¯¯BN=¯¯¯¯¯¯CB, then for the point of intersection X of ¯¯¯¯¯¯AB and ¯¯¯¯¯¯¯¯MN, which of the following is true
View SolutionABC is an equilateral triangle. P and Q are two points on ¯¯¯¯¯¯AB and ¯¯¯¯¯¯AC respectively such that ¯¯¯¯¯¯PQ∣∣¯¯¯¯¯¯BC. If ¯¯¯¯¯¯PQ=5cm, then area of ΔAPQ is :
View SolutionIf ABCD is a square, then
¯¯¯¯¯¯AB+2¯¯¯¯¯¯BC+3¯¯¯¯¯¯CD+4¯¯¯¯¯¯DA =View Solution