A rod of length l is in motion such that its ends A and B are moving along x-axis and y-axis respectively. It is given that red/sec always. P is a fixed point on the rod. Let M be the projection of P on x-axis. For the time interval in which changes from 0 to , choose the correct statement.

The acceleration of M is always directed towards right
M executes SHM
M moves with constant speed
M moves with constant acceleration
The correct Answer is:B
Let BP = a,
Hence M executes SHM within the given time period and its acceleration is opposite to x that means towards left.

Topper's Solved these Questions
- OSCILLATIONS Book:DISHA PUBLICATIONChapter:OSCILLATIONS Exercise:Exercise-1 : Concept Builder (TOPIC 3: Damped & Forced Oscillations and Resonance)
Explore 11 Videos
- NUCLEIBook:DISHA PUBLICATIONChapter:NUCLEIExercise:EXERCISE - 2 (CONCEPT APPLICATOR)
Explore 30 Videos
- PHYSICAL WORLD, UNITS AND MEASUREMENTSBook:DISHA PUBLICATIONChapter:PHYSICAL WORLD, UNITS AND MEASUREMENTSExercise:Exercise - 2 : Concept Applicator
Explore 30 Videos
Similar Questions
A rod of length l is in motion such that its ends A and B are moving along x-axis and y-axis respectively. It is given that dθdt=2 rad/sec always . P is a fixed point on the rod . Let M be the projection of P on x-axis . For the time interval in which θ changes from 0 to π2 , the correct statement is
View SolutionA light rigid rod of length l is constrained to move in a vertical plane, so that its ends are along the x and y axes respectively. Find the instantaneous axis of rotation of the rod when it makes an angle θ with horizontal.
View Solution
Knowledge Check
The equation of the line which cuts off intercepts 2asecθand2acosecθ on X-axis and Y-axis respectively is
Axsinθ+ycosθ−2a=0Bxcosθ+ysinθ−2a=0Cxsecθ+ycosecθ−2a=0Dxcosecθ+ysecθ−2a=0Three identical rods, each of mass m and length l are placed along x, y and z axis respectively. One end of each rod is at the origin. The moment of inertia of the rods x-axis will be
Aml2B5ml23C2ml23Dml23
A light rigid rod of length l is constrained to move in a vertical plane, so that its ends are along the x and y axes respectively. Find the instantaneous axis of rotation of the rod when it makes an angle θ with horizontal.
View SolutionFind the length of the foot of perpendicular drawn from the point P(a, b, c) on x-axis, y-axis and
z-axis respectively.
View SolutionTwo rods of lengths a and b slide along the x-axis and y-axis respectively in such a manner that their ends are concyclic. The locus of the centre of the circle passing through the end points is:
View SolutionA rod of length l cm moves with its ends A (on x=-axis) and B (on y-axis) always touching the coordinae axes. Prove that the point P on the rod which divides AB in the ratio λ(≠1) is ellipse. Alos, find the eccentricity of the ellipse.
View SolutionA rod of length l cm moves with its ends A (on x=-axis) and B (on y-axis) always touching the coordinae axes. Prove that the point P on the rod which divides AB in the ratio λ(≠1) is ellipse. Alos, find the eccentricity of the ellipse.
View SolutionA thin conducting rod of length l=5m is moved such that its end B moves along the X-axis while end A moves along the Y-axis A uniform magnetic field B=6ˆk T exists
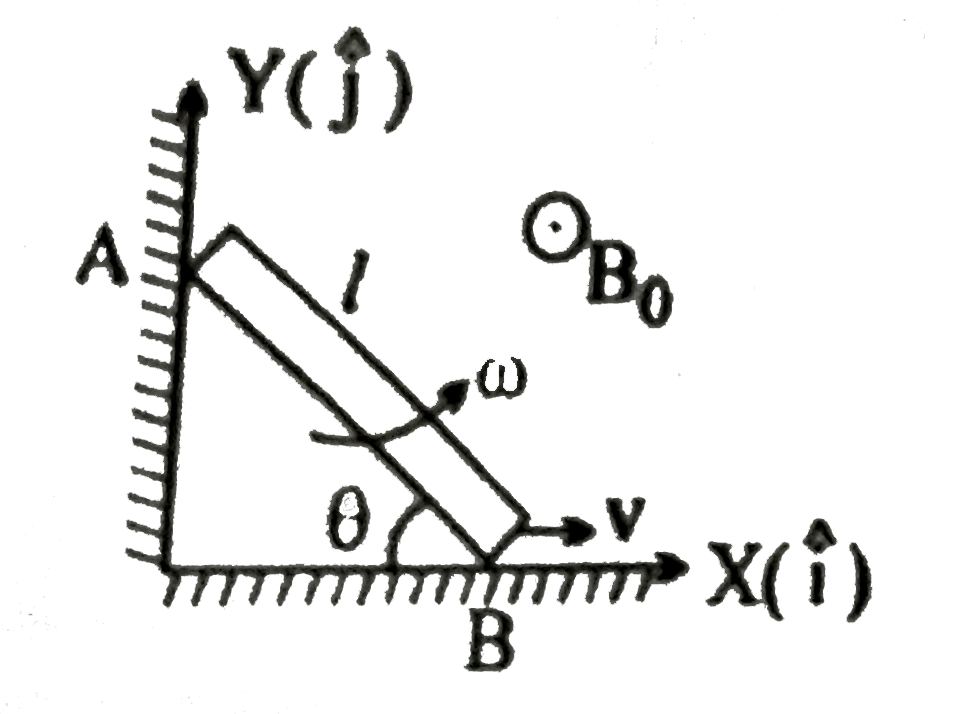
View Solution
