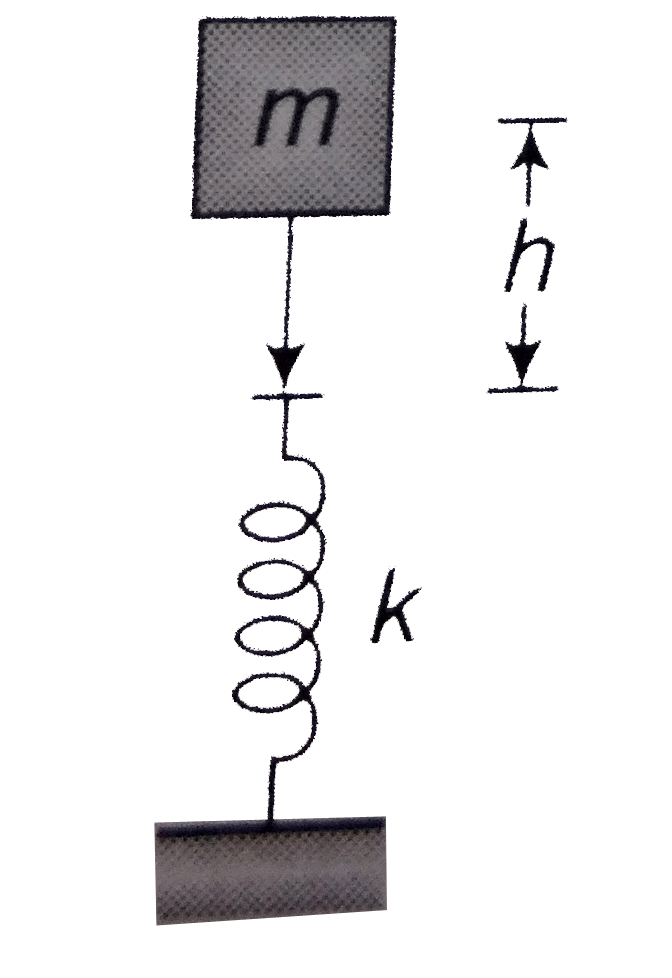
A mass m fall on spring of spring constant k and negligible mass from a height h. Assuming it sticks to the pan and executes simple harmonic motion, the maximum height upto which the pan will rise is

$\frac{m g}{k}$

$\frac{m g}{k} [\sqrt{1 + \frac{2 k h}{m g}} - 1]$

$\frac{m g}{k} [\sqrt{1 + \frac{2 k h}{m g}} + 1]$

$\frac{m g}{k} [\sqrt{1 + \frac{k h}{m g}} - 1]$

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

A body of mass m falls from a height h onto the pan of a spring balance. The masses of the pan and spring are negligible. The force constant of the spring is k. The body sticks to the pan and oscillates simple harmonically. The amplitude of oscillation is

A $\frac{m g}{k}$
B $\frac{m g}{k} \sqrt{1 + \frac{2 h k}{m g}}$
C $\frac{m g}{k} (1 + \sqrt{1 + \frac{2 h k}{m g}})$
D $\frac{m g}{k} \sqrt{1 + (\frac{2 h k}{m g}) - 1}$
Two springs of constants $k_{1}$ and $k_{2}$ have equal maximum velocities, when executing simple harmonic motion. The ratio of their amplitudes (masses are equal) will be
A $\frac{k_{1}}{k_{2}}$
B $\frac{k_{2}}{k_{1}}$
C ${(\frac{k_{1}}{k_{2}})}^{1 / 2}$
D ${(\frac{k_{2}}{k_{1}})}^{1 / 2}$
A 0.20kg object mass attached to a spring whose spring constant is 500N/m executes simple harmonic motion. If its maximum speed is 5.0m/s, the amplitude of its oscillation is
A0.0020m
B0.10m
C0.20m
D25m