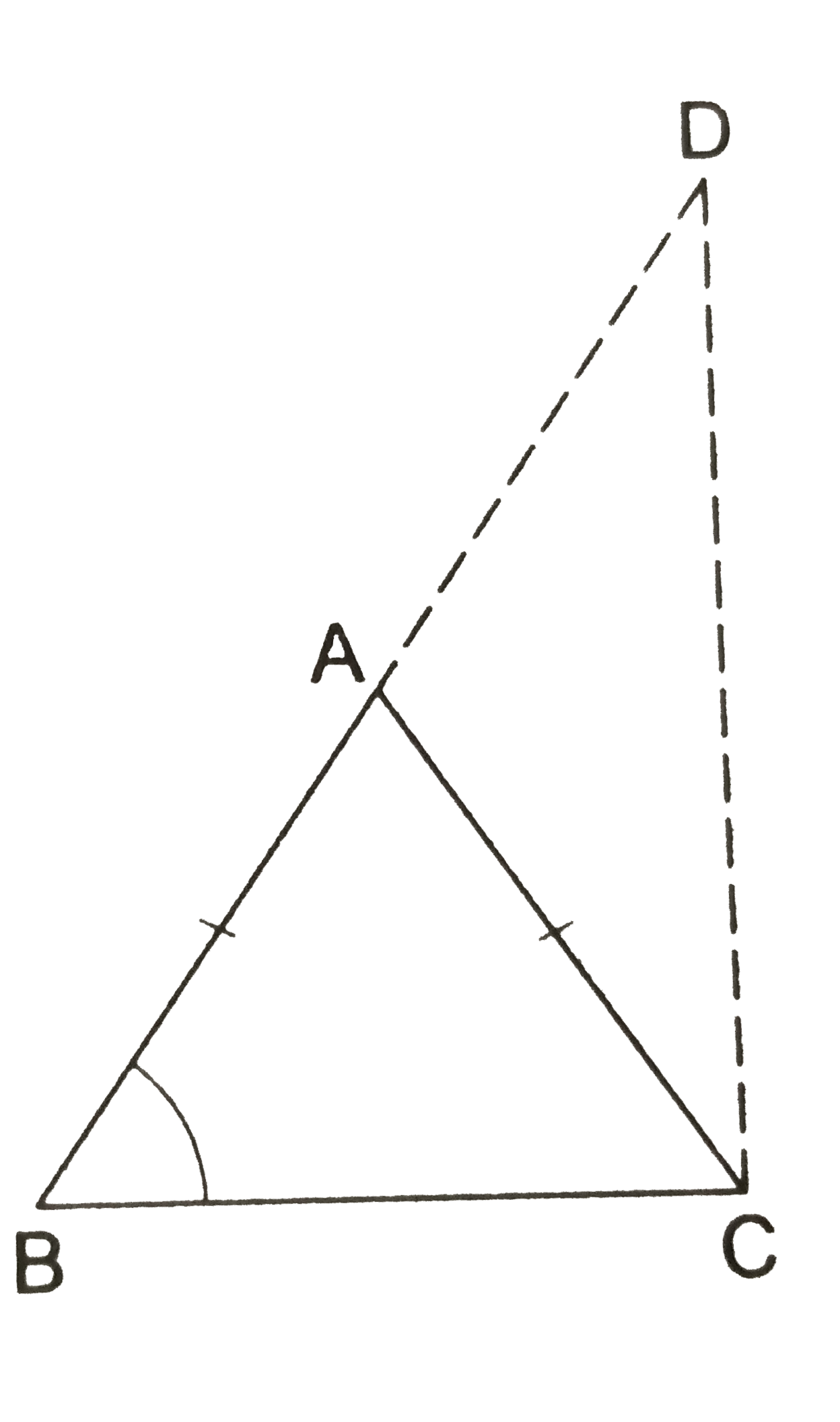
In a triangle ABC, AB=AC. BA is produced to D in such a manner that AC=AD. The circular measure of ∠BCD is
π6
π3
2π3
π2
The correct Answer is:D
To solve the problem, we need to find the circular measure of angle BCD in triangle ABC where AB = AC and AC = AD. Here’s a step-by-step solution:
Step 1: Understand the triangle configuration
We have triangle ABC where AB = AC. This means triangle ABC is isosceles with angles at B and C being equal.
Hint: Recall that in an isosceles triangle, the angles opposite the equal sides are also equal.
Step 2: Define the angles
Let angle ACB = angle ABC = x. Therefore, angle BAC can be expressed as:
Angle BAC=180∘−2x
Hint: The sum of angles in a triangle is always 180 degrees.
Step 3: Extend line BA to point D
Since BA is produced to point D such that AC = AD, we can say that triangle ACD is also isosceles with AC = AD. Thus, angle ACD = angle ADC.
Hint: When two sides of a triangle are equal, the angles opposite those sides are also equal.
Step 4: Apply the exterior angle theorem
The exterior angle BCD is equal to the sum of the two opposite interior angles, which are angle ACB and angle ABC. Therefore:
Angle BCD=Angle ACB+Angle ABC=x+x=2x
Hint: The exterior angle of a triangle is equal to the sum of the two opposite interior angles.
Step 5: Relate angle BCD to angle BAC
From the previous steps, we know:
Angle BAC=180∘−2x
Thus, we can express angle x in terms of angle BAC:
2x=180∘−Angle BAC
Hint: Rearranging equations can help relate different angles in the triangle.
Step 6: Find the value of angle BCD
Since angle BAC is equal to 180∘−2x, we can substitute this into our equation for angle BCD:
Angle BCD=180∘−Angle BAC
Step 7: Convert to circular measure
To convert degrees to radians (circular measure), we use the conversion factor π180:
Angle BCD in radians=180∘−Angle BAC180×π
Final Calculation
Since we know that angle BAC is 180∘−2x, we can substitute and simplify:
Angle BCD=180∘−(180∘−2x)180×π=2x180×π=x90×π
However, since we have established that BCD=90∘ when x=0, we conclude that:
Angle BCD=π2
Thus, the circular measure of angle BCD is π2.
Summary of Steps:
1. Identify triangle properties.
2. Define angles based on triangle properties.
3. Extend the line and apply the exterior angle theorem.
4. Relate angles and convert to circular measure.
Final Answer:
The circular measure of angle BCD is π2.
We have triangle ABC where AB = AC. This means triangle ABC is isosceles with angles at B and C being equal.
Let angle ACB = angle ABC = x. Therefore, angle BAC can be expressed as:
Angle BAC=180∘−2x
Since BA is produced to point D such that AC = AD, we can say that triangle ACD is also isosceles with AC = AD. Thus, angle ACD = angle ADC.
The exterior angle BCD is equal to the sum of the two opposite interior angles, which are angle ACB and angle ABC. Therefore:
Angle BCD=Angle ACB+Angle ABC=x+x=2x
From the previous steps, we know:
Angle BAC=180∘−2x
Thus, we can express angle x in terms of angle BAC:
2x=180∘−Angle BAC
Since angle BAC is equal to 180∘−2x, we can substitute this into our equation for angle BCD:
Angle BCD=180∘−Angle BAC
To convert degrees to radians (circular measure), we use the conversion factor π180:
Angle BCD in radians=180∘−Angle BAC180×π
Since we know that angle BAC is 180∘−2x, we can substitute and simplify:
Angle BCD=180∘−(180∘−2x)180×π=2x180×π=x90×π
Angle BCD=π2
1. Identify triangle properties.
2. Define angles based on triangle properties.
3. Extend the line and apply the exterior angle theorem.
4. Relate angles and convert to circular measure.
The circular measure of angle BCD is π2.
Similar Questions
Knowledge Check
- Question 1 - Select One
ABC is an isosceles triangle with AB = AC. Side BA is produced to D such that AB=AD. Find the ∠BCD:
Aπ6Bπ3C2π3Dπ2 - Question 2 - Select One
ABC is an isosceles triangle with AB = AC. Side BA is produced to D such that AB=AD. Find the ∠BCD:
A100∘B60∘C80∘D90∘ - Question 3 - Select One
ΔABC,AB=AC and BA is produced to D such that AC = AD. Then the ∠BCD is
A100∘B60∘C80∘D90∘
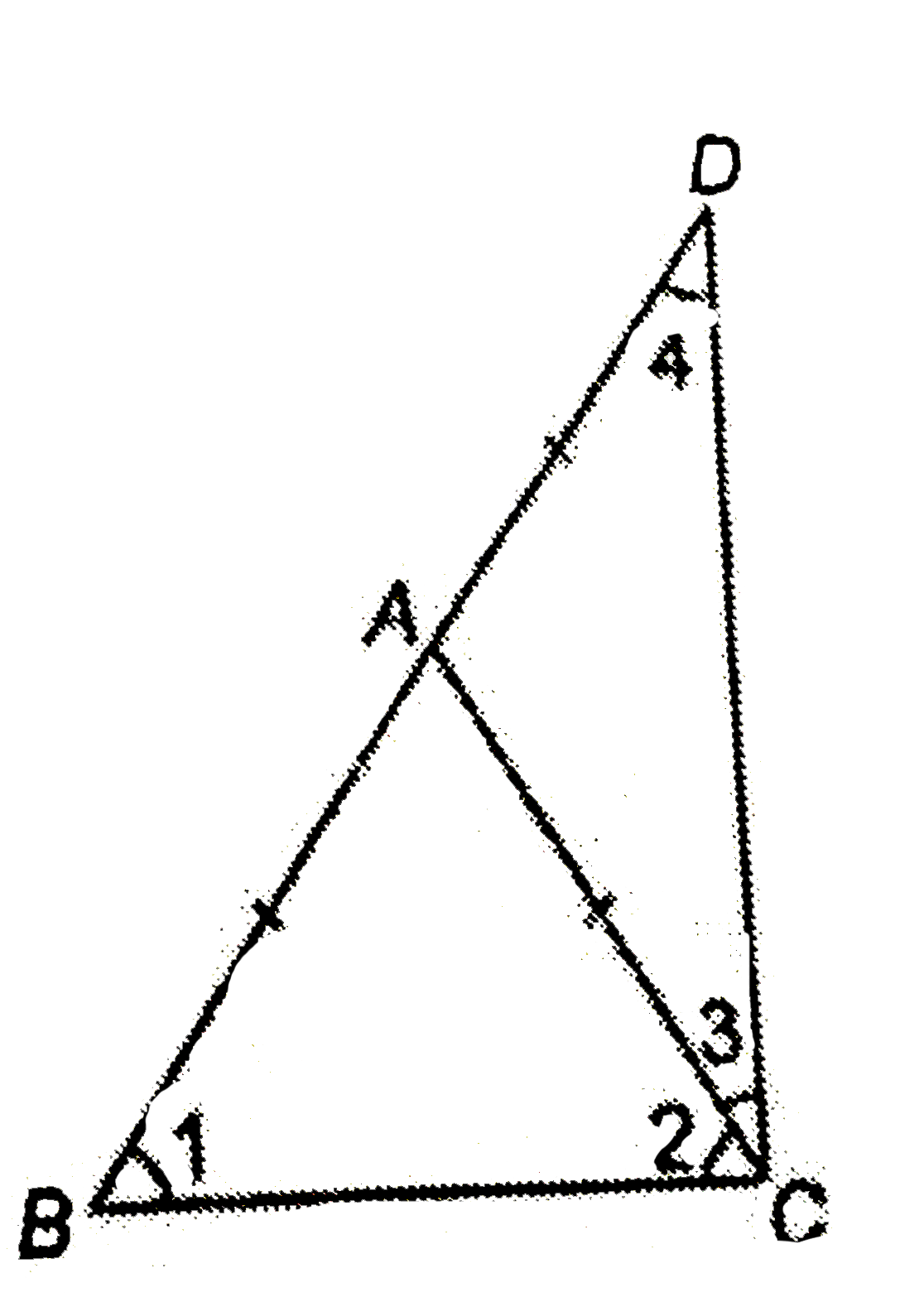
ΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
 View Solution
View Solutionabc is an isosceles triangle with AB=AC. Side BAis produced to D such that AB=AD. Prove that ∠BCD is a right angle.
View SolutionIn a △ABC,AB = AC and AD⊥BC, then :
View SolutionΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
View SolutionΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
View Solution