The vertices of a triangle are
,, .
Find the orthocentre of the triangle.
Video Solution
Text Solution
Let be a triangle whose vertices are , and .
Then, Slope of
Slope of
So, the equation of a line through A perpendicular to is ........
and the equation of a line through perpendicular to is
.........
The point of intersection of Eqs. and , is the orthocentre.
On subtracting Eq. from Eq. , we get .
On putting in Eq. , we get
Hence, the coordinates of the orthocentree are .
|
Topper's Solved these Questions
- STRAIGHT LINE AND PAIR OF STRAIGHT LINESBOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - VARIOUS FORMS OF STRAIGHT LINE (Integer Answer Type Question)
1 Videos
- STRAIGHT LINE AND PAIR OF STRAIGHT LINESBOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - ANGLE BETWEEN STRAIGHT LINE AND EQUATION OF ANGLE BISECTOR (Objective Questions II) (One or more than one correct option)
3 Videos
- STRAIGHT LINE AND PAIR OF STRAIGHT LINESBOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - VARIOUS FORMS OF STRAIGHT LINE (True/False)
3 Videos
- SOLVED PAPER 2019BOOK - IIT JEE PREVIOUS YEARCHAPTER - SOLVED PAPER 2019EXERCISE - Paper -2 section-3
4 Videos
- THEORY OF EQUATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - THEORY OF EQUATIONSEXERCISE - SOME SPECIAL FORMS
18 Videos
Similar Questions
Knowledge Check
If O is the orthocentre of triangle ABC whose vertices are at A(at21,2at1,B(at22,2at2)andC(at23,2at3) then the coordinates of the orthocentreof ΔO'BC are
A(a(t21+t22+t23),2a(t1+t2+t3))B(−a,0)C(at21,2at1)D(0,a)In any progression, if t2t3t1t4=t2+t3t1+t4=3(t2−t3t1−t4), then t1,t2,t3,t4 are in
AA.P.BG.P.CH.P.Dnone
The vertices of a triangle are [at1t2,a(t1+t2)], [at2t3,a(t2+t3)], [at3t1,a(t3+t1)] Then the orthocenter of the triangle is (a) (−a,a(t1+t2+t3)−at1t2t3) (b) (−a,a(t1+t2+t3)+at1t2t3) (c) (a,a(t1+t2+t3)+at1t2t3) (d) (a,a(t1+t2+t3)−at1t2t3)
View SolutionFind the area of that triangle whose vertices are (at21,2at1),(at22,2at2)and(at23,2at3).
View SolutionFind the are of triangle whose vertex are: (at1,at1)(at2,at2)(at3,at3)
View SolutionFind the coordinates o the vertices of a triangle, the equations of whose sides are: y(t1+t2)=2x+2at1at2, y(t2+t3)=2x+2at2t3 and ,y(t3+t1)=2x+2at1t3.
View SolutionProve that the area of the triangle whose vertices are :
(at21,2at1), (at22,2at2), (at23,2at3) is a2(t1−t2)(t2−t3)(t3−t1).
View Solution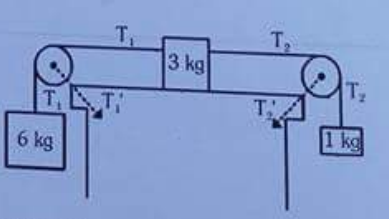
Calculate a, T1,T2,T1'&T2'.
View Solution
