In the figure given below, ΔPQR is right-angled at Q and the points S and T trisect the side QR. Prove that 8PT2=3PR2+5PS2
GIVEN AΔPQR in which ∠PQR=90∘, S and T are the points of trisection of QR.
TO PROVE 8TP2=3PR2+5PS2
PROOF Let AQ=ST=TR=x. Then,
QS=x,QT=2xandQR=3x.
From right triangle PQS,PQTandPQR by Pythagora's theorem we have,
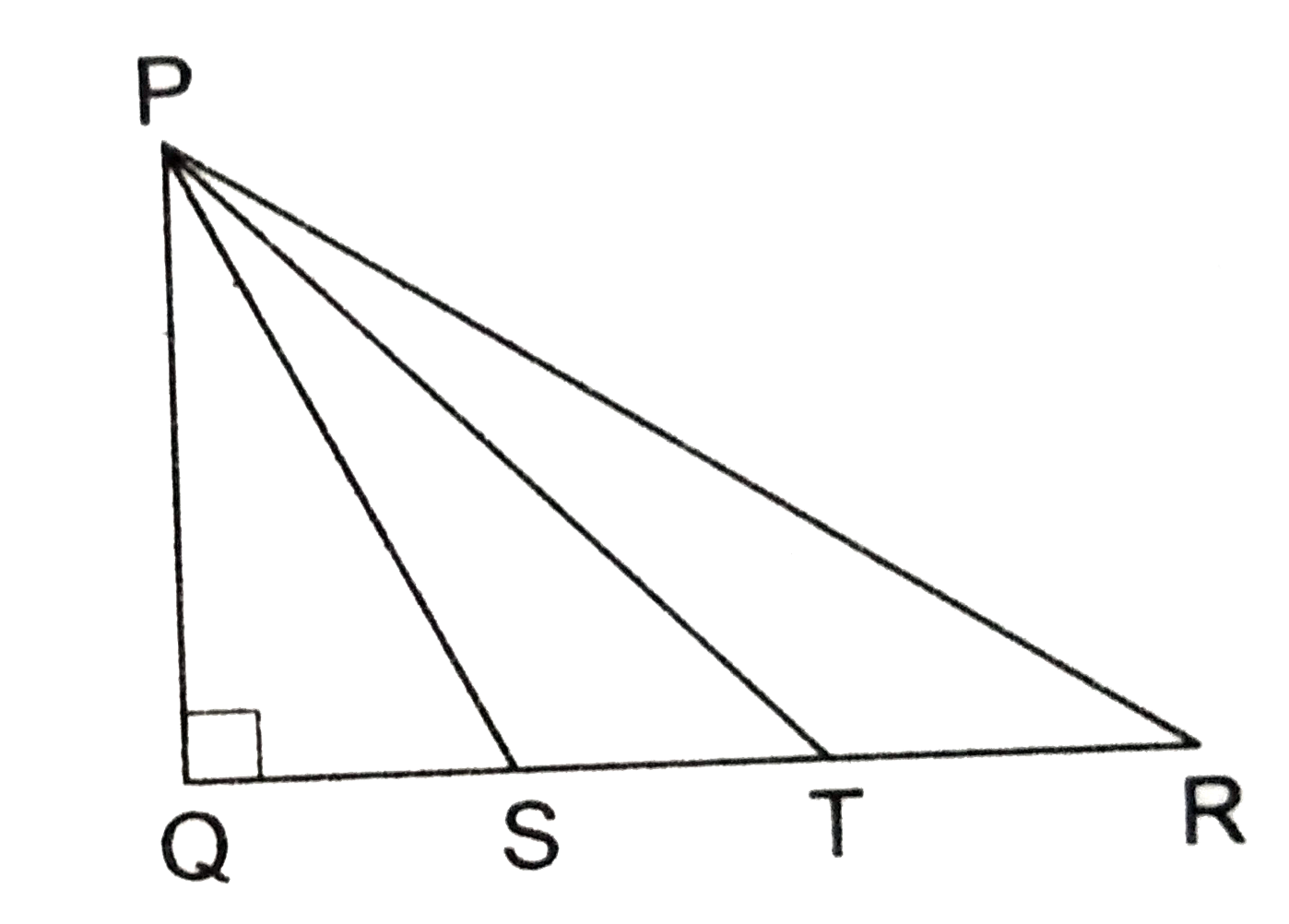
PS2=PQ2+QS2,PT2=PQ2+QT2andPR2=PQ2+QR2
∴3PR2+5PS2−TP2
=3(PQ2+QR2)+5(PQ2+QS2)−8(PQ2−QT2)
=3QR2+5QS2−8QT2 ltbr =3×(3x)2+5(x)2−8×(2x)2[∴QR=3x,QS=xandQT=2x]
=(27x+5x2−x2)=0
Thus, 3PR2+5PS2−8PT2=0
Hence, 8PT2=3PR2=3PR2+5PS2
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE4videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A1videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 1127videos
T-RATIOS OF SOME PARTICULAR ANGLES
- BOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself20videos
TRIGONOMETRIC IDENTITIES
Similar Questions
In Figure, ABC is a right triangle right angled at B and points DandE trisect BC . Prove that 8AE2=3AC2+5AD2.
View SolutionPQR is a triangle right-angled at P and M is a point on QR such that PM⊥QR. Show thatPM2=QM.MR.
View Solution1. In the figure △PQR is an equilateral triangle, the point N is on ray QR such that QR=RN. Prove that PN2=3PR2
View SolutionIn the figure 2.35,ΔPQR is an equilatral triangle. Point S is on seg QR such that QS=13QR. Prove that : 9PS2=7PQ2
View SolutionIn the adjoining figure, PQR, is a right triangle, right angled at Q. X and Y are the points on PQ and QR such that PX:XQ=1:2 and QY:YR=2:1. Prove that 9(PY2+XR2)=13PR2
View SolutionIn an isosceles triangle PQR, PQ =PR and S is any point on side QR. Then prove that: PQ2−PS2=QS×SR.
View SolutionIn the figure ΔPQR is an equilateral triangle. Point S is on seg QR such that
QS=13QR.
Prove that : 9PS2=7PQ2
 View Solution
View SolutionIn the adjoining figure, ΔPQR is an equilateral triangle. Point S is on seg QR. such that QS=13QR. Prove that : 9PS2=7PQ2.
View SolutionIn PQR , S is any point on the side QR . Show that PQ+QR+RP>2PS
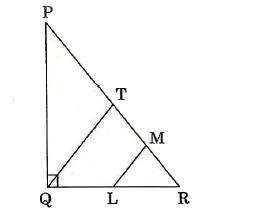
View SolutionIn the figure given below, PQR is a non-isosceles right-angled triangle, right angled at Q. If LM and QT are parallel and QT = PT, then what is ∠RLM equal to ?
 View Solution
View Solution

