Find the area of the triangle formed by the lines joining the vertex of the parabola x2=12yto the ends of its latus rectum.
9 square units
12 square units
14 square units
18 square units
The correct Answer is:D
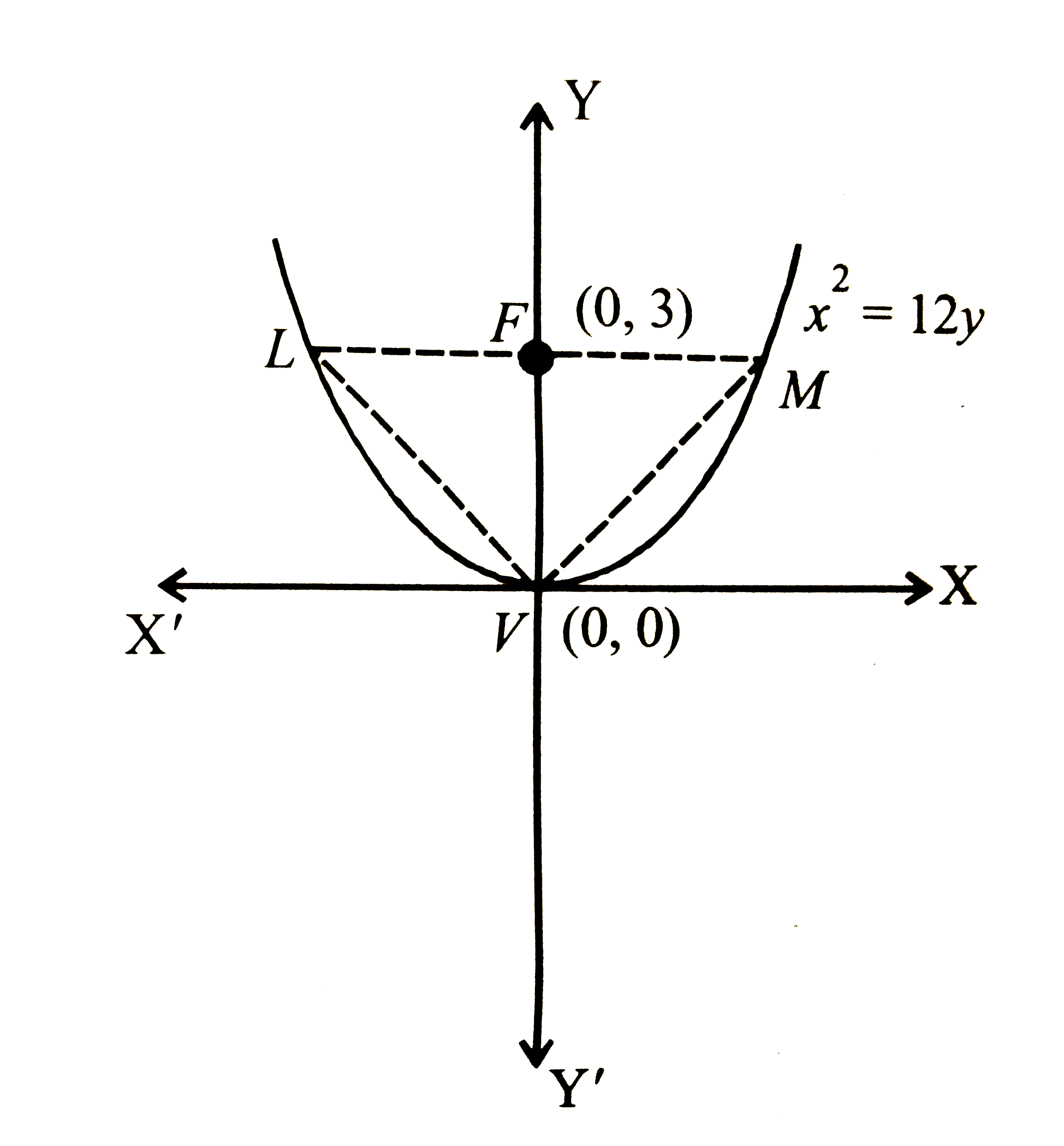
Given parabola is x2=12y which is of the form x2=4ay.
⇒ 4a=12⇒a=3
Now, LM is the latus rectum whose length =4a=4×3=12
So, area of ΔLML=12×LM×VF.
=(12×12×3)sq. unit
= 18 square unit
Topper's Solved these Questions
Similar Questions
Knowledge Check
The area of the triangle formed by the lines joining the focus of the parabola y2=12x to the points on it which have abscissa 12 are
A100 sq. unitsB130 sq. unitsC120 sq. unitsD108 sq. units
Find the area of the triangle formed by the lines joining the vertex of the parabola x2=8y to the ends of its latus rectum.
View SolutionFind the area of the triangle formed by the lines joining the vertex of the parabola x2=−36y to the ends of the latus rectum.
View SolutionFind the area of the triangle formed by the lines joining the vertex of he parabola x2=12y to the ends of its latus-rectum.
View SolutionFind the area of the triangle formed by the lines joining the vertex of the parabola y2=16x to the ends of the latus rectum.
View SolutionFind the area of the triangle formed by the lines joining the focus of the parabola y2=4x to the points on it which have abscissa equal to 16.
View SolutionFind the area of the triangle formed by the vertex and the ends of the latus rectum of the parabola
x2=−36y.
View SolutionFind the equation of a line joining the vertex of parabola y2=8x to its upper end of latus rectum.
View Solution
