In the adjoining figure, PQR, is a right triangle, right angled at Q. X and Y are the points on PQ and QR such that $P X : X Q = 1 : 2$ and $Q Y : Y R = 2 : 1$ . Prove that $9 (P Y^{2} + X R^{2}) = 13 P R^{2}$

Video Solution

Answer

Step by step video & image solution for In the adjoining figure, PQR, is a right triangle, right angled at Q. X and Y are the points on PQ and QR such that PX : XQ = 1:2 and QY: YR = 2:1. Prove that 9(PY^2 + XR^2) = 13PR^2 by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

TRIANGLESBOOK - VK GLOBAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - SELF ASSESSMENT TEST
10 Videos
TRIANGLESBOOK - VK GLOBAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - PROFICIENCY EXERCISE (SHORT ANSWER TYPE QUESTIONS-II)
39 Videos
REAL NUMBERSBOOK - VK GLOBAL PUBLICATIONCHAPTER - REAL NUMBERSEXERCISE - Self -Assessment Test
11 Videos
VALUE-BASED QUESTIONSBOOK - VK GLOBAL PUBLICATIONCHAPTER - VALUE-BASED QUESTIONSEXERCISE - Unit-VII : (Statistics & Probability)
8 Videos

Knowledge Check

In the figure below, PQR is a right-angled triangle, right angled at P. A perpendicular line PS is drawn from P to QR. PR = 5 cm and PQ = 12 cm.

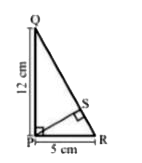
What is RS:SQ?
A $5 : 12$
B $13 : 17$
C $13 : 60$
D $25 : 144$

In given figure PQR is a right angled triangle, right angled at Q and QS $⊥$ PR. If PQ=6 cm and PS=4cm, then find QS, RS and QR.

View Solution
In a right triangle ABC , right angled at C, P and Q are the points of the sides CA and CB respectively , which divide these sides in the ratio $2 : 1$ Prove that
$9 (A Q^{2} + B P^{2}) = 13 A B^{2}$
View Solution
In the given Fig. PQR is a triangle, right angled at Q. If XY || QR, PQ =6 cm, PY= 4 cm and PX : XQ= 1 : 2. Calculate the lengths of PR and QR.
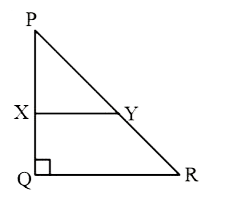
View Solution
PQR is a triangle right-angled at Q. If X and Y are the mid-points of the sides PQ and QR respectively, then which one of the following is not correct?
View Solution
In the adjoining figure, $Δ P Q R$ is an equilateral triangle. Point S is on seg QR. such that $Q S = \frac{1}{3} Q R .$ Prove that : $9 P S^{2} = 7 P Q^{2} .$
View Solution
M and N are the points on sides QR and PQ respectively of a $△ P Q R$ , right angled at Q. prove that $P M^{2} + R N^{2} = P R^{2} + M N^{2}$
View Solution
1. In the figure $△ P Q R$ is an equilateral triangle, the point N is on ray QR such that QR=RN. Prove that $P N^{2} = 3 P R^{2}$
View Solution

VK GLOBAL PUBLICATION-TRIANGLES-PROFICIENCY EXERCISE (LONG ANSWER QUESTIONS)

CD and GH are respectively the bisectors of /A C Band /E G Fsuch tha...
03:52
In the Fig. given below, OB is the perpendicular bisector of the line ...
04:37
In the adjoining figure, PQR, is a right triangle, right angled at Q. ...
03:49
14 In Fig. 6.21, PA, QB Rc and SD are all perpendiculars to a line l, ...
07:34
If a perpendicular is drawn from the vertex containing the right angle...
07:04
In given figure PQR is a right angled triangle, right angled at Q and ...
04:17
In fig.7.84, DEFG is a square and angleBAC = 90°. Prove that: (i)...
05:02
Prove that the area of the semicircle drawn on the hypotenuse of a rig...
04:09
Ii DeltaPQR, PDbotQR such that D lies on QR, if PQ=a,PR=b,QD=c and DR=...
02:48
P and Q are the mid-points of the sides CA and CB respectively of a tr...
02:51
In Fig. 7 .85, DEabs()BC and AD : DB = 5 : 4. Find ("Area"(DeltaDEF))/...
09:32
D and E are points on the sides A B and A C respectively of a A...
03:43
In Fig. 7.86, if D and E trisects BC. Prove that 8AE^(2) = 3AC^(2) + 5...
07:44
In a triangle A B C ,\ A C > A B , D is the mid-point of B C and...
03:08
In an equilateral triangle with side a , prove that Altitude =(a...
02:06

Home
Ask Doubt
Profile

In the adjoining figure, PQR, is a right triangle, right angled at Q. X and Y are the points on PQ and QR such that PX:XQ=1:2 and QY:YR=2:1. Prove that 9(PY2+XR2)=13PR2

Topper's Solved these Questions

Similar Questions

Knowledge Check

In the figure below, PQR is a right-angled triangle, right angled at P. A perpendicular line PS is drawn from P to QR. PR = 5 cm and PQ = 12 cm. What is RS:SQ?

VK GLOBAL PUBLICATION-TRIANGLES-PROFICIENCY EXERCISE (LONG ANSWER QUESTIONS)

In the adjoining figure, PQR, is a right triangle, right angled at Q. X and Y are the points on PQ and QR such that $P X : X Q = 1 : 2$ and $Q Y : Y R = 2 : 1$ . Prove that $9 (P Y^{2} + X R^{2}) = 13 P R^{2}$

In the figure below, PQR is a right-angled triangle, right angled at P. A perpendicular line PS is drawn from P to QR. PR = 5 cm and PQ = 12 cm.

What is RS:SQ?