Find the magnitude and direction of the resultant of two vectors A and B in terms of their magnitudes and angle θ between them .
 Solution in Bengali
Solution in Bengali
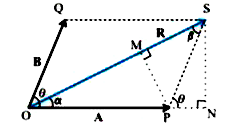
Let OP and OQ represent two vectors A and B making an angle θ (Fig . 4.10) . Then , using the parallelogram method of vector addition , OS represents the resultant vector R:
R = A+B
SN is normal to OP and PM is normal to OS . From the geometry of the figure,
OS2=ON2+SN2
but ON=OP+PN=A+Bcosθ
SN=Bsinθ
OS2=(A+Bcosθ)2+(Bsinθ)2
or , R2=A2+B2+2ABcosθ
R=√A2+B2+2ABcosθ
In ΔOSNSN=OSsinalpha=Rsinalpha,and<br>∈DeltaPSN,SN=PSsintheta=Bsintheta<br>Therefore,R sinalpha=Bsintheta<br>or(R)/(sintheta)=(B)/(sinalpha)<br>similarly<br>PM=Asinalpha=Bsinbeta<br>or(A)/(sinbeta)=(B)/(sinalpha)<br>orComb∈∈gEqs.(4.24b)and(4.24c),we≥t<br>(R)/(sintheta)=(A)/(sinbeta)=(B)/(sinalpha)<br>UsingEq.(424a).<br>or,tanalpha=(SN)/(OP+PN)=(Bsintheta)/(A+Bcostheta)
Equation (4.24a) gives the magnitude of the resultant and Eqs . (4.24e) and (4.24f) its direction. Equantion (4.24a) is known as the Law of cosines and Eq . (4.24d) as the Law of sines.

Topper's Solved these Questions
- MOTION IN A PLANEBOOK - NCERT BANGLISHCHAPTER - MOTION IN A PLANEEXERCISE - EXCERCISES
30 Videos
- MECHANICAL PROPERTIES OF SOLIDS BOOK - NCERT BANGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDS EXERCISE - Exercises
16 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT BANGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ADDITIONAL EXERCISES
5 Videos
Similar Questions
State , for each of the following physical quantities , if it is a scalar or a vector : volume , mass , speed , acceleration , density , number of moles , velocity , angular frequency , displacement , angular velocity .
View SolutionA steet wire of length 4.7 m and cross - sectional area 3.0×10−5m2 stretches by the same amount as a copper wire of length 3.5 m and cross- sectional area of 4.0×10−5m2 under a given load. What is the ratio of the Young's modulus of steel to that of copper ?
View SolutionA boy standing on a stationary lift (open from above) throws a ball upwards with the maximum initial speed he can, equal to 49ms−1. How much time does the ball take to return to his hands ? If the lift starts moving up with a uniform speed of 5ms−1 and the boy again throws the ball up with the maximum speed he can, how long does the ball take to return to his hands ?
View SolutionTwo vectors having equal magnitudes A make an angle θ with eahc other. Find the magnitude and direction of the resultant.
View SolutionFind the magnitude and direction of the resultant of two vectors A and B in the terms of their magnitudes and angle θ between them.

View SolutionFind the magnitude and direction of the resultant of two vectors A and B in the terms of their magnitudes and angle θ between them.

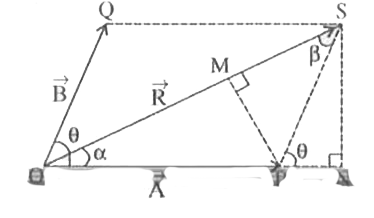
View Solutionचित्र में दिखाये गये दो सदिशों →A और →B के बीच का कोण θ है। इनके परिणामी सदिश का परिमाण तथा दिशा उनके परिमाणों तथा θ के पद मे निकालिए।
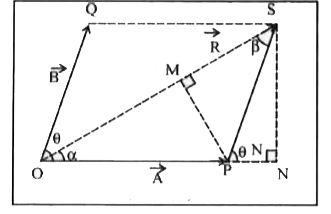
View Solutionचित्र में दिखाए गए दो सदिशों A तथा B के बीच का कोण θ है। इनके परिणामी सदिश का परिमाण तथा दिशा उनके परिमाणों तथा θ के पद में निकालिए।
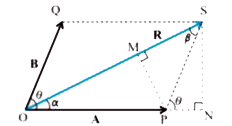
View SolutionFind the magnitude and direction of the resultant of two vectors A and B in terms of their magnitudes and angle theta between them .
View SolutionFind the magnitude and direction of the resultant of two vectors A and B in terms of their magnitudes and angle θ between them .
View SolutionIf magnitude of the resultant of two vectors equal of magnitude, is equal to the magnitude of either of the vectors, what is the angle between them ?
View SolutionFind the magnitude and direction of the resultant of two vectors A and B in the terms of their magnitudes and angle θ between them.

View SolutionTwo vectors having equal magnitudes A make an angle θ with eahc other. Find the magnitude and direction of the resultant.
View SolutionIf magnitude of the resultant of two vectors equal of magnitude, is equal to the magnitude of either of the vectors, what is the angle between them ?
View SolutionFind the magnitude and direction of the resultant of two vectors vecA and vecB in terms of their magnitudes and angle between them.
View Solution
