If tangents PA and PB from a point P to a circle with centre O are inclined to each other at angle of 80∘, then ∠POA is equal to
To solve the problem step by step, we will follow the reasoning presented in the video transcript.
Step 1: Understand the Given Information
We have a circle with center O, and two tangents PA and PB drawn from an external point P. The angle between these tangents, ∠APB, is given as 80 degrees.
Step 2: Identify Important Angles
Since PA is a tangent to the circle at point A, the radius OA is perpendicular to the tangent PA. Therefore, we have:
∠OAP=90∘
Step 3: Analyze the Triangles
In triangles OAP and OBP:
- OA = OB (both are radii of the circle)
- PA = PB (tangents from the same external point are equal)
- OP is common to both triangles.
This means that triangles OAP and OBP are congruent (by the Side-Side-Side postulate).
Step 4: Use the Congruence to Find Angles
Since triangles OAP and OBP are congruent, the angles ∠OPA and ∠OPB are equal:
∠OPA=∠OPB
Step 5: Relate Angles Using the Given Information
From the triangle APB, we know:
∠APB=80∘
Since ∠OPA and ∠OPB are equal, we can express them as:
∠OPA=∠OPB=12∠APB
Thus:
∠OPA=12×80∘=40∘
Step 6: Find Angle POA
Now, in triangle OAP, we can find angle POA using the fact that the sum of angles in a triangle is 180 degrees:
∠OAP+∠OPA+∠POA=180∘
Substituting the known values:
90∘+40∘+∠POA=180∘
This simplifies to:
130∘+∠POA=180∘
Thus:
∠POA=180∘−130∘=50∘
Final Answer
Therefore, the angle ∠POA is:
∠POA=50∘
---
We have a circle with center O, and two tangents PA and PB drawn from an external point P. The angle between these tangents, ∠APB, is given as 80 degrees.
Since PA is a tangent to the circle at point A, the radius OA is perpendicular to the tangent PA. Therefore, we have:
∠OAP=90∘
In triangles OAP and OBP:
- OA = OB (both are radii of the circle)
- PA = PB (tangents from the same external point are equal)
- OP is common to both triangles.
Since triangles OAP and OBP are congruent, the angles ∠OPA and ∠OPB are equal:
∠OPA=∠OPB
From the triangle APB, we know:
∠APB=80∘
Since ∠OPA and ∠OPB are equal, we can express them as:
∠OPA=∠OPB=12∠APB
Thus:
∠OPA=12×80∘=40∘
Now, in triangle OAP, we can find angle POA using the fact that the sum of angles in a triangle is 180 degrees:
∠OAP+∠OPA+∠POA=180∘
Substituting the known values:
90∘+40∘+∠POA=180∘
This simplifies to:
130∘+∠POA=180∘
Thus:
∠POA=180∘−130∘=50∘
Therefore, the angle ∠POA is:
∠POA=50∘
Topper's Solved these Questions
- CIRCLESBOOK - NCERT ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.1
2 Videos
- CIRCLESBOOK - NCERT ENGLISHCHAPTER - CIRCLESEXERCISE - SOLVED EXAMPLES
3 Videos
- ARITHMETIC PROGRESSIONSBOOK - NCERT ENGLISHCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - All Questions
65 Videos
- CONSTRUCTIONSBOOK - NCERT ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - EXERCISE 11.2
7 Videos
Similar Questions
If tangents PA and PB from a point P to a circle with centre O are inclined to each other at an angle of 80o then ∠POA is equal to :
View SolutionDraw a pair of tangents to a circle of radius 5cm which are inclined to each other at an angle of 60o .
View SolutionTwo tangents TP and TQ are drawn from an external point T to a circle with centre O as shown in figure. If they are inclined to each other at an angle of 100∘, then what is the value of ∠POQ?
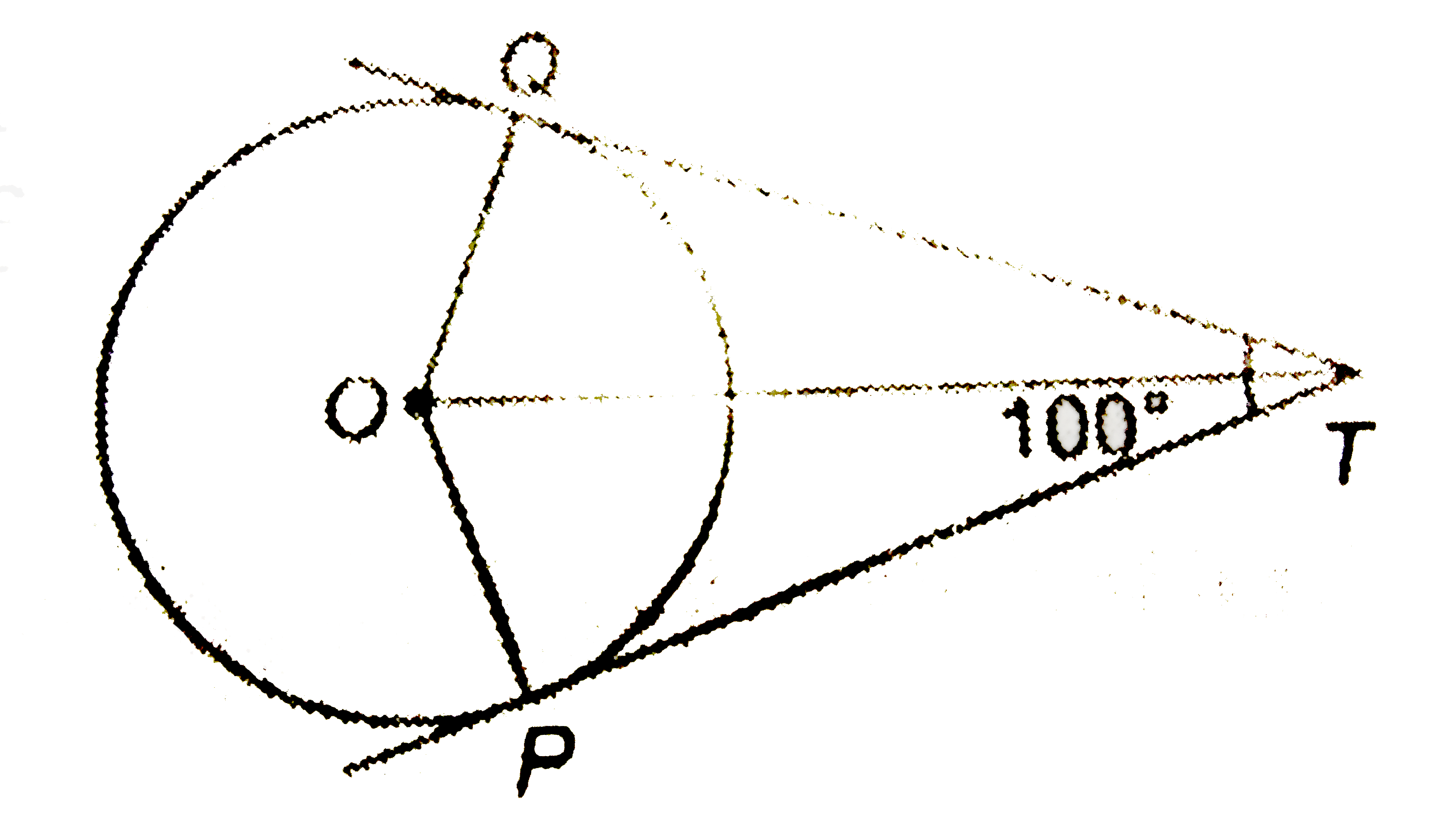
View SolutionDraw a pair of tangents to a circle of radius 4.5 cm, which are inclined to each other at an angle of 45o
View SolutionTwo tangents TP and TQ are drawn from an external point T to a circle with centre O as shown in Fig. 10.73. If they are inclined to each other at an angle of 100o , then what is the value of ∠POQ ?
View SolutionPQ is a tangent drawn from a point P to a circle with centre O and QOR is a diameter of the circle such that ∠POR=120o , then ∠OPQ is 60o (b) 45o (c) 30o (d) 90o
View SolutionFrom a point P , two tangents PA and PB are drawn to a circle with centre O . If OP= diameter of the circle, show that △APB is equilateral.
View SolutionTwo tangents are draw from a point P, 40 units from the centre of the circle and inclined to each other at 60∘. What is the area , to the nearet integer, of the region AXBP ?
View SolutionIn figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to

View SolutionPQ is a tangent to a circle with centre O at the point P . If △OPQ is an isosceles triangle, then ∠OQP is equal to :
View Solution
