In Fig. 7.48, sides ABandAC of ΔABC are extended to points PandQ respectively. Also, ∠PBC <∠QCB. Show that AC > AB.
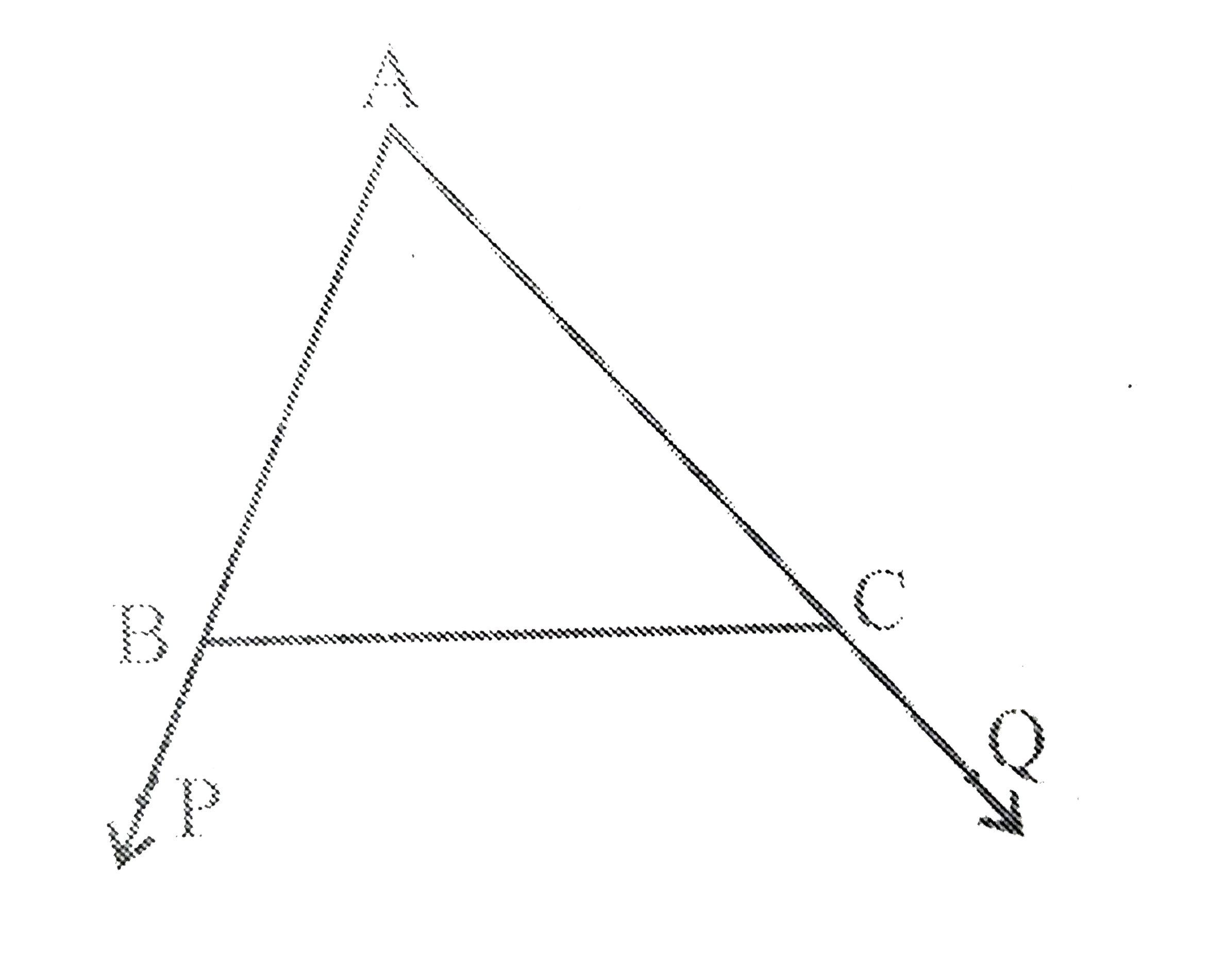
(##IX07_E0402_s01##)
In the given figure,
∠ABC+∠PBC=180∘ [Linear pair of angles]
Also, ∠ABC=180∘−∠PBC ... (1)
∠ACB+∠QCB=180∘
[Linear pair]
∠ACB=180∘−∠QCB ... (2)
As ∠PBC<∠QCB (given),
180∘−∠PBC>180∘−∠QCB
∠ABC>∠ACB [From Equations (1) and (2)]
Thus, AC>AB (the Side opposite to the larger angle is larger).
Hence proved, AC>AB.
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.1
8 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Solved Examples
9 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.2
8 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.9
3 Videos
Similar Questions
The sides AB and AC of a ABC are produced to P and Q respectively. If the bisectors of ∠PBC AND ∠QCB intersect at O, then ∠boc=900−12∠A GIVEN : A ABC in which sides AB and AC are produced to P and Q respectively. The bisectors of ∠PBC and ∠QCB intersect at O.
View SolutionIn ABC and PQR Figure, AB=PQ,BC=QR and CB and RQ are extended to X and Y respectively and ∠ABX =∠PQY. Prove that ABC≅PQR. Figure
View SolutionIf a line intersects sides AB and AC of a ΔABCat D and E respectively and is parallel to BC, prove that ADAB=AEAC
View SolutionIn ΔABC and ΔPQR, AB=PQ, BC=QR and CB and RQ are extended to X and Y respectively and ∠ABX=∠PQY. Prove that ABC≅ PQR.
View SolutionIn Fig. 4.34, A, B and C are points on OP, OQ and OR respectively such that ABPQ and BCQR . Show that ACPR . (FIGURE)
View SolutionIn Fig. 10.20, the sides AB, BC and CA of triangle ABC touch a circle with centre O and radius r at P, Q and R respectively. (FIGURE) Prove that: 1) AB + CQ = AC + BQ
View SolutionTriangles ABC and PQR are both isosceles with AB=AC and PQ=PR respectively. If also, AB=PQ and BC=QR, are the two triangles congruent? Which condition do you use? If△B= 500, what is the measure of∠R?
View SolutionIn Fig.100, AB∣∣CD and EF is a transversal intersecting AB and CD at P and Q respectively. The measure of ∠DQP is (a) 65 (b) 25 (c) 115 (d) 105
View SolutionIn a triangle ABC , let P and Q be points on AB and AC respectively such that PQ∣∣BC . Prove that the median AD bisects PQ.
View SolutionIn Figure altitudes AD and CE of A B C intersect each other at the point P. Show that:(i) ΔAEP~ΔCDP (ii) ΔABD~ΔCBE (iii) ΔAEP~ΔADB(iv) ΔPDC~ΔBEC
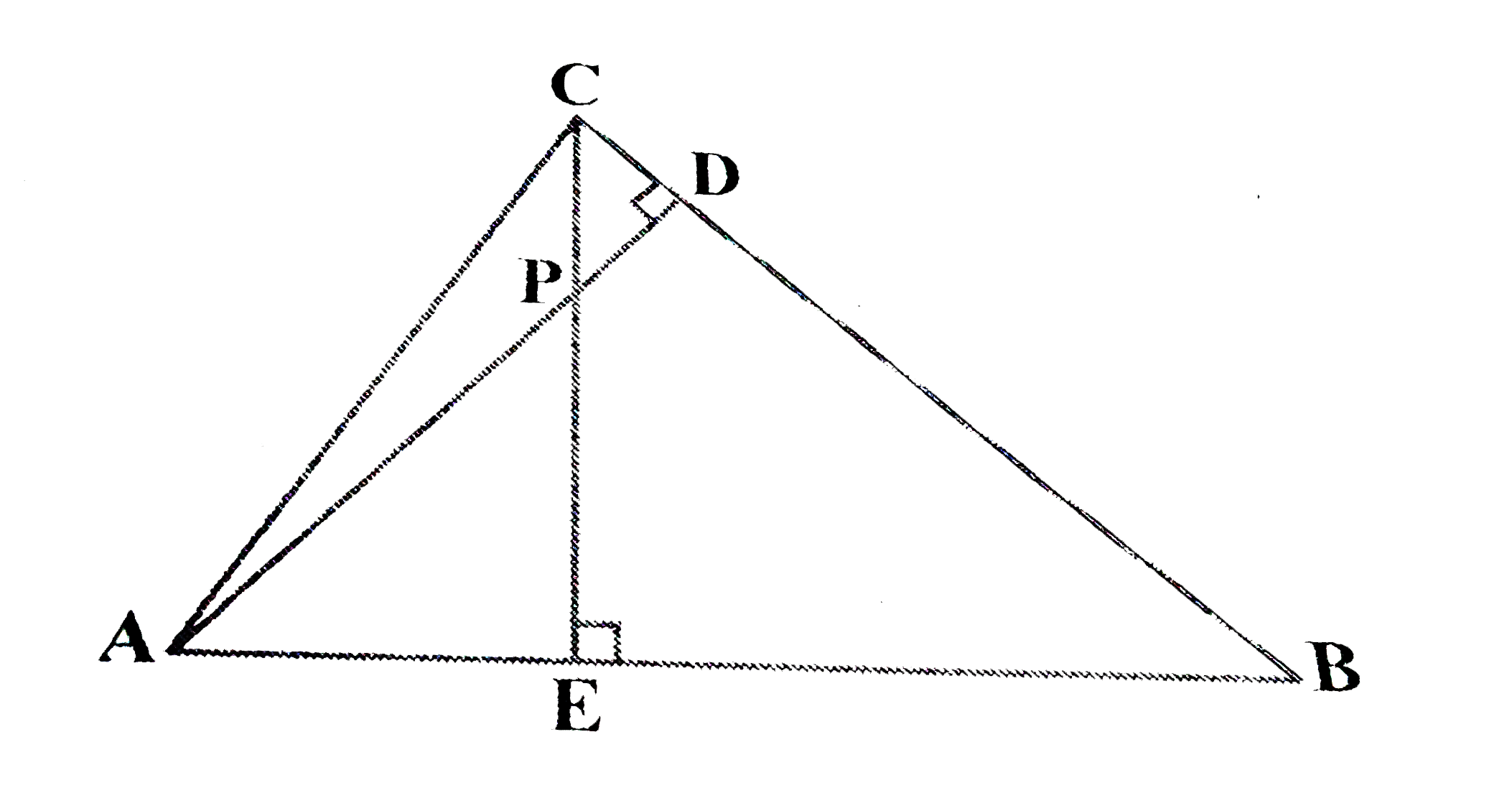
View Solution
