In Fig. 9.32, ABCD is a parallelogram and BC is produced to a point Q such that $A D = C Q$ . If AQ intersect DC at P, show that $a r (B P C) = a r (D P Q) \dot{}$
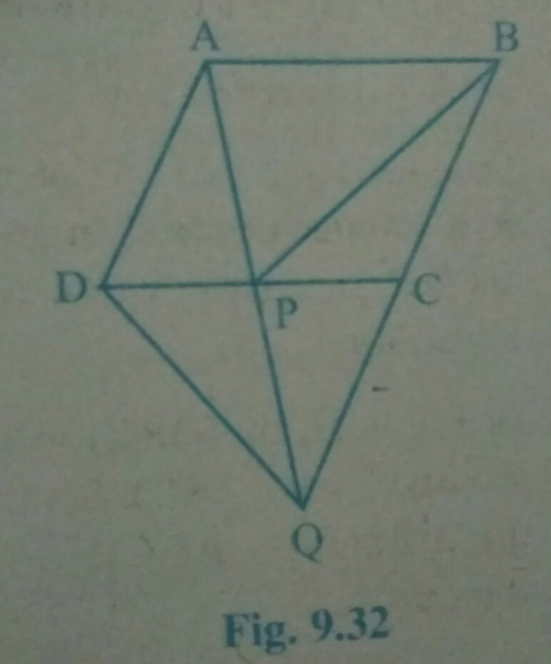
$In Fig. 9.32, ABCD is a parallelogram and BC is produced to a point Q such thatA D\ =\ C Q. If AQ intersect DC at P, show that a r\ (B P C)\ =\ a r\ (D P Q)dot$

Video Solution

Text Solution

Verified by Experts

It is given that $A B C D$ is a parallelogram.
$A D ∣ ∣ B C$ and $A B ∣ ∣ D C$ (Opposite sides of a parallelogram are parallel to each other).
Now, join the points A and C. Consider $△ A P C$ and $△ B P C$
$△ A P C$ and $△ B P C$ are lying on the same base $P C$ and between the same parallels $P C and A B .$
According to Theorem 9.2:
Two triangles on the same base (or equal bases) and between the same parallels are equal in area.
Therefore, $A r e a (△ A P C) = A r e a (△ B P C$ ) ...(1)
In quadrilateral $A C Q D$ , it is given that $A D = C Q$
Since $A B C D$ is a parallelogram,
$A D ∣ ∣ B C$ (Opposite sides of a parallelogram are parallel)
$C Q$ is a line segment that is obtained when line segment $B C$ is produced.
$A D ∣ ∣ C Q$
We have, $A D = C Q$ and $A D ∣ ∣ C Q$
Hence, $A C Q D$ is a parallelogram.
Consider $△ D C Q$ and $△ A C Q$
These are on the same base $C Q$ and between the same parallels $C Q$ and $A D .$
Therefore, $A r e a (△ D C Q) = A r e a (△ A C Q)$
$A r e a (△ D C Q) - A r e a (△ P Q C) = A r e a (△ A C Q) - A r e a (△ P Q C)$
[Subtracting $A r e a$ ( $△ P Q C$ ) on both sides.]
$A r e a (△ D P Q) = A r e a (△ A P C)$ ...(2)
From equations (1) and (2), we obtain
$A r e a (Δ B P C) = A r e a (Δ D P Q),$ proved.

|

Updated on:21/07/2023

Class 9 MATHS AREA OF PARALLELOGRAMS AND TRIANGLES

Topper's Solved these Questions

Similar Questions

NCERT ENGLISH-AREAS OF PARALLELOGRAMS AND TRIANGLES-EXERCISE 9.4

Home
Ask Doubt
Profile