Question

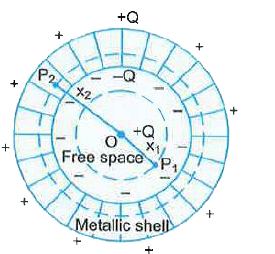
A small metal sphere carrying charge +Q is located at the centre of a sphereical cavity inside a large uncharged metallic spherical shell. Use Gauss' law to find the expression for the electric field
At a point $P_1$ situated inside the cavity at a distance $x_1$ from centre.
(ii) At a point $P_2$ situated in the metalic spherical shell at a distance $x_2$ from the centre.

Explanation

Let charge +Q is located at the centre O of a spherical cavity inside a large uncharged metallic spherical shell as shown in Then a charge -Q is induced on inner surface of shell and a charge +Q is induced on the outer surface of the shell.
Let `P_1 ` be a point inside the cavity at a distance `x_1 ` from the centre O. To find electric field ` E_1 ` at point `P_1` let us consider a spherical surface of radius `x_1` as the Gaussian surface. Then ,electric flus.
` phi _in =int oversetto E . oversetto (dS) =E_1 s_1 = E_1 (4 pi x_1^(2)) `
` " " = (1)/( in_0) ("charge enclosed") =(Q)/(in_0) `
` rArr " "E_1 =(Q)/( 4 pi in _0 x_1^(2))`
Let `P_2` be a point situated in the metallic shell at a distance `x_2` from the centre. To find electric field `E_2` at point ` P_2` let us again consider a spherical surface of radius `x_2` as the Gaussian surface. Then Electric flux
` " " phi_in =E_2s_2 =E_2 ( 4 pi x_2^(2)) =(1)/( in _0) ("charge enclosed") = (1)/( in_0) (Q-Q)= 0 `
`rArr " "E_2 =0`