If AB =12cm, BC=16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is
6 cm
8 cm
10 cm
12 cm
The correct Answer is:C
To find the radius of the circle passing through points A, B, and C, we can follow these steps:
Step 1: Understand the Triangle
Given that AB = 12 cm, BC = 16 cm, and AB is perpendicular to BC, we can identify triangle ABC as a right triangle with the right angle at B.
Step 2: Apply Pythagorean Theorem
In a right triangle, the Pythagorean theorem states that:
AC2=AB2+BC2
Substituting the given values:
AC2=122+162
Step 3: Calculate the Squares
Calculate 122 and 162:
- 122=144
- 162=256
Step 4: Sum the Squares
Now, add these two results:
AC2=144+256=400
Step 5: Find AC
To find AC, take the square root of 400:
AC=√400=20cm
Step 6: Determine the Diameter and Radius
Since AC is the diameter of the circle, we can find the radius by dividing the diameter by 2:
Radius=AC2=202=10cm
Final Answer
Thus, the radius of the circle passing through points A, B, and C is 10 cm.
---
Given that AB = 12 cm, BC = 16 cm, and AB is perpendicular to BC, we can identify triangle ABC as a right triangle with the right angle at B.
In a right triangle, the Pythagorean theorem states that:
AC2=AB2+BC2
Substituting the given values:
AC2=122+162
Calculate 122 and 162:
- 122=144
- 162=256
Now, add these two results:
AC2=144+256=400
To find AC, take the square root of 400:
AC=√400=20cm
Since AC is the diameter of the circle, we can find the radius by dividing the diameter by 2:
Radius=AC2=202=10cm
Thus, the radius of the circle passing through points A, B, and C is 10 cm.
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.2
10 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.3
20 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.4 Long Answer Type Questions
10 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
Let ABC be a right triangle in which AB = 6 cm, BC = 8 cm and ∠B=90∘. BD is the= perpendicular from B on AC. The circle through B, C, D is drawn. Construct the tangents from A to this circle.
View SolutionLet ABC is a right triangle in which AB = 6 cm, BC = 8 cm, ∠B=90∘.BD is the perpendicular from B on AC. The circle through B,C and D is drawn. Construct the tangents from A to this circle.
View SolutionDraw a circle with centre at a point O and radius 5.6 cm. Draw any chord AB. Draw a perpendicular bisector of AB. Does the bisector pass through the centre O ?
View SolutionConstruct a triangle ABC, in which AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm. Draw perpendicular bisector of BC which meets AC at point D. Prove that D is equidistant from B and C.
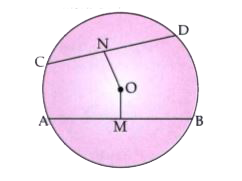
View SolutionIn the figure given below, O is the centre of the circle. AB and CD are two chords of the circle. OM is perpendicular to AB and ON is perpendicular to CD. AB = 24 cm, OM = 5 cm, ON = 12 cm. Find the :
(i) radius of the circle
(ii) length of chord CD
View SolutionConstruct a triangle ABC in which AC=5cm, BC= 7 cm and AB= 6cm.
Construct a circle which touches BC at C and passes through D, where D is the midpoint of AB.
View SolutionConstruct a right-angled triangle right-angled at A, hypotenuse BC = 8 cm and AB = 5 cm. Construct the perpendicular bisectors of AB and AC. Mark the point of intersection of the perpendicular bisectors as P. Measure PA, PB and PC.
View SolutionConstruct ΔABC with AB = 8 cm, BC = 6 cm, and CA = 7 cm. Find the midpoint D on AB. Through the point D, draw DE parallel to BC, meeting AC at E. Measure DE. Find DE : BC.
View SolutionA circle passes through a fixed point A and cuts two perpendicular straight lines through A in B and C. If the straight line BC passes through a fixed-point (x1,y1), the locus of the centre of the circle, is
View SolutionTwo variable chords AB and BC of a circle x2+y2=a2 are such that AB=BC=a. M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C is
View Solution
