A tangent at a point on the circle x2+y2=a2 intersects a concentric circle C at two points PandQ . The tangents to the circle X at PandQ meet at a point on the circle x2+y2=b2. Then the equation of the circle is x2+y2=ab x2+y2=(a−b)2 x2+y2=(a+b)2 x2+y2=a2+b2
x2+y2=ab
x2+y2=(a−b)2
x2+y2=(a+b)2
x2+y2=a2=b2
The correct Answer is:1
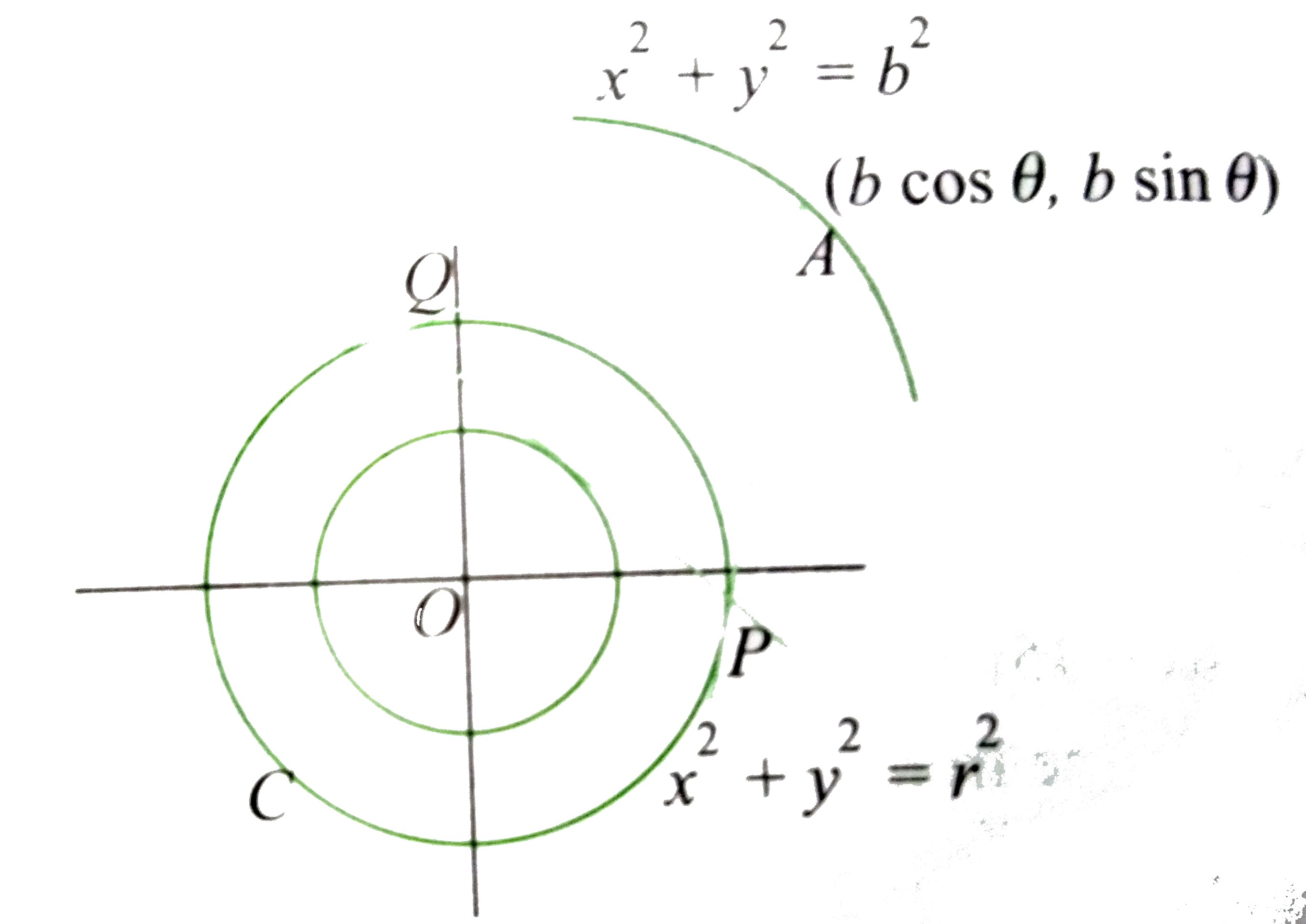
Chord of contact of point A w.r.t x2+y2=r2 is
xbcosθ+ybsinθ=2 (1)
This must be a tangent to the circle x2+y2=a2. Therefore,
[r2√b2cos2θ+b2sin2θ]=a or r2=ab
Hence, the equation of circle is x2+y2=ab
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Multiple Correct Anser Type29videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Linked Comprehension Type (For Problem 1-3)3videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.201videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix4videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
A tangent is drawn to the circle 2(x2+y2)−3x+4y=0 and it touches the circle at point A. If the tangent passes through the point P(2, 1),then PA=
View SolutionIf the tangent at the point on the circle x2+y2+6x+6y=2 meets the straight ine 5x−2y+6=0 at a point Q on the y- axis then the length of PQ is
View SolutionThe line 2x - y + 1 = 0 is tangent to the circle at the point (2,5) and the centre of the circles lies on x-2y = 4. The radius of the circle is :
View SolutionAngle between tangents drawn from any point on the circle x2+y2=(a+b)2 , to the ellipse x2a+y2b=(a+b) is-
View SolutionIf tangent at (1, 2) to the circle C1:x2+y2=5 intersects the circle C2:x2+y2=9 at A and B and tangents at A and B to the second circle meet at point C, then the co- ordinates of C are given by
View SolutionA tangent to the circle x2+y2=1 through the point (0, 5) cuts the circle x2+y2=4 at P and Q. If the tangents to the circle x2+y2=4 at P and Q meet at R, then the coordinates of R are
View SolutionIf the line ax+by=2 is a normal to the circle x2+y2−4x−4y=0 and a tangent to the circle x2+y2=1 , then
View SolutionThe angle between the tangents drawn from a point on the director circle x2+y2=50 to the circle x2+y2=25, is
View SolutionThe area of the triangle formed by the tangent at the point (a, b) to the circle x2+y2=r2 and the coordinate axes, is
View SolutionThe length of the tangent from a point on the circle x2+y2+4x−6y−12=0 to the circle x2+y2+4x−6y+4=0 is
View Solution

