There are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks:, 0, 1, 2, 3, 4, 5 Frequency , , , , , , . Determine mean and standard deviation of the marks.
To solve the problem, we need to determine the mean and standard deviation of the marks obtained by the students based on the given frequency distribution.
Step 1: Set up the frequency distribution table
The marks and their corresponding frequencies are given as follows:
- Marks: 0, 1, 2, 3, 4, 5
- Frequencies: x−2, x, x2, (x+1)2, 2x, x+1
We know that the total number of students is 60. Therefore, we can write the equation:
(x−2)+x+x2+(x+1)2+2x+(x+1)=60
Step 2: Simplify the equation
Expanding the equation:
1. (x+1)2=x2+2x+1
Substituting this back into the equation:
(x−2)+x+x2+(x2+2x+1)+2x+(x+1)=60
Combining like terms:
x−2+x+x2+x2+2x+1+2x+x+1=60
This simplifies to:
2x2+7x=60
Step 3: Rearranging to form a quadratic equation
Rearranging gives us:
2x2+7x−60=0
Step 4: Factor the quadratic equation
We can factor the quadratic:
(2x+15)(x−4)=0
Setting each factor to zero gives:
1. 2x+15=0⇒x=−152 (not valid since frequency cannot be negative)
2. x−4=0⇒x=4
Step 5: Calculate the frequencies
Substituting x=4 into the frequency expressions:
- x−2=4−2=2
- x=4
- x2=42=16
- (x+1)2=(4+1)2=25
- 2x=2⋅4=8
- x+1=4+1=5
So, the frequencies are:
- 0: 2
- 1: 4
- 2: 16
- 3: 25
- 4: 8
- 5: 5
Step 6: Construct the frequency table
| Marks (x) | Frequency (f) |
|-----------|---------------|
| 0 | 2 |
| 1 | 4 |
| 2 | 16 |
| 3 | 25 |
| 4 | 8 |
| 5 | 5 |
| Total | 60 |
Step 7: Calculate the mean
To find the mean, we use the formula:
Mean=Σ(f⋅x)Σf
Calculating f⋅x:
- 0⋅2=0
- 1⋅4=4
- 2⋅16=32
- 3⋅25=75
- 4⋅8=32
- 5⋅5=25
Now, summing these products:
0+4+32+75+32+25=168
Thus, the mean is:
Mean=16860=2.8
Step 8: Calculate the standard deviation
To find the standard deviation, we use the formula:
σ=√Σ(f⋅(x−Mean)2)Σf
Calculating (x−Mean)2:
- For x=0: (0−2.8)2=7.84
- For x=1: (1−2.8)2=3.24
- For x=2: (2−2.8)2=0.64
- For x=3: (3−2.8)2=0.04
- For x=4: (4−2.8)2=1.44
- For x=5: (5−2.8)2=4.84
Now, calculating f⋅(x−Mean)2:
- 2⋅7.84=15.68
- 4⋅3.24=12.96
- 16⋅0.64=10.24
- 25⋅0.04=1.00
- 8⋅1.44=11.52
- 5⋅4.84=24.20
Summing these products:
15.68+12.96+10.24+1.00+11.52+24.20=75.60
Now, substituting into the standard deviation formula:
σ=√75.6060=√1.26≈1.12
Final Results
- Mean: 2.8
- Standard Deviation: 1.12
- Frequencies: x−2, x, x2, (x+1)2, 2x, x+1
2. x−4=0⇒x=4
- x=4
- x2=42=16
- (x+1)2=(4+1)2=25
- 2x=2⋅4=8
- x+1=4+1=5
- 1: 4
- 2: 16
- 3: 25
- 4: 8
- 5: 5
|-----------|---------------|
| 0 | 2 |
| 1 | 4 |
| 2 | 16 |
| 3 | 25 |
| 4 | 8 |
| 5 | 5 |
| Total | 60 |
- 1⋅4=4
- 2⋅16=32
- 3⋅25=75
- 4⋅8=32
- 5⋅5=25
- For x=1: (1−2.8)2=3.24
- For x=2: (2−2.8)2=0.64
- For x=3: (3−2.8)2=0.04
- For x=4: (4−2.8)2=1.44
- For x=5: (5−2.8)2=4.84
- 4⋅3.24=12.96
- 16⋅0.64=10.24
- 25⋅0.04=1.00
- 8⋅1.44=11.52
- 5⋅4.84=24.20
- Standard Deviation: 1.12
Topper's Solved these Questions
Similar Questions
There are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks: 0 1 2 3 4 5 Frequency x−2 , x , x2 , (x+1)2 , 2x , x+1 Determine the mean and standard deviation.
View SolutionThere are 60 students in a class. The followng is the frequency distribution of marks obtained by the students in a test.
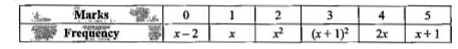
where x is positive integer. Determine the mean of the marks.
View SolutionIf for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
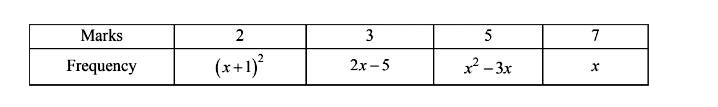
Then the mean of the marks is:
View SolutionFind the mode of the following frequency distribution of marks obtained by 50 students. Marks obtained 0−10 10−20 20−30 30−40 40−50 No. of students 5 12 20 10 3
View SolutionCalculate the arithmetic mean, correct to one decimal place, for the following frequency distribution of marks obtained in a Geometry test.

View SolutionFind the mean from the following frequency distribution of marks at a test in statistics: Marks (x) : 5 10 15 20 25 30 35 40 45 50 No. of students (f) : 15 50 80 76 72 45 39 9 8 6
View SolutionFind the median for the following frequency distribution :
Marks obtained(xi)293234274237Number of students(fi)25101284
View SolutionFollowing is the distribution fo marks obtained by 50 students in a test.
Marks more than01020304050No. of Students50464020103
If 60% of the students pass the test, find the minimum marks obtained by a pass students.
View SolutionIf the mean of the distribution is 2.6, then the value of y is
Variate x12345Frequency f of x45y12
View SolutionThe frequency distribution of marks obtained by 28 students in a test carrying 40 marks is given below:
Marks:0−1010−2020−3030−40Number of students:6xy6
If the mean of the above data is 20, then the difference between x and y is
View Solution
