A guy wire attached to a vertical pole of height 18m is 24m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
To solve the problem, we will use the Pythagorean theorem, which relates the lengths of the sides of a right triangle. Here's the step-by-step solution:
Step 1: Draw the Diagram
We start by visualizing the scenario. We have a vertical pole (AB) of height 18 meters, and a guy wire (AC) of length 24 meters attached to the top of the pole. The stake (C) is driven into the ground at some distance (BC) from the base of the pole (B).
Step 2: Identify the Right Triangle
In this situation, we can form a right triangle ABC where:
- AB = height of the pole = 18 m
- AC = length of the wire = 24 m
- BC = distance from the base of the pole to the stake, which we will denote as x.
Step 3: Apply the Pythagorean Theorem
According to the Pythagorean theorem:
AB2+BC2=AC2
Substituting the known values:
182+x2=242
Step 4: Calculate the Squares
Now, we calculate the squares:
- 182=324
- 242=576
So, we can rewrite the equation:
324+x2=576
Step 5: Rearrange the Equation
To find x2, we rearrange the equation:
x2=576−324
Step 6: Perform the Subtraction
Now, we perform the subtraction:
x2=252
Step 7: Take the Square Root
To find x, we take the square root of both sides:
x=√252
Step 8: Simplify the Square Root
We can simplify √252:
√252=√36×7=√36×√7=6√7
Final Answer
Thus, the distance from the base of the pole to where the stake should be driven is:
x=6√7 meters
---
We start by visualizing the scenario. We have a vertical pole (AB) of height 18 meters, and a guy wire (AC) of length 24 meters attached to the top of the pole. The stake (C) is driven into the ground at some distance (BC) from the base of the pole (B).
In this situation, we can form a right triangle ABC where:
- AB = height of the pole = 18 m
- AC = length of the wire = 24 m
- BC = distance from the base of the pole to the stake, which we will denote as x.
According to the Pythagorean theorem:
AB2+BC2=AC2
Substituting the known values:
182+x2=242
Now, we calculate the squares:
- 182=324
- 242=576
324+x2=576
To find x2, we rearrange the equation:
x2=576−324
Now, we perform the subtraction:
x2=252
To find x, we take the square root of both sides:
x=√252
We can simplify √252:
√252=√36×7=√36×√7=6√7
Thus, the distance from the base of the pole to where the stake should be driven is:
x=6√7 meters
Topper's Solved these Questions
Similar Questions
A guy attached wire to a vertical pole of height 18m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
View SolutionA flag pole 18 m high casts a shadow 9.6 m long. Find the distance of the top of the pole from the far end of the shadow.
View Solution
Knowledge Check
A metal wire of length L1 and area of cross section A is attached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
A[(Y1Y2)A][2(Y1L2+Y2L1)]B[(Y1Y2)A](L1L2)2C[(Y1Y2)A](Y1L2+Y2L1)D[(Y1Y2)1/2A](L2+L1)1/2A metal wire of length L1 and area of cross section A is ttached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
AY1Y2A2(Y1L2+Y2L1)BY1Y2A(L1L2)1/2CY1Y2A(Y1L2+Y2L1)D(Y1Y2)1/2A(L1L2)1/2
A metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
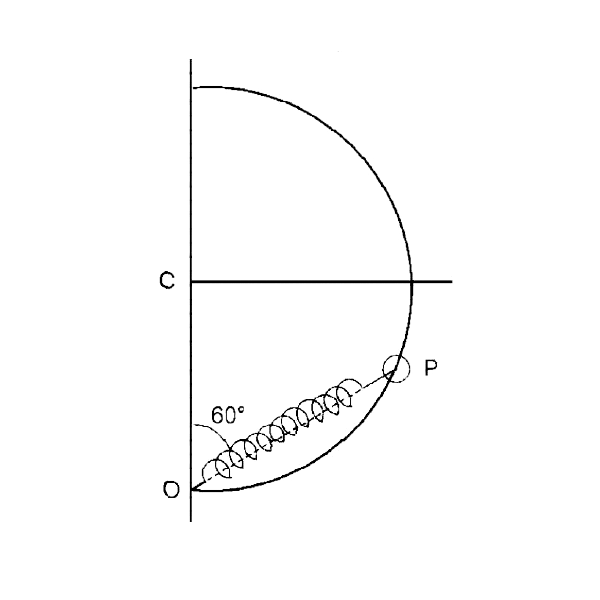
View SolutionA smooth semicircular wire-track of radius R is fixed in a vertical plane. One end of a massless spring of natural length 3R/4 is attached to the lowest point O of the wire-track. A small ring of mass m, which can slide on the track, is attached to the other end of the spring. The ring is held staionary at point P such that the spring makes an angle of 60∘ with the vertical. The spring constant K=mg/R. Consider the instant when the ring is released, and (i) draw the free body diagram of the ring, (ii) determine the tangential acceleration of the ring and the normal reaction.
View SolutionA transverse sinusoidal wave is generated at one end of a long horizontal string by a bar that moves with an amplitude of 1.12 cm . The motion of the bar is continuous and is repeated regularly 120 times per second. The string has linear density of 117g/m. The other end of the string is attached to a mass 4.68 kg. The string passes over a smooth pulley and the mass attached to the other end of the string hangs freely under gravity.
The maximum magnitude of the transverse speed is
View SolutionA flute which we treat as a pipe open at both ends is 60cm long. (a) What is the fundamental frequency when all the holes are covered? (b) How far from the mouthpieces should a hole be uncovered for the fundamental frequency to be 330HZ ? Take speed of sound in air as 340m/s.
View SolutionA rod of length 6m has a mass of 12kg. It is hined at one end of the rod at a distance of 3m below the water surface.
a.What must be the weight of a block that is attached to the other end of the rod so that 5m of the rod's length is under water?
b. Find the magnitude and direction of the force exerted by the hinge on the rod. The specific gravity of the material of rod is 0.5.
View SolutionTwo vertical poles of height 10 m and 40 m stand apart on a horizontal plane. The height (in meters) of the point of intersection of the line joining the top of each pole to the foot of the other, from this horizontal plane is
View Solution
