AB is a chord of length 16cm of a circle of radius 10cm. The tangents at A and B intersect at a point P . Find the length of PA .
To find the length of PA where A and B are points on a circle with a chord AB of length 16 cm and a radius of 10 cm, we can follow these steps:
Step 1: Understand the Geometry
We have a circle with center O and radius OA=OB=10 cm. The chord AB is 16 cm long. The tangents at points A and B intersect at point P.
Step 2: Find the Length of AQ
Since AB is a chord, we can find the lengths AQ and BQ where Q is the foot of the perpendicular from O to AB. Since AB=16 cm, we have:
AQ=AB2=162=8 cm
Step 3: Use the Right Triangle OAQ
In triangle OAQ, we can apply the Pythagorean theorem:
OA2=OQ2+AQ2
Substituting the known values:
102=OQ2+82
100=OQ2+64
OQ2=100−64=36
OQ=√36=6 cm
Step 4: Analyze Triangle PAQ
Now, we consider triangle PAQ where PQ is the length we want to find. Again, applying the Pythagorean theorem:
PA2=PQ2+AQ2
Substituting the known values:
PA2=PQ2+82
PA2=PQ2+64(Equation 1)
Step 5: Analyze Triangle PAO
In triangle PAO, we have:
PO2=PA2+OA2
Since PO=OQ (because both are perpendicular to AB):
OQ2=PA2+102
Substituting the known values:
62=PA2+102
36=PA2+100
PA2=36−100=−64(This is incorrect; we should have used the correct relation)
Step 6: Correctly Relate PO and PA
We actually need to relate PO and PA correctly:
PA2=PO2+OA2
Substituting PO=OQ=6 and OA=10:
PA2=62+102
PA2=36+100=136
Step 7: Solve for PA
Now we can find PA:
PA=√136=2√34 cm
Final Answer
Thus, the length of PA is 403 cm.
We have a circle with center O and radius OA=OB=10 cm. The chord AB is 16 cm long. The tangents at points A and B intersect at point P.
Since AB is a chord, we can find the lengths AQ and BQ where Q is the foot of the perpendicular from O to AB. Since AB=16 cm, we have:
AQ=AB2=162=8 cm
In triangle OAQ, we can apply the Pythagorean theorem:
OA2=OQ2+AQ2
Substituting the known values:
102=OQ2+82
100=OQ2+64
OQ2=100−64=36
OQ=√36=6 cm
Now, we consider triangle PAQ where PQ is the length we want to find. Again, applying the Pythagorean theorem:
PA2=PQ2+AQ2
Substituting the known values:
PA2=PQ2+82
PA2=PQ2+64(Equation 1)
In triangle PAO, we have:
PO2=PA2+OA2
Since PO=OQ (because both are perpendicular to AB):
OQ2=PA2+102
Substituting the known values:
62=PA2+102
36=PA2+100
PA2=36−100=−64(This is incorrect; we should have used the correct relation)
We actually need to relate PO and PA correctly:
PA2=PO2+OA2
Substituting PO=OQ=6 and OA=10:
PA2=62+102
PA2=36+100=136
Now we can find PA:
PA=√136=2√34 cm
Thus, the length of PA is 403 cm.
Topper's Solved these Questions
Similar Questions
PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T (see Fig. 10.10). Find the length TP.
View SolutionIn the adjoining figure, AB is a chord of length 9.6 cm of a circle with centre O and radius 6 cm. The tangents at A and B intersect at P. Find the length of PA.
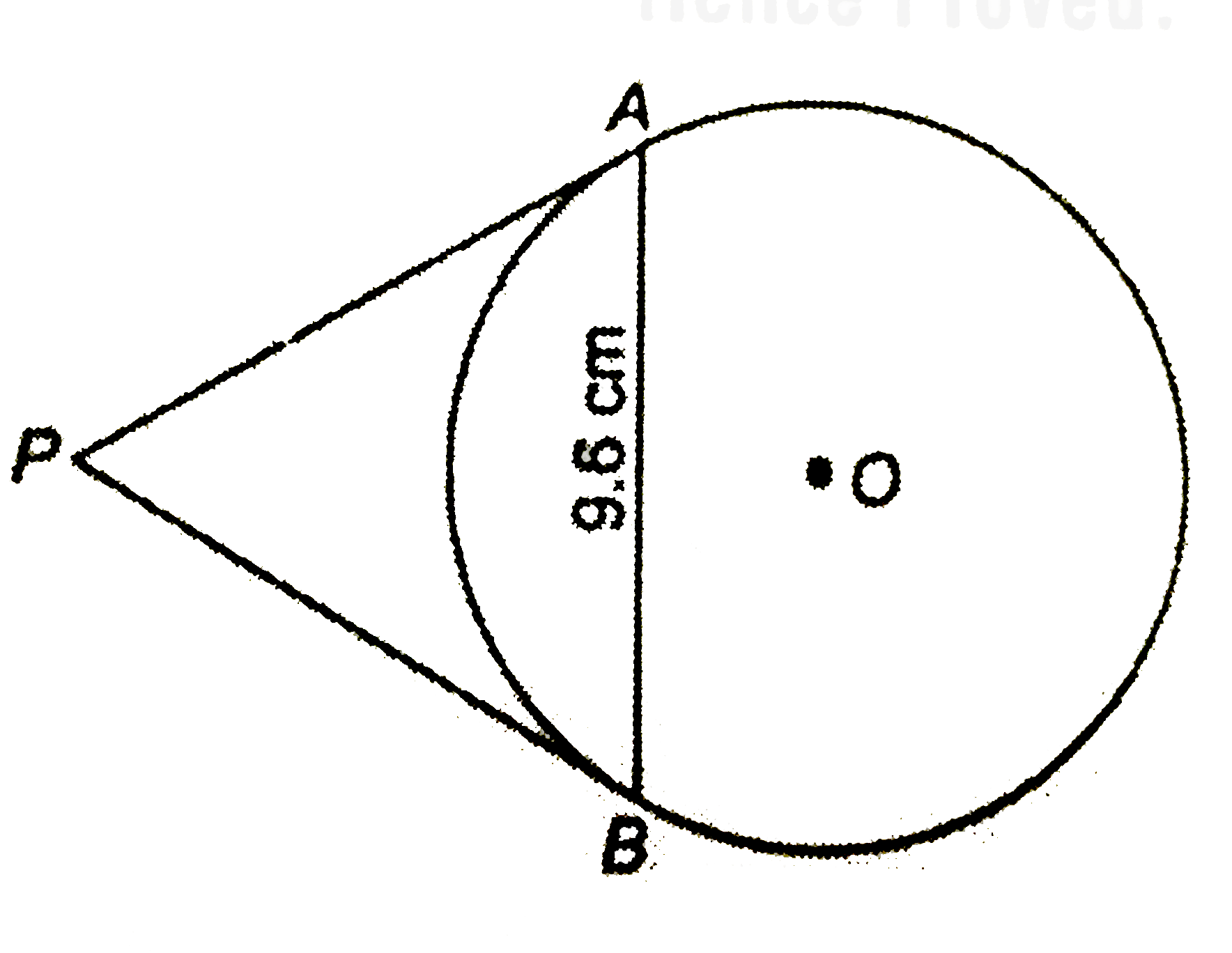
View SolutionDraw a circle of radius 3.0 cm. Take a point P on it. Construct a tangent at point P.
View SolutionO is the centre of a circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E, find the length AB.
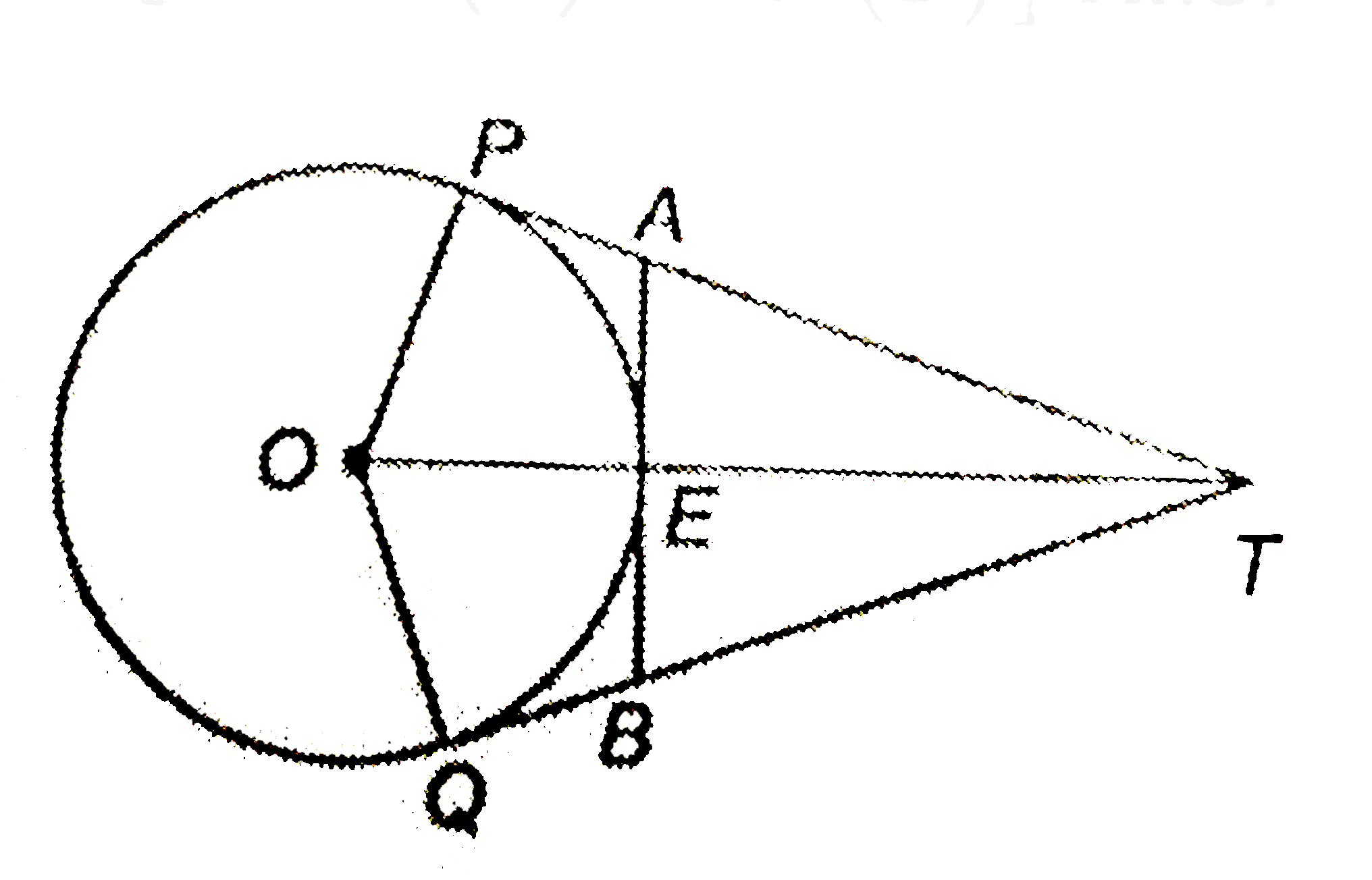
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ=13cm . Find the length of PQ .
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ=12cm. Length PQ is :
(A) 12 cm (B) 13 cm (C) 8.5 cm (D) √119cm.
View SolutionA chord of length 6 cm is drawn in a circle of radius 5 cm. Calculate its distance from the centre of the circle.
View SolutionA chord of a circle of radius 10cm subtends a right angle at the centre. The length of the chord (in cm) is
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q such that OQ=12cm . Length PQ is
View Solution