A circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk each other. Find the length of the string of each phone.
To solve the problem, we need to find the length of the string of each phone that the boys Ankur, Syed, and David are using to communicate while sitting at equal distances on the boundary of a circular park with a radius of 20 meters.
1. Understand the Configuration:
- The three boys are sitting at equal distances on the boundary of a circular park. This means they form an equilateral triangle.
- Let’s denote the positions of the boys as points A (Ankur), S (Syed), and D (David) on the circumference of the circle.
2. Define the Radius:
- The radius of the circular park is given as R=20 meters.
3. Equilateral Triangle:
- Since the boys are sitting at equal distances, triangle ASD is equilateral. Let the length of each side of the triangle be X meters.
4. Draw the Diagram:
- Draw a perpendicular line from the center of the circle (O) to the side SD of the triangle. Let this perpendicular meet SD at point B.
5. Use Properties of the Triangle:
- Since OB is perpendicular to SD, it bisects SD. Therefore, SB=BD=X2.
6. Apply Pythagorean Theorem:
- In triangle ABS, we can apply the Pythagorean theorem:
AS2=AB2+BS2
- Substituting the known values:
X2=AB2+(X2)2
- This simplifies to:
X2=AB2+X24
- Rearranging gives:
AB2=X2−X24=3X24
- Thus, we find:
AB=√3X24=√32X
7. Relate AB to Radius:
- Since AB=AO+OB and AO=20 meters (the radius), we have:
OB=AB−AO=√32X−20
8. Use Pythagorean Theorem in Triangle OBS:
- In triangle OBS, we apply the Pythagorean theorem:
OS2=OB2+SB2
- Substituting the known values:
202=(√32X−20)2+(X2)2
- This expands to:
400=(34X2−20√3X+400)+X24
- Combining terms gives:
400=X2−20√3X+400
- Thus, we simplify to:
0=X2−20√3X
9. Solve for X:
- Factoring out X:
X(X−20√3)=0
- This gives us:
X=20√3 meters
10. Conclusion:
- Therefore, the length of the string of each phone is 20√3 meters.
- The three boys are sitting at equal distances on the boundary of a circular park. This means they form an equilateral triangle.
- Let’s denote the positions of the boys as points A (Ankur), S (Syed), and D (David) on the circumference of the circle.
- The radius of the circular park is given as R=20 meters.
- Since the boys are sitting at equal distances, triangle ASD is equilateral. Let the length of each side of the triangle be X meters.
- Draw a perpendicular line from the center of the circle (O) to the side SD of the triangle. Let this perpendicular meet SD at point B.
- Since OB is perpendicular to SD, it bisects SD. Therefore, SB=BD=X2.
- In triangle ABS, we can apply the Pythagorean theorem:
AS2=AB2+BS2
- Substituting the known values:
X2=AB2+(X2)2
- This simplifies to:
X2=AB2+X24
- Rearranging gives:
AB2=X2−X24=3X24
- Thus, we find:
AB=√3X24=√32X
- Since AB=AO+OB and AO=20 meters (the radius), we have:
OB=AB−AO=√32X−20
- In triangle OBS, we apply the Pythagorean theorem:
OS2=OB2+SB2
- Substituting the known values:
202=(√32X−20)2+(X2)2
- This expands to:
400=(34X2−20√3X+400)+X24
- Combining terms gives:
400=X2−20√3X+400
- Thus, we simplify to:
0=X2−20√3X
- Factoring out X:
X(X−20√3)=0
- This gives us:
X=20√3 meters
- Therefore, the length of the string of each phone is 20√3 meters.
Topper's Solved these Questions
Similar Questions
A circular park of radius 20m is situated in a colony. Three boys Ankur, Syed andDavid are sitting at equal distance on its boundary each having a toy telephone inhis hands to talk each other. Find the length of the string of each phone.
View SolutionA circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk to each other. Find the length of the string of each phone.
View SolutionA circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk to each other. Find the length of the string of each phone.
View SolutionThree girls skating on a circular ice ground of radius 200m start from a point (P) on the edge of the ground and reach a point Q diametrically opposite to (P) following different paths as shown in Fig. What is the magnitude of the displacement vector for each ? which girl's displacement is equal to the actual length of path skate ?
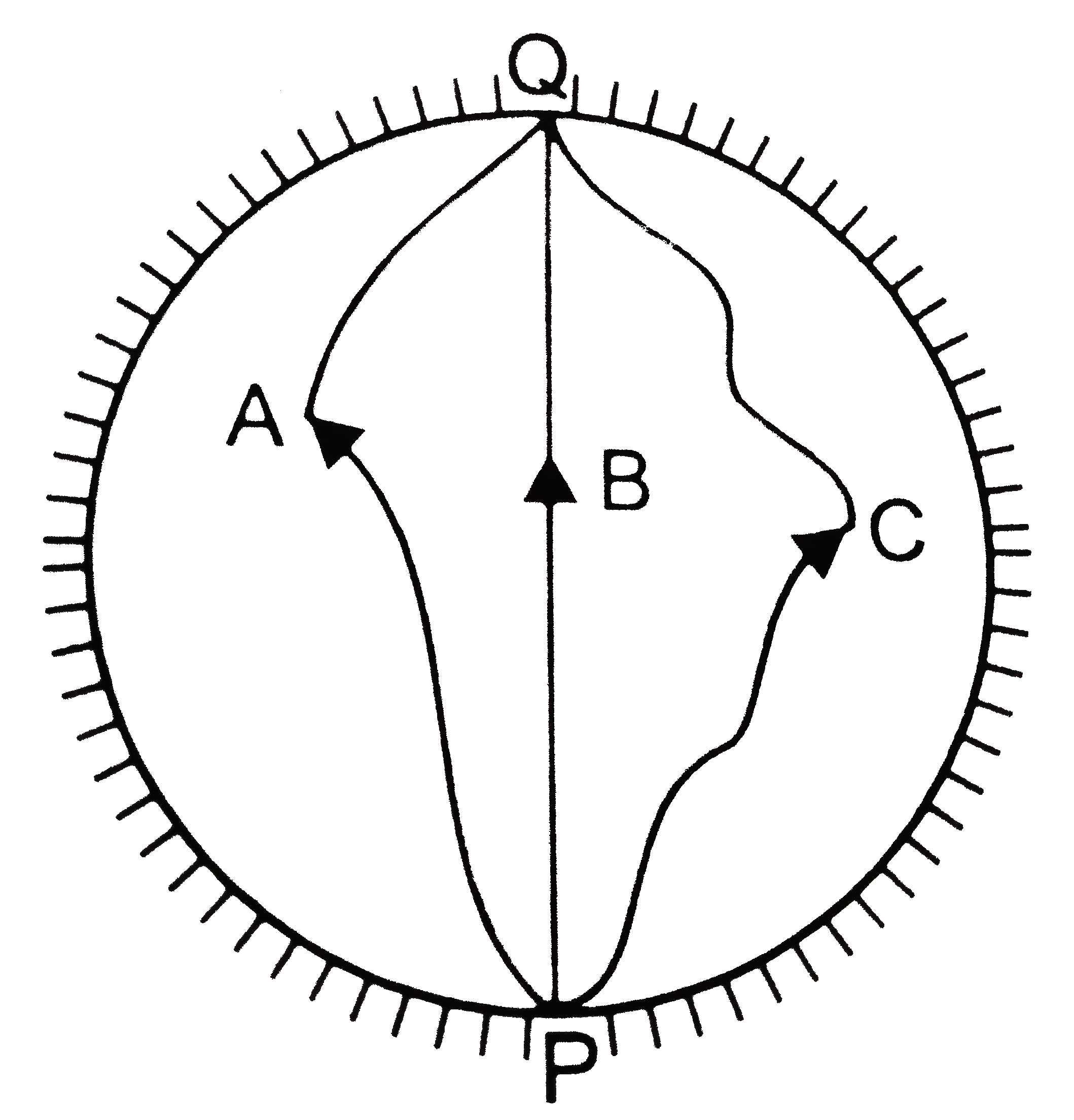 .
.
View SolutionA uniform thin cylindrical disk of mass M and radius R is attaached to two identical massless springs of spring constatn k which are fixed to the wall as shown in the figure. The springs are attached to the axle of the disk symmetrically on either side at a distance d from its centre. The axle is massless and both the springs and the axle are in horizontal plane. the unstretched length of each spring is L. The disk is initially at its equilibrium position with its centre of mass (CM) at a distance L from the wall. The disk rolls without slipping with velocity →V0=vacV0ˆi. The coefficinet of friction is μ.
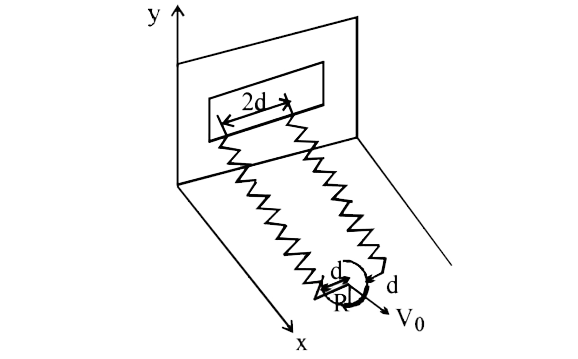
The centre of mass of the disk undergoes simple harmonic motion with angular frequency ω equal to -
View SolutionA uniform bar of length 12L and mass 48m is supported horizontally on two fixed smooth tables as shown in figure. A small moth (an insect) of mass 8m is sitting on end A of the rod and a spider (an insect) of mass 16m is sitting on the other end B. Both the insects moving towards each other along the rod with moth moving at speed 2v and the spider at half this speed (absolute). They meet at a point P on the rod and the spider eats the moth. After this the spider moves with a velocity v2 relative to the rod towards the end A. The spider takes negligible time in eating on the other insect. Also, let v=LT where T is a constant having value 4s.
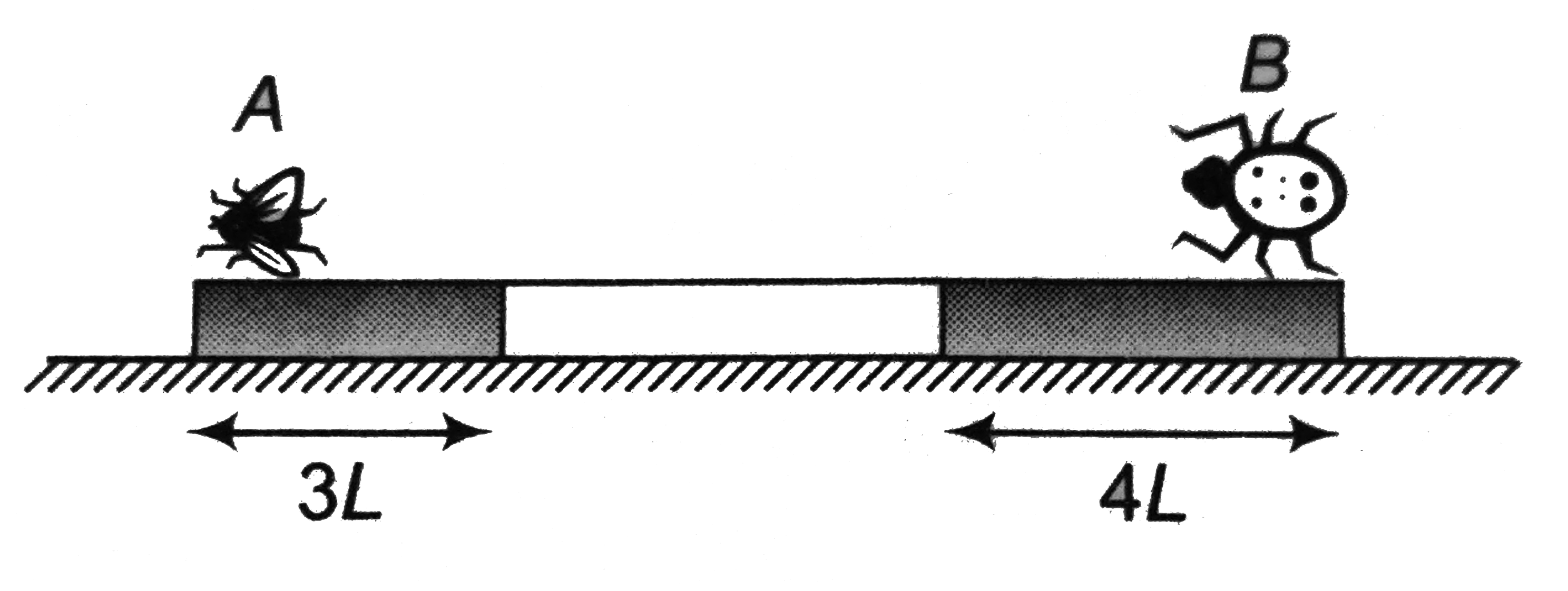
By what distance the centre of mass of the rod shifts during this time?
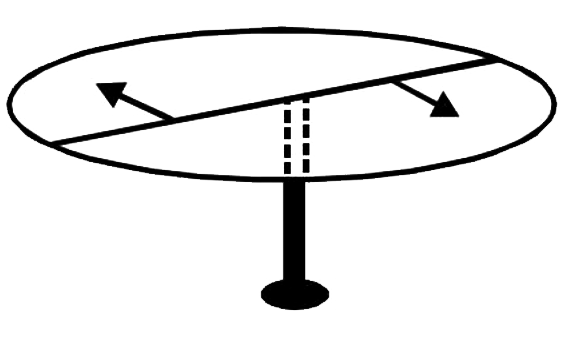
View SolutionA horizontal circular platform of radius 0.5 m and mass axis. Two massless spring toy-guns, each carrying a steel ball of mass 0.05 kg are attached to the platform at a distance 0.25m from the centre on its either sides along its diameter (see figure). Each gun simultaneously fires the balls horizontally and perpendicular to the diameter in opposite directions. After leaving the platform, the balls have horizontal speed of 9ms−1 with respect to the ground. The rotational speed of the platform in rads−1 after the balls leace the platform is
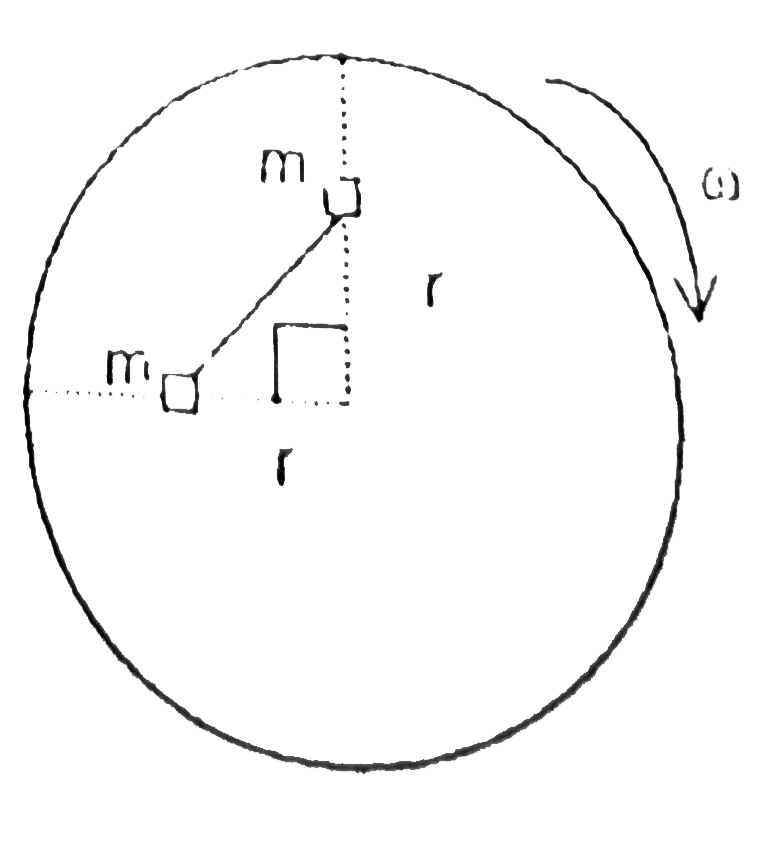
View SolutionTwo particles of mass m each are kept on a horizontal circular platfrom on two mutually perpendicular radii at equal distance r from the center of the table. The particles are connected with a string, which is just taught when the platfrom is not rotating. The coefficient of static friction between the platfrom and block is μ (now if angular speed of platfrom m is slowly increased). Find the maximum angular speed (ω) of platfrom about it center so that the blocks remain stationery relative to platform. (If μ=1√2, r=2.5m and g=10m/s2)
View SolutionA track consists of two circular pars ABC and CDE of equal radius 100 m and joined smoothly as shown in figure.Each part subtends a right angle at its centre. A cycle weighing 100 kg together with rider travels at a constant speed of 18 km/h on the track. A. Find the normal contact force by the road on the cycle when it is at B and at D. b.Find the force of friction exerted by the track on the tyres when the cycle is at B,C and D. c. Find the normal force between the road and the cycle just before and just after the cycle crosses C. d. What should be the minimum friction coefficient between the road and the tyre, which will ensure that the cyclist can move with constant speed? Take g=10ms2

View SolutionConsider a point charge q=1mC placed at a corner of a cube of sides 10 cm . Determine the electric flux through each face of the cube.
Strategy : We will learn about the utility of symmetry in solving problems with the help of Gauss's law . Here we'll use the symmetry of the situation,which involves the faces joining at the corner at which the charge resides.

(a) A charge q is placed atthe corner of a cube. (b) By surrounding the charge with a series of cubes such that the charge is at the centre of a larger cube, we have created an arrangement sufficiently symmetric to be able to solve for desired flux values.
You can see from figure that for these faces →E.ˆn=0. Since the normal is perpendicular to the surfaces while the electric field goes off in a spherically symmetric pattern and lies in the sides . In other words , the electric field that originates at the charge is tangential to the surface of these three sides. This means there is no flux through these sides. The electric flux through each of the remaining three faces of the be must be equal by symmetry . We'll referto these faces with the label F.
To fidn the flux through each of the sides F, we acan use a technique that puts the single charge in the middle of a larger cube. It takes seven other similarly placed cubes to surrounds the points charge q completely in figre. The charge is at the dead centre of the enw larger cube. So, the flux through each of the six sides of the large cube will now have an electric flux of one sixth of the total flux F. So given that the total structure is completely symmetric, the flux through a side F is one fourth of the flux through the larger side.
View Solution
