An empty plastic box of mass m is found to accelerate up at the rate of g/6 when placed deep inside water. How much sand should be put inside the box so that it may accelerate down at the rate of g/6?
Let the air resistance force is Fa aned Bouyant force is B.
Given that, Fa=av
where v= velocity
→FM=kv,
where k= proportioN/Ality constant

When the ballon is moving downward
B+kv=Mg ................i.
→m=b+kvg
fortheball∞n→risewithaconstantvelocityv(upward)≤tthemassofm<br> Here , B-(mg+kv)=0<br> B=mg+kv<br>rarr m=(B+kv)/g<br>:.Amountofmasstˆshod––beremoved=M−m
=B+kvg−B−kvg
=B+kv−B+kvg
2kvg=2(Mg−B)g
2{M−Bg}
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Questions for short Answer
17 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective -2
9 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMA ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - All Questions
64 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer
14 Videos
Similar Questions
An empty plastic box of mass 5 kg is observed to accelerate up at the rate of g/6 when placed deep inside water. What mass of sand should be put inside the box so that it may accelerate dow at the rate of g/6 ?
View SolutionA balloon with mass m is descending down with an acceleration a (wherea<g) . How much mass should be removed from it so that it starts moving up with an acceleration a?
View Solution
Knowledge Check
The length, width and depth of a rectangular box are 6 feet, 5 feet and 7 feet, respectively. A hose supplies water at a rate of 6 cubic feet per minute. How much time in minutes would it take to fill a conical box whose volume is three times the volume of the rectangle box?
A105B125C205D235
A balloon of mass m is descending down with an acceleration How much mass should be g2 removed from it so that it starts moving up with same acceleration?
View SolutionA jet is releasing water at the rate of 6 kg/see with speed of 4 m/sec. Now this water strikes a block of mass 10 kg. find the force exerted on block & its acceleration ?
View SolutionA hot- air balloon consists of a basket, one passenger, and some carge. Let the total mass be M. Even though there is an upward lift force on the balloon, the balloon is initially acceleration downwards at a rate of g/3.
(a) Draw a free-body diagram for the descending balloon.
(b) Find the upward lift force in terms of the initial total weight Mg.
(c ) The passenger notices that he is heading straight for a waterfall and decides he needs to go up. What fraction of the total weight must he drop overboard so that the balloon accelerates upward at a rate of g/2? Assume that the upward lift force remains the same.
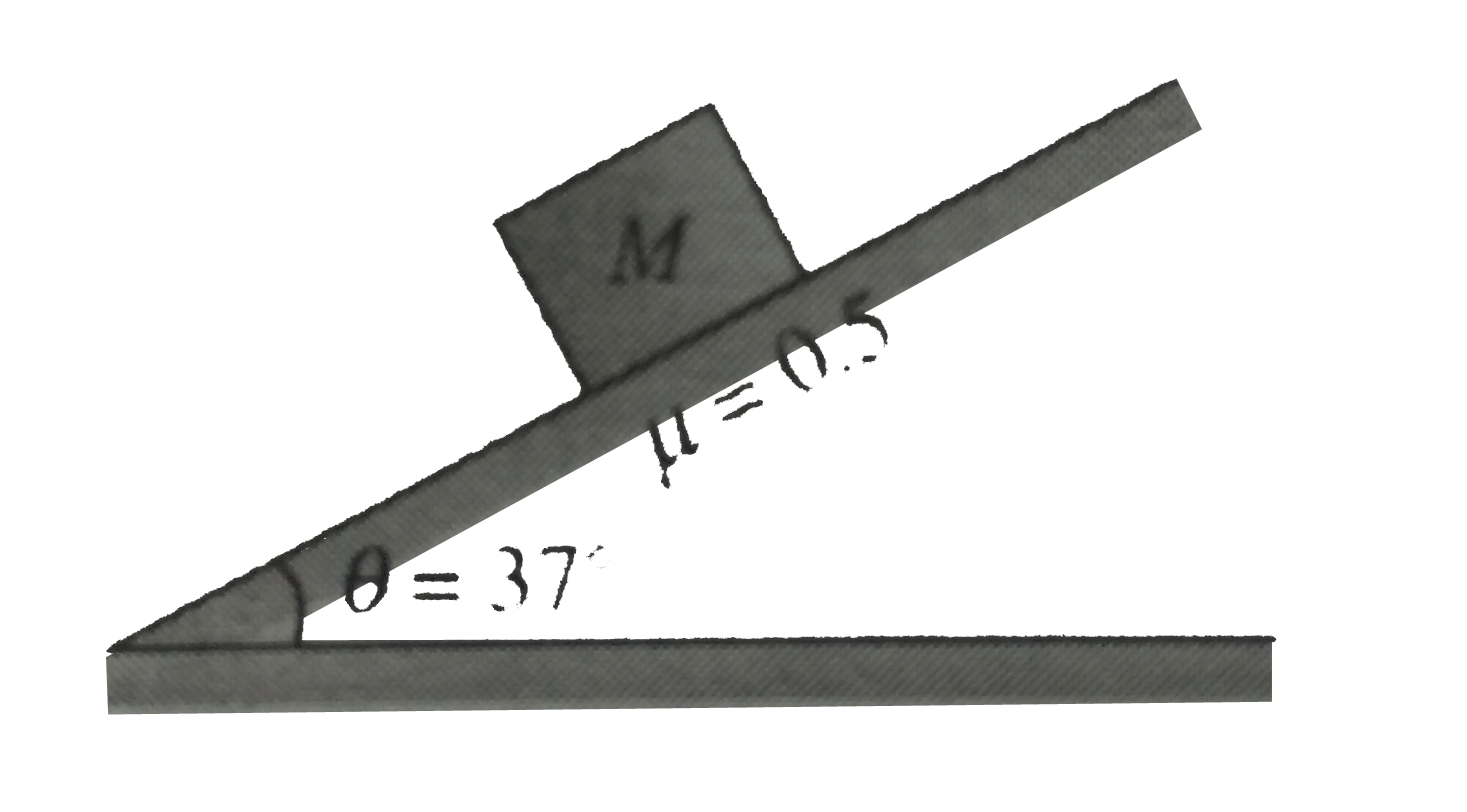
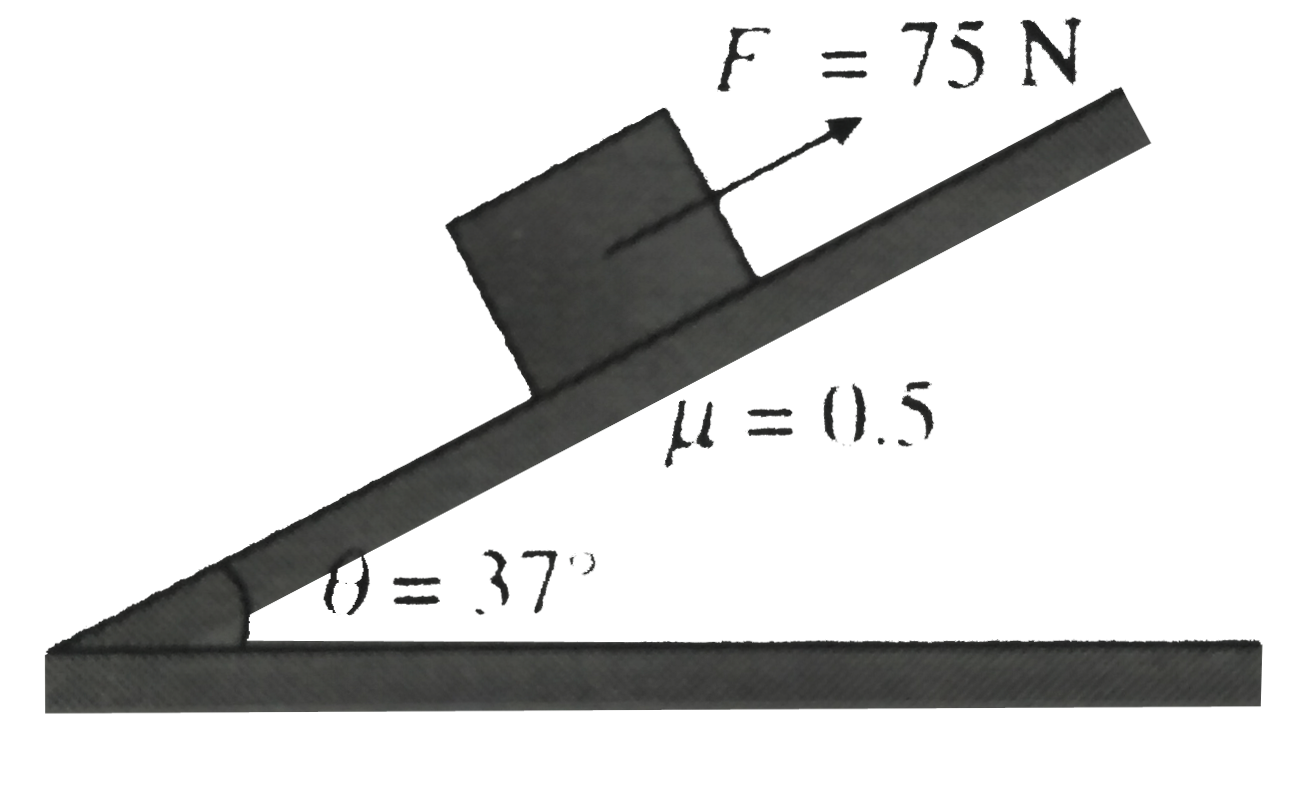
View SolutionA block of mass M=10kg is placed inclined plane , inclined at angle θ=37∘ with horizontal The coefficient of friction between the block and inclined is μ=0.5
a. Calculate the acceleration of the block when it is released
b. Now a force F=75N is appling on block as shown find out the acceleration of the block If the block is initially at rest .
c. In case (b), how much force should be added to 75N force so that block the starts to move up the incline.
d. In case (b), what is the minimum force by which 75N force should be replaced with so that the block does not move?
View SolutionA man of mass m runs without sliding from rest from one end of a boat of mass M and length l with an acceleration a relative to the boat. If the friction between water and boat is neglected find the

a. acceleration of CM of the system (M+m)
b. acceleration of the man and boat
c. position of man at the time when he reaches to other end of the boat
d. frictional force
e. work done by friction on boat.
f. total work done by friction.
g. velocities of man and boat when the man reaches other end of the boat.
h. work down by man
View SolutionFigure shows water in a container having 2.0−mm thick walls made of a material of thermal conductivity 0.50Wm−1C−1 . The container is kept in a melting-ice bath at 0∘C . The total surface area in contact with water is0.05m2 . A wheel is clamped inside the water and is coupled to a block of mass M as shown in the figure. As the goes down, the wheel rotates. It is found that after some time a steady state is reached in which the block goes down with a constant speed of 10cms−1 and the temperature of the water remains constant at 1.0∘C .Find the mass M of the block. Assume that the heat flow out of the water only through the walls in contact. Take g=10ms−2 .

View SolutionAn amusement park ride consist of a large verticle cylinder that spins about its axis first fast enough that any person inside is held up againest the wall when the is μs and the radius of the cylider is R

a. Shown that maximum period of revolation neccessary to keep the person from falling is T=(4π2μsR/g)1/2
b. Obtain a numerical value for T taking R=4.00m and μs=0.400 . How many revolations per minute does the cylinder make?
c. If the rate of revolation of the cylinder is make to be some what larger what happens to the magnitude of each one of the forces acting on the person ? What happens to the motion of the person ?
d. If instead the cylinder rate revolution is made to be somewhat smaller what happens to the magnitude and what happens to the motion of the person ?
View Solution
