Question

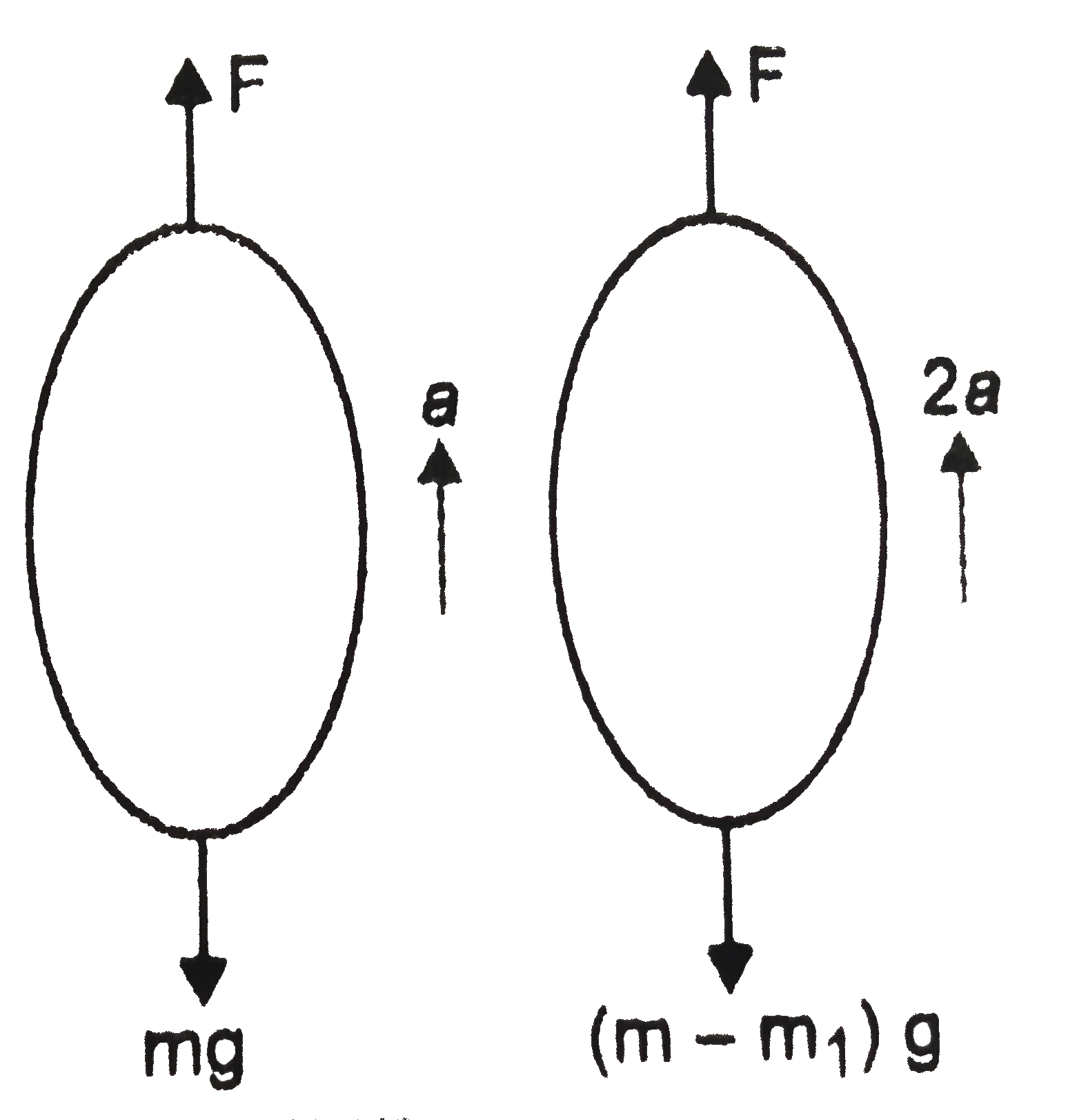
A balloon with mass $m$ is descending down with an acceleration a $(wherea<g)$ . How much mass should be removed from it so that it starts moving up with an acceleration a?

Options

A. `(2M(g))/(a)`
B. `(M(g +a))/(g)`
C. `(Mg)/(g + a)`
D. `(2Ma)/(g + a)`

Answer

D

Explanation

To solve the problem of how much mass should be removed from a descending balloon so that it starts moving upwards with a specified acceleration, we can follow these steps:

Step 1: Analyze the Forces Acting on the Balloon
The balloon has a mass `m` and is descending with an acceleration `a`, where `a < g` (g is the acceleration due to gravity). The forces acting on the balloon are:
- The weight of the balloon, which is `W = mg` (downward).
- The buoyant force `F` (upward).

Step 2: Set Up the Equation for Downward Motion
Since the balloon is descending with an acceleration `a`, we can apply Newton's second law:
\( mg - F = ma \)
Rearranging gives us:
\( F = mg - ma \)
This is our Equation 1.

Step 3: Analyze the Forces When Mass is Removed
Let’s denote the mass that needs to be removed as `m'`. After removing this mass, the new mass of the balloon becomes `m - m'`. We want the balloon to ascend with the same acceleration `a`.

Step 4: Set Up the Equation for Upward Motion
For the balloon to move upwards with an acceleration `a`, we can write:
\( F - (m - m')g = (m - m')a \)
Rearranging gives us:
\( F = (m - m')g + (m - m')a \)
This is our Equation 2.

Step 5: Substitute Equation 1 into Equation 2
From Equation 1, we know that:
\( F = mg - ma \)
Now we substitute this expression for `F` into Equation 2:
\( mg - ma = (m - m')g + (m - m')a \)

Step 6: Expand and Rearrange the Equation
Expanding the right side:
\( mg - ma = mg - m'g + ma - m'a \)
Now, we can cancel `mg` from both sides:
\( -ma = -m'g + ma - m'a \)
Rearranging gives:
\( -ma - ma = -m'g - m'a \)
This simplifies to:
\( -2ma = -m'g - m'a \)

Step 7: Factor Out m'
Rearranging gives:
\( 2ma = m'(g + a) \)
Now, we can solve for `m'`:
\( m' = \frac{2ma}{g + a} \)

Conclusion
The mass that should be removed from the balloon so that it starts moving upwards with an acceleration `a` is:
\( m' = \frac{2ma}{g + a} \)

Final Answer
The correct option is Option 4: \( m' = \frac{2ma}{g + a} \).

---