The free surface of a liquid resting in an inertial frame is horizontal. Does the normal to the free surface pass through the centre of the earth? Think separately if the liquid is a. at te equaltor b. at a pole c. somewhere else.
To solve the question, we need to analyze the behavior of the free surface of a liquid in relation to the gravitational forces acting on it at different locations on Earth: at the equator, at the poles, and at some other location.
1. Understanding the Free Surface of a Liquid:
- The free surface of a liquid at rest in an inertial frame is horizontal. This means that the surface is level and does not slope in any direction.
2. Gravitational Force:
- The gravitational force acts towards the center of the Earth. This force is uniform and acts on every particle of the liquid.
3. Normal Force:
- The normal force acts perpendicular to the surface of the liquid. Since the free surface is horizontal, the normal force will also be vertical, acting upwards against the weight of the liquid.
4. Analyzing Different Locations:
- At the Equator:
- At the equator, the gravitational force acts directly towards the center of the Earth. Therefore, the normal to the free surface (which is vertical) will pass through the center of the Earth.
- At the Pole:
- At the poles, the situation is similar. The gravitational force still acts directly towards the center of the Earth. Hence, the normal to the free surface will also pass through the center of the Earth.
- Somewhere Else (Mid-Latitudes):
- In mid-latitudes, the gravitational force still acts towards the center of the Earth. The free surface remains horizontal, and thus the normal to this surface will also pass through the center of the Earth.
5. Conclusion:
- In all three cases (at the equator, at the poles, and at other locations), the normal to the free surface of the liquid will pass through the center of the Earth.
Final Answer:
Yes, the normal to the free surface of the liquid passes through the center of the Earth at the equator, at the poles, and at any other location.
- The free surface of a liquid at rest in an inertial frame is horizontal. This means that the surface is level and does not slope in any direction.
- The gravitational force acts towards the center of the Earth. This force is uniform and acts on every particle of the liquid.
- The normal force acts perpendicular to the surface of the liquid. Since the free surface is horizontal, the normal force will also be vertical, acting upwards against the weight of the liquid.
- At the Equator:
- At the equator, the gravitational force acts directly towards the center of the Earth. Therefore, the normal to the free surface (which is vertical) will pass through the center of the Earth.
- At the Pole:
- At the poles, the situation is similar. The gravitational force still acts directly towards the center of the Earth. Hence, the normal to the free surface will also pass through the center of the Earth.
- In mid-latitudes, the gravitational force still acts towards the center of the Earth. The free surface remains horizontal, and thus the normal to this surface will also pass through the center of the Earth.
- In all three cases (at the equator, at the poles, and at other locations), the normal to the free surface of the liquid will pass through the center of the Earth.
Yes, the normal to the free surface of the liquid passes through the center of the Earth at the equator, at the poles, and at any other location.
Topper's Solved these Questions
Similar Questions
A very light, rectangular wire-frame of dimensions 7cm×5cm hangs just above the free surface of a liquid of surface tension T, with its plane parallel to the free surface. The wire -frame is just brought in contact with the liquid surface and then, lifted up. If the force required to lift the wire-frame is 3.36 N, then what is the value of T in (Nm−1)?
View SolutionThe angle which the free surface of a liquid filled in a container will make with horizontal if the container is accelerated horizontally with acceleration g√3 is
View SolutionA vessel contains a liquid has a constant acceleration 19.6m/s2 in horizontal direction. The free surface of water get sloped with horizontal at angle
View SolutionA small body of mass 100 g moving with a velocity 2 m/s is strikes a solid cylinder of mass 2 kg and radius 20 cm. The cylinder is initially at rest and is mounted on a fixed horizontal axle that passes through the centre of mass. The line of motion of the small body is at a perpendicular lar distance of 15 cm from the centre of the cylinder. The small body strikes the cylinder and adheres to its surface. What is the angular speed of the system just after the collision ? Assume there is no friction.
View SolutionA trolley containing a liquid slides down a smooth inclined plane of angle α with the horizontal. Find the angle of inclination θ of the free surface with the horizontal.

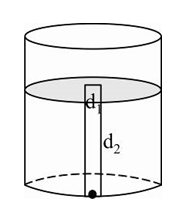
View SolutionA thin rod of length L and area of cross section S is pivoted at its lowest point P inside a stationary, homogeneous and non-viscous liquid. The rod is free to rotate in a vertical plane about a horizontal axis passing through P. The density d1of the rod is smaller than the density d2 of the liquid. The rod is displaced by a small angle theta from its equilibrium position and then released. Shown that the motion of the rod is simple harmonic and determine its angular frequency in terms of the given parameters ___________ .
View SolutionA ray of light is incident on a surface in a direction given by vector A=2i−2j+k. The normal to that surface passing through the point of incidence is along the vector N=j−2k. The unit vector in the direction of reflected ray is given by R=aj+bj+ck. Find three equations in terms of a,b,c using which we can find the values of a,b,&c
View SolutionA plate of area 2m2 is made to move horizontally with a speed of 2m/s by applying a horizontal tangential force over the free surface of a liquid. If the depth of the liquid is 1m and the liquid in contact with the bed is stationary. Coefficient of viscosity of liquid is 0.01 poise. Find the tangential force needed to move the plate.
View SolutionA plate of area 2m2 is made to move horizontally with a speed of 2m/s by applying a horizontal tangential force over the free surface of a liquid. If the depth of the liquid is 1m and the liquid in contact with the bed is stationary. Coefficient of viscosity of liquid is 0.01 poise. Find the tangential force needed to move the plate.
View SolutionThe force of interaction between the molecules of same substance is called cohesive force hile force of interaction between molecules of different substances is called adhesive force . Both the forces are attracive and have a range of about 10 A . If we consider a molecule . A well inside a liquid we find that it is equally attracted by the surrounding molecules in all the dirctions ( with a sphere of radius 10 Å ) and so it does not experience any resultant force . On or near the surface of a liquid , the molecules do not exist on the free side and so a molecule ( say B ) experiences a resultant force vertically downwards into the liquid . For this reason , the molecules of the surface have a tendency to go into the liquid . Thus , the surface tends to contract and gives rise to surface tension .
As surface molecules like B experience a net downward force of cohension and have tendency to go down , so in increasing the area of the surface more molecules are to be shifted to the surface from inside . Thus , more mechnical work will have to be done against the net downward force of cohension . This work is stored by the molecules in the surfaces as potential energy called free surface energy .
Furthermore , molcules like P , in contact with the liquid will stick to the solid surface , i.e will meet it if the force of adhesion A is greater than net force of cohension C . The liquid will not the solid surface if C>A
Also , molecules like Q at the surface in contact with the solid will experience a net force of cohension CR . If adhesion A is greater then cohension CR , the resultant force will be F . As the liquid can't support tangential force , F must be perpendicular to the surface of the liquid . So , the menisus will be concave and the angle of contact will be acute (>90∘) . However , if adhesion is lesser than cohension , the resultant force F will be directed into the liquid and so the menisus ( which is perpendicular to F as liquid support tangential force ) will be convex and the angle of contact will be obtuse (>90∘)
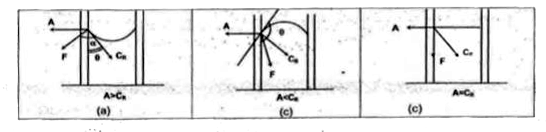
The free suface energy , stored in the surface is because of work done
View Solution
