An empty balloon weighs W1. If air equal in weight to W is pumped into the balloon, the weight of the balloon becomes W2. Suppose that the density of air inside and outside the balloon is the same. Then
W2=W1
W2=W1+W
W2<W1+W
W2>W1
The correct Answer is:A, C
To solve the problem, we need to analyze the situation step by step.
Step 1: Understand the Initial Conditions
- The weight of the empty balloon is denoted as W1.
- When air equal in weight to W is pumped into the balloon, the new weight of the balloon becomes W2.
Step 2: Define the New Volume
- When air is pumped into the balloon, the volume of the balloon changes. The volume V of the air inside the balloon can be expressed in terms of the mass of the air and its density:
V=massdensity=W/gρ
where W is the weight of the air added, g is the acceleration due to gravity, and ρ is the density of air.
Step 3: Calculate the Real Weight of the Balloon
- The real weight of the balloon after adding the air is:
Real Weight=W1+W
Step 4: Consider the Buoyancy Force
- The balloon is in the atmosphere, which exerts a buoyant force FB on it. The buoyant force is equal to the weight of the air displaced by the volume of the balloon:
FB=ρgV
- From the previous step, we know that V=Wρg, so substituting this into the buoyant force equation gives:
FB=ρg(Wρg)=W
Step 5: Calculate the Apparent Weight of the Balloon
- The apparent weight W2 of the balloon (the weight we measure) is given by:
W2=Real Weight−FB
Substituting the values we found:
W2=(W1+W)−W
Simplifying this gives:
W2=W1
Conclusion
- Therefore, we conclude that:
W2=W1
- This means the apparent weight of the balloon after pumping in air equal to weight W is the same as the weight of the empty balloon.
Final Answer
The correct option is that W1=W2.
---
- The weight of the empty balloon is denoted as W1.
- When air equal in weight to W is pumped into the balloon, the new weight of the balloon becomes W2.
- When air is pumped into the balloon, the volume of the balloon changes. The volume V of the air inside the balloon can be expressed in terms of the mass of the air and its density:
V=massdensity=W/gρ
where W is the weight of the air added, g is the acceleration due to gravity, and ρ is the density of air.
- The real weight of the balloon after adding the air is:
Real Weight=W1+W
- The balloon is in the atmosphere, which exerts a buoyant force FB on it. The buoyant force is equal to the weight of the air displaced by the volume of the balloon:
FB=ρgV
- From the previous step, we know that V=Wρg, so substituting this into the buoyant force equation gives:
FB=ρg(Wρg)=W
- The apparent weight W2 of the balloon (the weight we measure) is given by:
W2=Real Weight−FB
Substituting the values we found:
W2=(W1+W)−W
Simplifying this gives:
W2=W1
- Therefore, we conclude that:
W2=W1
- This means the apparent weight of the balloon after pumping in air equal to weight W is the same as the weight of the empty balloon.
The correct option is that W1=W2.
Topper's Solved these Questions
Similar Questions
A ballon has 2 g of air . A small hole is pierced into it . The air comes out with relative velocity 4 m/s . If the balloon shrinks completely in 2.5 s , the average force acting on the balloon is
View SolutionA rubber balloon has 200 g of water in it. Its weight in water will be (neglect the weight of balloon)
View SolutionHow does a hot air balloon work?
View SolutionStatement-1: Weight of a empty balloon measured in air is w1. If air at atmospheric pressure is filled inside balloon and again weight of balloon is measured. Weight of balloon is w2 is second case. Then W2 is equal to w1
Statement-2: Upthrust is equal to weight of the fluid displaced by the body.
View SolutionA conducting bolloon of the radius a is charged to potential V0 and held at a large height above the earth ensures that charge distribution on the surface of the balloon remains unaffected by the presence of the earth. It is connected to the earth through a resistance R and a value in the balloon is opened . The gas inside the balloon escapes from value and the size of the balloon decreases. The rate of decrease in radius of the balloon is controlled in such a manner that potential of the balloon remains constant. Assume the electric permittivity of the surrounding air equals to that of free space (ε0) and charge cannot leak to the surrounding air.
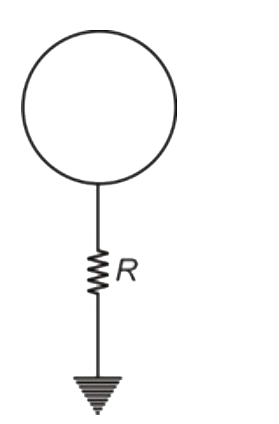
The rate at which radius r of the balloon changes with time is best represented by the equation
View SolutionTwo identical He-filled spherical balloons each carrying a charge q are tied to a weight W with strings and float in equlibrium, as shown in figure.
(i) Find the magnitude of q, assuming that the charge on each balloon acts as if it were concentrated at the center.
(ii) Find the volume of each balloon. Take the density of He as ρHe and the density of air as ρa. Ignore the weight of the unfilled balloons .
 ,
,
View SolutionThe force of buoyancy exerted by the atmosphere on a balloon is B in the upward direction and remains constant. The force of air resistance on the balloon acts opposite to the direction of velocity and is proportional to it. The balloon carries a mass M and is found to fall down near the earth's surface with a constant velocity v. How uch mass hsould be removed from teh balloon so that it may rise with a constant velocity v?
View SolutionA body weighs W newton at the surface of the earth. Its weight at a height equal to half the radius of the earth, will be
View SolutionA body weighs W newton at the surface of the earth. Its weight at a height equal to half the radius of the earth, will be
View SolutionFor meteorological purposes , hot air is filled into balloons.
View Solution
