If water be used to construct a barometer, what would be the height of water column at a standard atmospheric presure (76cm of mercury)?
The correct Answer is:A, C
To find the height of the water column in a barometer at standard atmospheric pressure (76 cm of mercury), we can follow these steps:
1. Understand the relationship between pressure and height of liquid columns:
The pressure exerted by a liquid column is given by the formula:
P=ρgh
where P is the pressure, ρ is the density of the liquid, g is the acceleration due to gravity, and h is the height of the liquid column.
2. Identify the known values:
- The atmospheric pressure P0 is given as 76 cm of mercury.
- The density of mercury ρHg is approximately 13,600kg/m3.
- The density of water ρw is approximately 1,000kg/m3.
- Convert the height of mercury from centimeters to meters:
hHg=76cm=0.76m
3. Set up the equation for mercury:
The pressure exerted by the mercury column can be expressed as:
P0=ρHgghHg
4. Set up the equation for water:
The pressure exerted by the water column at the same height can be expressed as:
P0=ρwghw
5. Equate the two pressure equations:
Since both expressions equal P0, we can set them equal to each other:
ρHgghHg=ρwghw
6. Cancel g from both sides:
Since g is present in both equations, it can be canceled out:
ρHghHg=ρwhw
7. Solve for the height of the water column hw:
Rearranging the equation gives:
hw=ρHghHgρw
8. Substitute the known values:
Plug in the values for ρHg, hHg, and ρw:
hw=13,600kg/m3×0.76m1,000kg/m3
9. Calculate hw:
hw=10,336kg/m21,000kg/m3=10.336m
Rounding this gives:
hw≈10.34m
Final Answer:
The height of the water column at standard atmospheric pressure (76 cm of mercury) would be approximately 10.34 meters.
The pressure exerted by a liquid column is given by the formula:
P=ρgh
where P is the pressure, ρ is the density of the liquid, g is the acceleration due to gravity, and h is the height of the liquid column.
- The atmospheric pressure P0 is given as 76 cm of mercury.
- The density of mercury ρHg is approximately 13,600kg/m3.
- The density of water ρw is approximately 1,000kg/m3.
- Convert the height of mercury from centimeters to meters:
hHg=76cm=0.76m
The pressure exerted by the mercury column can be expressed as:
P0=ρHgghHg
The pressure exerted by the water column at the same height can be expressed as:
P0=ρwghw
Since both expressions equal P0, we can set them equal to each other:
ρHgghHg=ρwghw
Since g is present in both equations, it can be canceled out:
ρHghHg=ρwhw
Rearranging the equation gives:
hw=ρHghHgρw
Plug in the values for ρHg, hHg, and ρw:
hw=13,600kg/m3×0.76m1,000kg/m3
hw=10,336kg/m21,000kg/m3=10.336m
Rounding this gives:
hw≈10.34m
The height of the water column at standard atmospheric pressure (76 cm of mercury) would be approximately 10.34 meters.
Topper's Solved these Questions
Similar Questions
At a given place, a mercury barometer records a pressure of 0.70 m of Hg. What would be the height of water column if mercury in barometer is replaced by water? Take R.D. of mercury = 13.6.
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. What will be length of the air column above mercury in the tube now? (atmospheric pressure =76 cm of Hg)
View Solution
Knowledge Check
A manometer reads the pressure of a gas in an enclosure as shown in the figure. The absolute and gauge pressure of the gas in cm of mercury is (take atmospheric pressure =76 cm of mercury)
A76,20B20,76C96,20D20,96Torricelli's barometer used mercury but pascal duplicated it using French wine of density 984kgm−3 . In that case, the height of the wine column for normal atmospheric pressure is
(Take the density of mercury =1.36×103kgm−3)A5.5 mB10.5 mC9.8 mD15 m
A faulty barometer contains certains amount of air and saturated water vapour . It reads 74.0cm when the atmospheric pressure is 76.0cm of mercury and reads 72.10cm when the atmospheric pressure is 74.0cm of mercury.Saturation vapour pressure at air temperature =1.0cm of mercury. Find the length of the baromerer tube above the mercury level in the reservoir.
View SolutionA barometer correctly reads the atmospheric pressure as 76cm of mercury. Water droplets are slowly introduced into the barometer tube by a dropper. The height of the mercury column first decreases and then become constant. If the saturation vapour pressure at the atmospheric temperature is 0.80cm of mercury, find the height if the mercury column when it reaches its minimum value.
View SolutionWhy mercury is used in barometer for measuring atmospheric pressure?
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. What will be length of the air column above mercury in the tube now? (atmospheric pressure =76 cm of Hg)
View SolutionA cubical metal block of edge 12 cm floats in mercry with one fifth of the height inside the mercury. Water poured till the surface of the block is just immersed in it. Find the height of the water column to be poured. Specific gravity of mercury =13.6.
View SolutionA manometer reads the pressure of a gas in a enclosure as shown in figure(a) When some of the gas is removed by a pump, the manometer reads as in (b). The liquid used in the manometers is mercury and the atmospheric pressure is 76cm of mercury.
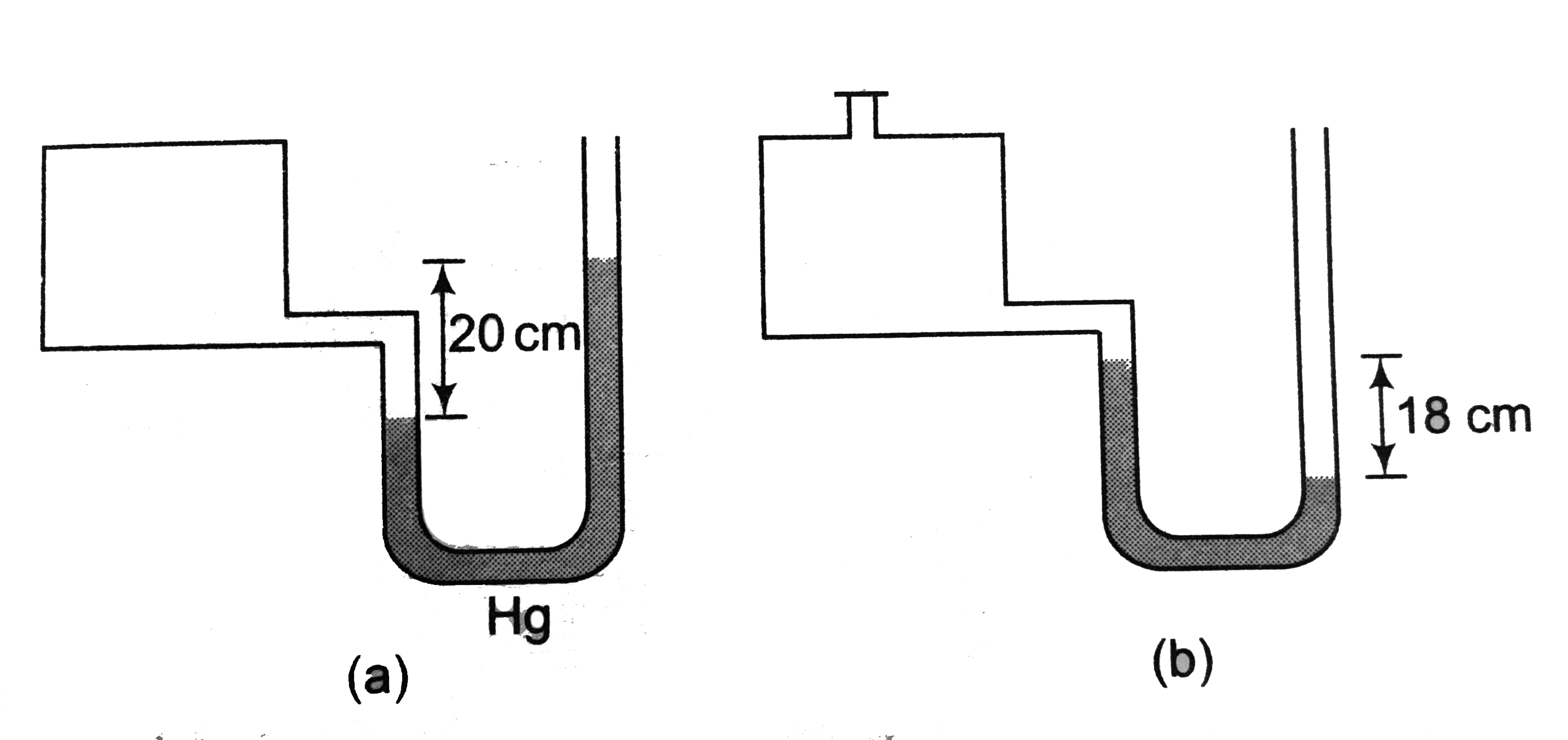
(i) Give the absolute and gauge pressure of the gas in the enclosure for cases (a) and (b) in units of cm of mercury .
(ii) How would the level change in case (b) if 13.6cm of water are poured into the right limb of the manometer?
View Solution