A cubical block of ice floating in water has to support a metal piece weighing 0.5 kg. What can be the minimum edge of the block so that it does not sink in water? specific gravity of ice=0.9.
The correct Answer is:A, C
To solve the problem, we need to determine the minimum edge length of a cubical block of ice that can float while supporting a metal piece weighing 0.5 kg. The specific gravity of ice is given as 0.9.
1. Understand the Forces Involved:
- The block of ice is floating in water and must support the weight of the metal piece.
- The buoyant force acting on the ice must equal the total weight of the ice plus the weight of the metal piece.
2. Identify the Weights:
- The weight of the metal piece (W_m) is given by:
Wm=m⋅g=0.5kg⋅9.8m/s2=4.9N
- The weight of the ice block (W_i) can be expressed as:
Wi=density of ice⋅g⋅Vi
- The volume of the ice block (V_i) is x3 where x is the edge length of the cube.
3. Calculate the Buoyant Force:
- The buoyant force (B) acting on the ice is equal to the weight of the water displaced, which is:
B=density of water⋅g⋅Vd
- Since the volume of the ice block submerged is equal to its volume when floating, we have:
Vd=x3
- Therefore, the buoyant force can be expressed as:
B=density of water⋅g⋅x3
4. Set Up the Equation for Equilibrium:
- At equilibrium, the buoyant force equals the total weight:
B=Wm+Wi
- Substituting the expressions we have:
density of water⋅g⋅x3=Wm+density of ice⋅g⋅x3
5. Substituting Known Values:
- The density of water is 1g/cm3 and the specific gravity of ice is 0.9, so:
density of ice=0.9g/cm3
- The equation simplifies to:
1⋅g⋅x3=4.9+0.9⋅g⋅x3
6. Cancel g and Rearrange:
- Cancel g from both sides:
x3=4.9+0.9x3
- Rearranging gives:
x3−0.9x3=4.9
- This simplifies to:
0.1x3=4.9
- Thus:
x3=4.90.1=49
7. Calculate the Edge Length:
- Taking the cube root:
x=√(3)49≈3.65cm
Final Answer:
The minimum edge length of the block of ice required to support the metal piece without sinking is approximately 3.65 cm.
- The block of ice is floating in water and must support the weight of the metal piece.
- The buoyant force acting on the ice must equal the total weight of the ice plus the weight of the metal piece.
- The weight of the metal piece (W_m) is given by:
Wm=m⋅g=0.5kg⋅9.8m/s2=4.9N
- The weight of the ice block (W_i) can be expressed as:
Wi=density of ice⋅g⋅Vi
- The volume of the ice block (V_i) is x3 where x is the edge length of the cube.
- The buoyant force (B) acting on the ice is equal to the weight of the water displaced, which is:
B=density of water⋅g⋅Vd
- Since the volume of the ice block submerged is equal to its volume when floating, we have:
Vd=x3
- Therefore, the buoyant force can be expressed as:
B=density of water⋅g⋅x3
- At equilibrium, the buoyant force equals the total weight:
B=Wm+Wi
- Substituting the expressions we have:
density of water⋅g⋅x3=Wm+density of ice⋅g⋅x3
- The density of water is 1g/cm3 and the specific gravity of ice is 0.9, so:
density of ice=0.9g/cm3
- The equation simplifies to:
1⋅g⋅x3=4.9+0.9⋅g⋅x3
- Cancel g from both sides:
x3=4.9+0.9x3
- Rearranging gives:
x3−0.9x3=4.9
- This simplifies to:
0.1x3=4.9
- Thus:
x3=4.90.1=49
- Taking the cube root:
x=√(3)49≈3.65cm
The minimum edge length of the block of ice required to support the metal piece without sinking is approximately 3.65 cm.
Topper's Solved these Questions
Similar Questions
A cubical block of ice floating in water has to suport metal piece weighing 0.5 kg. What can be the minimum edge of the block so that it does not sink in water/ specific gravity of ice=0.9.
View SolutionA cubical box is to be constructed with iron sheets 1 mm in thickness. What can be the minimum value of the external edge so that the cube does not sink in water? Density of iron =8000kgm−3and density of water =1000kgm−3.
View SolutionA cubical block of wood weighing 200 g has a lead piece fastened underneath. Find the mass of the lead piece which will just allow the block of float in water. Specific gravity of wood is 0.8 and that of lead is 11.3.
View SolutionA cubical block of side 0.5 M floats on water with 30% of its volume under water. What is the maximum weight that can be put on the block without fully submerging it under water?
[Take density of water =103kg/m3]
View SolutionA cubical metal block of edge 12 cm floats in mercry with one fifth of the height inside the mercury. Water poured till the surface of the block is just immersed in it. Find the height of the water column to be poured. Specific gravity of mercury =13.6.
View SolutionA metal ball of specific gravity 4.5 and specific heat 0.1cal/gm−∘C is placed on a large slab of ice at 0∘C. Half of the ball sinks in the ice. The initial temperature of the ball is:- (Latent heat capacity of ice =80cal/g, specific gravity of ice =0.9)
View SolutionA block of ice of total area A and thicknes 0.5m is floating in water. In order to just support a man of nass 100kg, the area A should be (the specific gravity of ice is 0.9)
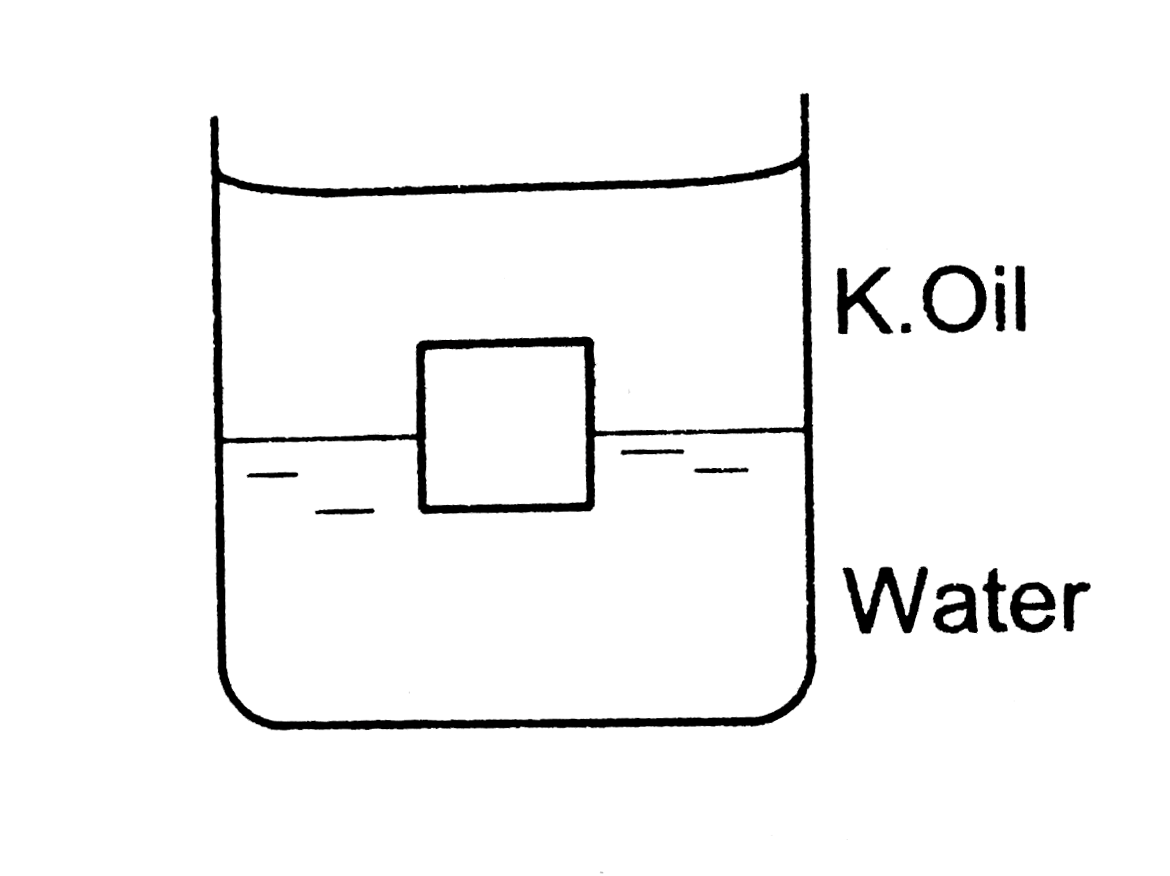
View SolutionA cube of ice floats partly in water and partly in K.oil figure. Find the ratio of the volume of ice immersed in water to that in K.oil. Specific gravity of K.oil is 0.8 and that of ice is 0.9.
View SolutionA block of ice is floating in a liquid of specific gravity 1.2 contained in the beaker. What will be the effect on the level of liquid in the beaker when the whole ice melts?
View SolutionAn ice-cube of edge is 10 cm floating in water. Time period of small vertical oscillations of the cube is: (Specific gravity of ice is 0.9)
View Solution
