The radii of curvature of the two surfaces of a lens are 20 cm and 30 cm and the refractive index of the material of the lens is 1.5. If the lens is concave -convex, then the focal length of the lens is
24 cm
10 cm
15 cm
120 cm
The correct Answer is:D
To find the focal length of a concave-convex lens with given radii of curvature and refractive index, we can use the lensmaker's formula:
1f=(μ−1)(1R1−1R2)
Where:
- f is the focal length of the lens,
- μ is the refractive index of the lens material,
- R1 is the radius of curvature of the first surface,
- R2 is the radius of curvature of the second surface.
Step 1: Identify the values given in the problem
- The radius of curvature of the first surface R1=20cm (for a convex surface, this is positive).
- The radius of curvature of the second surface R2=−30cm (for a concave surface, this is negative).
- The refractive index μ=1.5.
Step 2: Substitute the values into the lensmaker's formula
Using the lensmaker's formula, we substitute the values:
1f=(1.5−1)(120−1−30)
Step 3: Simplify the equation
Calculate μ−1:
μ−1=1.5−1=0.5
Now calculate 120−1−30:
120+130=360+260=560=112
Step 4: Substitute back into the equation
Now substitute back into the equation:
1f=0.5⋅112=0.512=124
Step 5: Calculate the focal length f
Taking the reciprocal gives us:
f=24cm
Final Result
The focal length of the lens is 24cm.
---
- f is the focal length of the lens,
- μ is the refractive index of the lens material,
- R1 is the radius of curvature of the first surface,
- R2 is the radius of curvature of the second surface.
- The radius of curvature of the first surface R1=20cm (for a convex surface, this is positive).
- The radius of curvature of the second surface R2=−30cm (for a concave surface, this is negative).
- The refractive index μ=1.5.
Using the lensmaker's formula, we substitute the values:
Calculate μ−1:
Now substitute back into the equation:
Taking the reciprocal gives us:
The focal length of the lens is 24cm.
Topper's Solved these Questions
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - EFFICIENT
49 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- REVISION TEST-15 JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-15 JEE - 2020EXERCISE - PHYSICS
25 Videos
Similar Questions
The radius of curvature of the convex face of a plano convex lens is 12cm and the refractive index of the material of the lends 1.5. Then, the focal length of the lens is
View SolutionA converging bundle of rays is intercepted by a biconcave lens. The radii of curvature of both surfaces are 20cm and the refractive index of the material of the lens is 1.5. If the rays originially converged to a point 10cm in front of the lens, where will they now converge after passing through the lens?
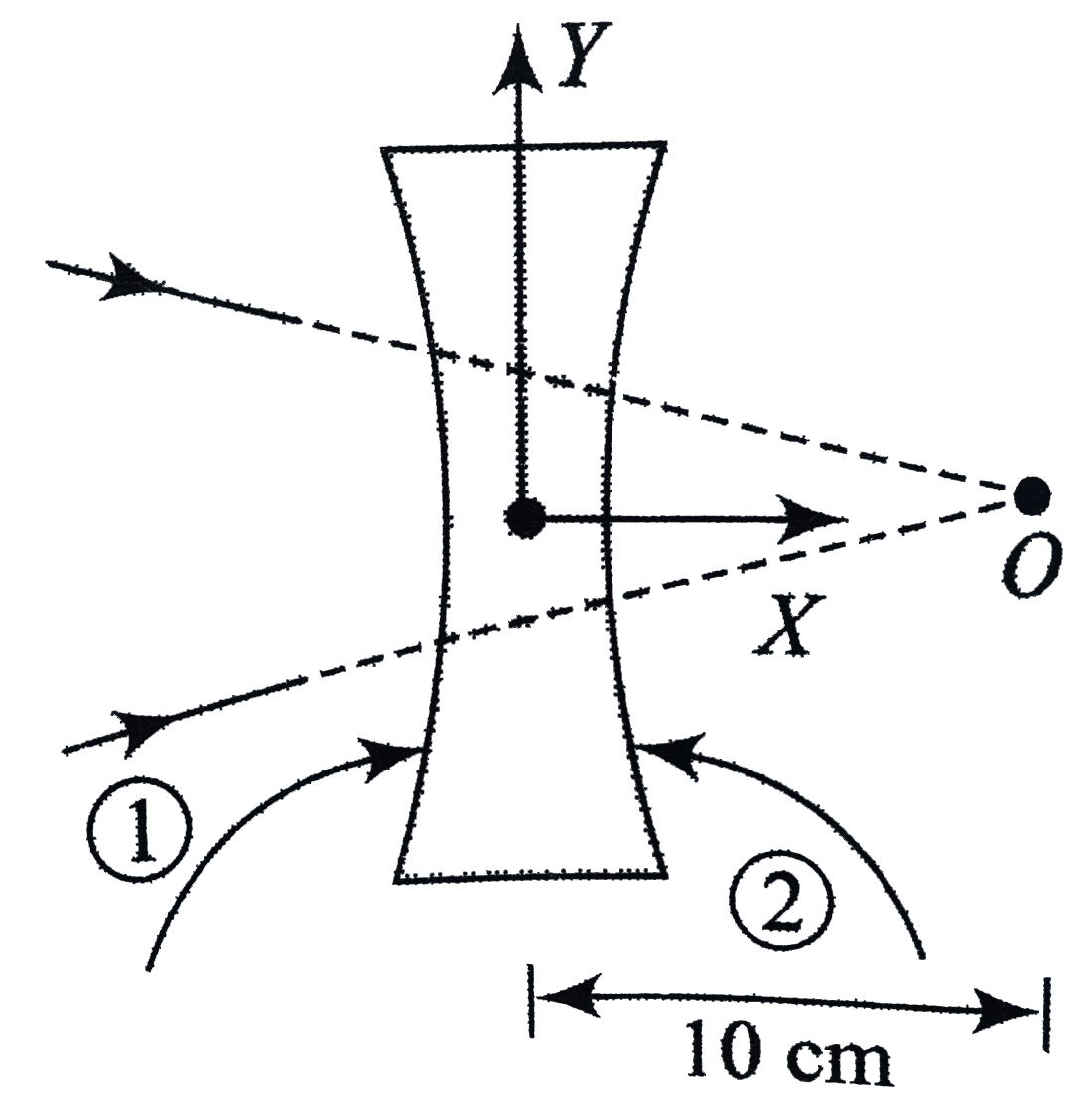
View Solution
Knowledge Check
The radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
Aact as a convex lens only for the objects that lie on its curved side.Bact as a concave lens for the objects that lie on its curved side.Cact as a convex lens irrespective of the side on which the object lies.Dact as a concave lens irrespective of side on which the object lies.
The effective focal length of the lens combination shown in figure is -60 cm. The radius of curvature of the curved surfaces of the plano-convex lenses are 12 cm each and refractive index of the material of the lens is 1.5. The refractive index of the liquid is
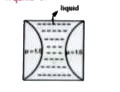
View SolutionFind the radius of curvature of the convex surface of a plan-convex lens, whose focal length is 0.3 m and the refractive index of the material of the lens is 1.5.
View SolutionA bicovex lens has radii of cuvature 20 cm each. If the refractive index of the material of the lens is 1.5, what is its focal length?
View SolutionThe radius of curvature of the convex surface of a thin plano-convex lens is 12 cm, and the refractive index of its material is 1.6. What is the power of lens ?
View SolutionThe refractive index of a material of a plano-concave lens is 53, the radius of curvature is 0.3 m. The focal length of the lens in air is
View SolutionThe radii of curvatures of a convex lens are 0.04 m and 0.04m. Its refractive index is 1.5. Its focal length is
View SolutionIf in a planoconvex lens, the radius of curvature of the convex surface is 10cm and the focal length is 30cm, the refractive index of the material of the lens will be
View Solution
