The left end of a copper rod (length=20cm, Area of cross section=0.2cm2) is maintained at 20∘C and the right end is maintained at 80∘C . Neglecting any loss of heat through radiation, find (a) the temperature at a point 11cm from the left end and (b) the heat current through the rod. thermal conductivity of copper =385Wm−1C−1 .
The correct Answer is:A, B, C
To solve the problem, we will break it down into two parts: (a) finding the temperature at a point 11 cm from the left end of the rod, and (b) calculating the heat current through the rod.
Given Data:
- Length of the rod, L=20cm=0.2m
- Area of cross-section, A=0.2cm2=0.2×10−4m2=2×10−5m2
- Temperature at the left end, TL=20∘C
- Temperature at the right end, TR=80∘C
- Thermal conductivity of copper, k=385W/m⋅C
Part (a): Finding the Temperature at a Point 11 cm from the Left End
1. Identify the position of the point:
- The point is located at x=11cm=0.11m from the left end.
2. Use the formula for temperature distribution:
The temperature T(x) at a distance x from the left end can be found using the linear temperature gradient:
T(x)=TL+(TR−TLL)x
Substituting the known values:
T(0.11)=20+(80−200.2)⋅0.11
T(0.11)=20+(600.2)⋅0.11
T(0.11)=20+300⋅0.11
T(0.11)=20+33=53∘C
Temperature at a point 11 cm from the left end is 53∘C.
Part (b): Calculating the Heat Current through the Rod
1. Use the formula for heat current:
The heat current I can be calculated using the formula:
I=k⋅A⋅(TR−TL)L
Substituting the known values:
I=385⋅(2×10−5)⋅(80−20)0.2
I=385⋅(2×10−5)⋅600.2
I=385⋅1.2×10−30.2
I=0.4620.2=2.31W
The heat current through the rod is 2.31W.
Summary of Answers:
- (a) Temperature at a point 11 cm from the left end: 53∘C
- (b) Heat current through the rod: 2.31W
- Length of the rod, L=20cm=0.2m
- Area of cross-section, A=0.2cm2=0.2×10−4m2=2×10−5m2
- Temperature at the left end, TL=20∘C
- Temperature at the right end, TR=80∘C
- Thermal conductivity of copper, k=385W/m⋅C
- The point is located at x=11cm=0.11m from the left end.
The temperature T(x) at a distance x from the left end can be found using the linear temperature gradient:
T(x)=TL+(TR−TLL)x
Substituting the known values:
T(0.11)=20+(80−200.2)⋅0.11
T(0.11)=20+(600.2)⋅0.11
T(0.11)=20+300⋅0.11
T(0.11)=20+33=53∘C
The heat current I can be calculated using the formula:
I=k⋅A⋅(TR−TL)L
Substituting the known values:
I=385⋅(2×10−5)⋅(80−20)0.2
I=385⋅(2×10−5)⋅600.2
I=385⋅1.2×10−30.2
I=0.4620.2=2.31W
- (a) Temperature at a point 11 cm from the left end: 53∘C
- (b) Heat current through the rod: 2.31W
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER11videos
HEAT TRANSFER
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE II6videos
HEAT TRANSFER
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 26videos
HEAT AND TEMPERATURE
- BOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer4videos
INTRODUCTION TO PHYSICS
Similar Questions
One face of a copper cube of edge 10 cm is maintained at 100∘C and the opposite face is maintained at 0∘C. All other surfaces are covered with an insulating material. Find the amount of heat flowing per second through the cube. Thermal conductivity of copper is 385Wm−1C−1.
View SolutionFigure shows an aluminium rod joined to a copper rod. Each of the rods has a length of 20cm and area of cross section 0.20cm2 . The junction is maintained at a constant temperature 40∘C and the two ends are maintained at 80∘C . Calculate the amount of heat taken out from the cold junction in one minute after the steady state is reached. The conductivities are KAl=200Wm−1C−1 . and KCu=400Wm−1C−1 .
 View Solution
View SolutionA steel bar 10.0 cm long is welded end to end to a copper bar 20.0 cm long. Both bars are insulated perfectly on their sides . Each bar has a separate cross-section, 2.00 cm on a side . The free end of the steel bar is maintained at 100∘C by placing it in contact with steam and free end of the copper bar is maintained at 0∘C by placing it in contact with ice . find the temperature at the junction of the two bars and the total rate of heat flow . thermal conductivity of steel =50.2Wm−1K−1.thermal conductivity of copper =385Wm−1K−1.
View SolutionThe ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK
 View Solution
View SolutionTwo ends of a conducting rod of varying cross-section are maintained at 200∘C and 0∘C respectively. In steady state:
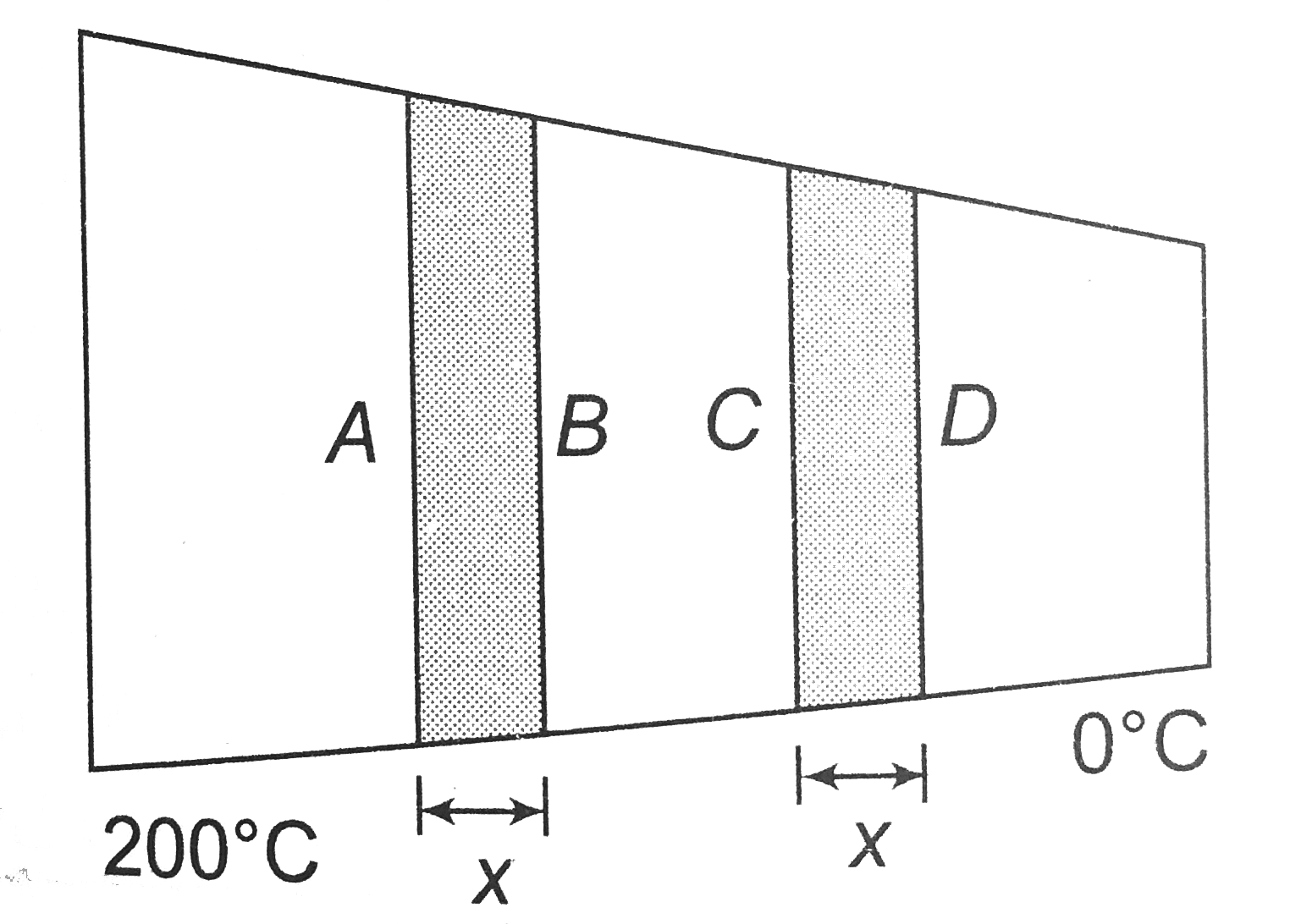 View Solution
View SolutionOne end of a copper rod of length 1 m and area of cross - section 400×(10−4)m2 is maintained at 100∘C. At the other end of the rod ice is kept at 0∘C. Neglecting the loss of heat from the surrounding, find the mass of ice melted in 1h. Given, KCu=401W/m−KandLf=3.35×(105)J/kg.
View SolutionFind the thermal resistance of an aluminium rod of length 20cm and area of cross section 1cm2 . The heat current is along the length of the rod. Thermal conductivity of aluminium =200Wm−1K−1 .
View SolutionTwo parallel plates A and B are joined together to form a compound plate . The thicknesses of the plate are 4.0cm and 2.5cm respectively and the area of cross section is 100cm2 for each plate. The thermal conductivities are KA=200Wm−1C−1 for plate A and KB=400Wm−1C−1 for the plate B. The outer surface of the plate A is maintained at100∘C and the outer surface of the plate B is maintained at 0∘C. Find (a) the rate of heat flow through any cross section, (b) the temperature at the interface and (c) the equivalent thermal conductivity of the compound plate.
 View Solution
View SolutionFigure shows a steel rod joined to a brass rod. Each of the rods has length of 31cm and area of cross-section 0.20cm2. The junction is maintained at a constant temperature 50∘C and the two ends are maintained at 100∘C. The amount of heat taken out from the cold junction in 10 minutes after the steady state is reached in n×102J. Find 'n' the thermal conductivities are Ksteel=46W/m−∘C and Kbrass=109W/m−∘C.
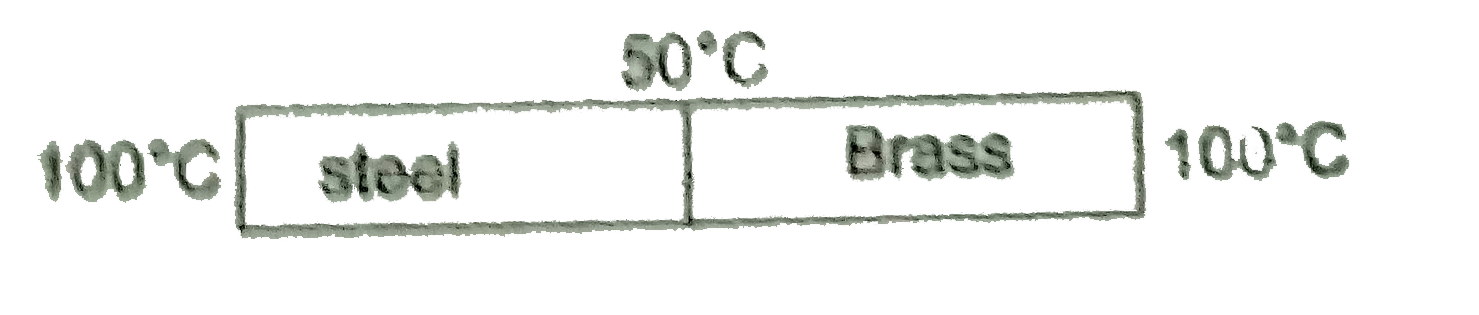 View Solution
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View Solution

