Two conducting spheres of radiiR1andR2 are kept widely separated from each other . What are their individual capacitances?
To find the individual capacitances of two widely separated conducting spheres with radii R1 and R2, we can follow these steps:
Step 1: Understand the Concept of Capacitance for a Sphere
The capacitance C of a conducting sphere is given by the formula:
C=4πϵ0R
where R is the radius of the sphere and ϵ0 is the permittivity of free space.
Step 2: Apply the Formula for Each Sphere
Since the spheres are widely separated, we can treat them as isolated capacitors. Therefore, we can calculate the capacitance for each sphere individually.
1. For the first sphere with radius R1:
C1=4πϵ0R1
2. For the second sphere with radius R2:
C2=4πϵ0R2
Step 3: Write the Final Answers
Thus, the individual capacitances of the two spheres are:
- The capacitance of the first sphere is:
C1=4πϵ0R1
- The capacitance of the second sphere is:
C2=4πϵ0R2
Summary
The individual capacitances of the two conducting spheres are:
- C1=4πϵ0R1
- C2=4πϵ0R2
The capacitance C of a conducting sphere is given by the formula:
C=4πϵ0R
where R is the radius of the sphere and ϵ0 is the permittivity of free space.
Since the spheres are widely separated, we can treat them as isolated capacitors. Therefore, we can calculate the capacitance for each sphere individually.
C1=4πϵ0R1
C2=4πϵ0R2
Thus, the individual capacitances of the two spheres are:
- The capacitance of the first sphere is:
C1=4πϵ0R1
- The capacitance of the second sphere is:
C2=4πϵ0R2
The individual capacitances of the two conducting spheres are:
- C1=4πϵ0R1
- C2=4πϵ0R2
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - short answer7videos
CAPACITORS
- BOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE 26videos
CAPACITORS
- BOOK - HC VERMA ENGLISHCHAPTER - BOHR'S MODEL AND PHYSICS OF THE ATOMEXERCISE - Short Answer10videos
BOHR'S MODEL AND PHYSICS OF THE ATOM
- BOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Question for short Answer6videos
DISPERSION AND SPECTRA
Similar Questions
Three plates A, B and C are placed close to each other with +Q charge given to the middle plate. The inner surfaces to A and C can be connected to earth through plate D and keys K1 and K2 . The plates D is a dielectric slab with dielectric constant K1 then the charge that will flow through plate D and keys K1 and K2 . The plate D is a dielectric slab with dielectric constant K, then the charge that will flow though plate D when K2 is closed and K2 is open is
 View Solution
View SolutionTwo conducting spheres of radii r and 2r are placed at very large separation. Each sphere possesses charge Q. These spheres are connected with a conducting wire of resistance R. Then, which of the following is true?
View SolutionThere are two concentric conducting shells of radius R,2R respectively. Charge on each surface is as shown in the figure.
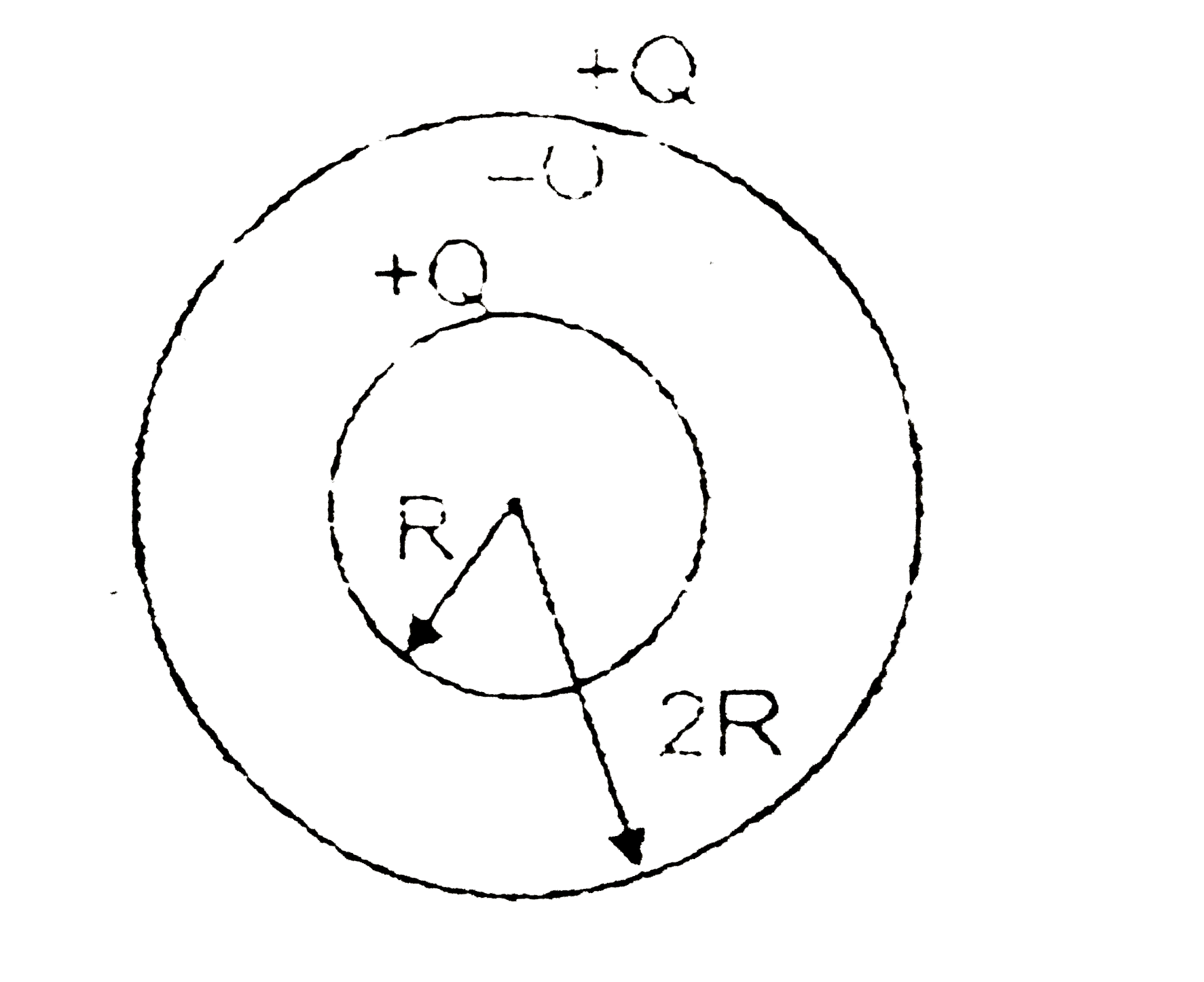
Equivalent capacitance of this system is:View SolutionTwo metallic spheres of radii a and b are placed faraway from each other and are connected for a thin conducting wire. A charge Q is given to one of the spheres . Calculate the charge on each sphere at electrostatic equilibrium.
View SolutionTwo similar conducting balls having charges + q and - q are placed at a separation d from each other in air. The radius of each ball is r and the separation between their centres is d (d >> r ). Calculate the capacitance of the two ball system
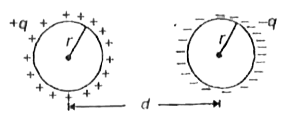 View Solution
View SolutionTwo spherical conductors of radii R1andR2 are separated by a distance much larger than the radius of eighter sphere. The spheres are connected by a conducting wire as shown in (Fig. 3.128). If the charges on the spheres in equilibrium are q1andq2, respectively, what is the ratio of the field strength at the surfaces of the spheres ?
 .View Solution
.View SolutionThe figure shows a system of two concentric spheres of radii r1andr2 are kept at temperature T1andT2, respectively. The radial rate of flow of heat in a substance between the two concentric spheres is proportional to
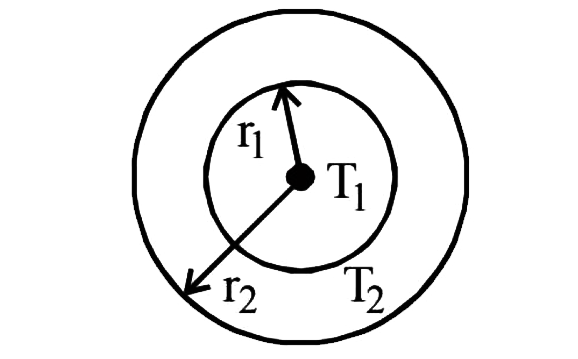 View Solution
View SolutionConsider two conducting spheres of radii R1andR2 with R1>R2. If the two are at the same potential, the larger sphere has more charge than the smaller sphere. State whetehr the charge density of the smaller sphere is more or less than that of the larger oe.
View SolutionTwo conducting spheres of radii 3 cm and 1 m are separated by a distance of 10 cm in free space. If the spheres are charged to same potential of 10 V each, then the force of repulsion between them is
View SolutionTwo concentric hollow conducting spheres of radius r and R are shown. The charge on outer shell is Q. what charge should be given to inner sphere so that the potential at any point P outside the outer sphere is zero ?
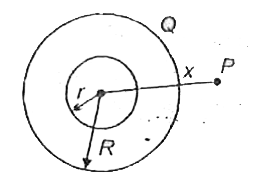 View Solution
View Solution

