A straight of length L extends from x=a to x=L+a. the gravitational force it exerts on a point mass 'm' at x=0, if the mass per unit length of the rod is is given by :
To find the gravitational force exerted by a straight rod of length L with mass per unit length given by λ(x)=A+Bx2 on a point mass m located at x=0, we can follow these steps:
Step 1: Define the mass element
The mass per unit length of the rod is given by λ(x)=A+Bx2. To find the mass of a small segment dx of the rod, we can express it as:
dm=λ(x)dx=(A+Bx2)dx
Step 2: Determine the force due to the mass element
The gravitational force dF exerted by the mass element dm on the point mass m located at x=0 is given by Newton's law of gravitation:
dF=Gmdmx2
Substituting dm from step 1:
dF=Gm(A+Bx2)dxx2
Step 3: Set up the integral for total force
The rod extends from x=a to x=L+a. Therefore, we need to integrate dF from x=a to x=L+a:
F=∫L+aadF=∫L+aaGm(A+Bx2)x2dx
Step 4: Split the integral
We can split the integral into two parts:
F=Gm(∫L+aaAx2dx+∫L+aaBx2x2dx)
This simplifies to:
F=Gm(A∫L+aa1x2dx+B∫L+aa1dx)
Step 5: Evaluate the integrals
1. The first integral:
∫1x2dx=−1x
Thus,
∫L+aa1x2dx=−1L+a+1a=1a−1L+a
2. The second integral:
∫1dx=x
Thus,
∫L+aa1dx=(L+a)−a=L
Step 6: Substitute back into the force equation
Now substituting back into the force equation:
F=Gm(A(1a−1L+a)+B⋅L)
Final Result
Thus, the total gravitational force exerted by the rod on the point mass m is:
F=Gm(A(1a−1L+a)+BL)
The mass per unit length of the rod is given by λ(x)=A+Bx2. To find the mass of a small segment dx of the rod, we can express it as:
dm=λ(x)dx=(A+Bx2)dx
The gravitational force dF exerted by the mass element dm on the point mass m located at x=0 is given by Newton's law of gravitation:
dF=Gmdmx2
Substituting dm from step 1:
dF=Gm(A+Bx2)dxx2
The rod extends from x=a to x=L+a. Therefore, we need to integrate dF from x=a to x=L+a:
F=∫L+aadF=∫L+aaGm(A+Bx2)x2dx
We can split the integral into two parts:
F=Gm(∫L+aaAx2dx+∫L+aaBx2x2dx)
This simplifies to:
F=Gm(A∫L+aa1x2dx+B∫L+aa1dx)
1. The first integral:
∫1x2dx=−1x
Thus,
∫L+aa1x2dx=−1L+a+1a=1a−1L+a
∫1dx=x
Thus,
∫L+aa1dx=(L+a)−a=L
Now substituting back into the force equation:
F=Gm(A(1a−1L+a)+B⋅L)
Thus, the total gravitational force exerted by the rod on the point mass m is:
F=Gm(A(1a−1L+a)+BL)
Topper's Solved these Questions
Similar Questions
A straight rod of length L has one of its end at the origin and the other at X=L. If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
View SolutionA straight rod of length L has one of its end at the origin and the other at X=L. If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
View SolutionA straight rod of length L has one of its end at the origin and the other at X=L. If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
View SolutionA straight rod of length 'l' extends from x=l to x=2l. If linear mass density of rod is μ=(μ0l3X3), then the gravitational force exerted by rod on a particle of mass m at x=0 , is
View SolutionFind the potential energy of the gravitational interation of a point mass of mass m and a thin uniform rod of mass M and length l, if they are located along a straight line such that the point mass is at a distance 'a' from one end. Also find the force of their interaction.
View SolutionCalculate the force exerted by point mass m on rod of uniformly distributed mass M and length l (Placed as shown in figure)
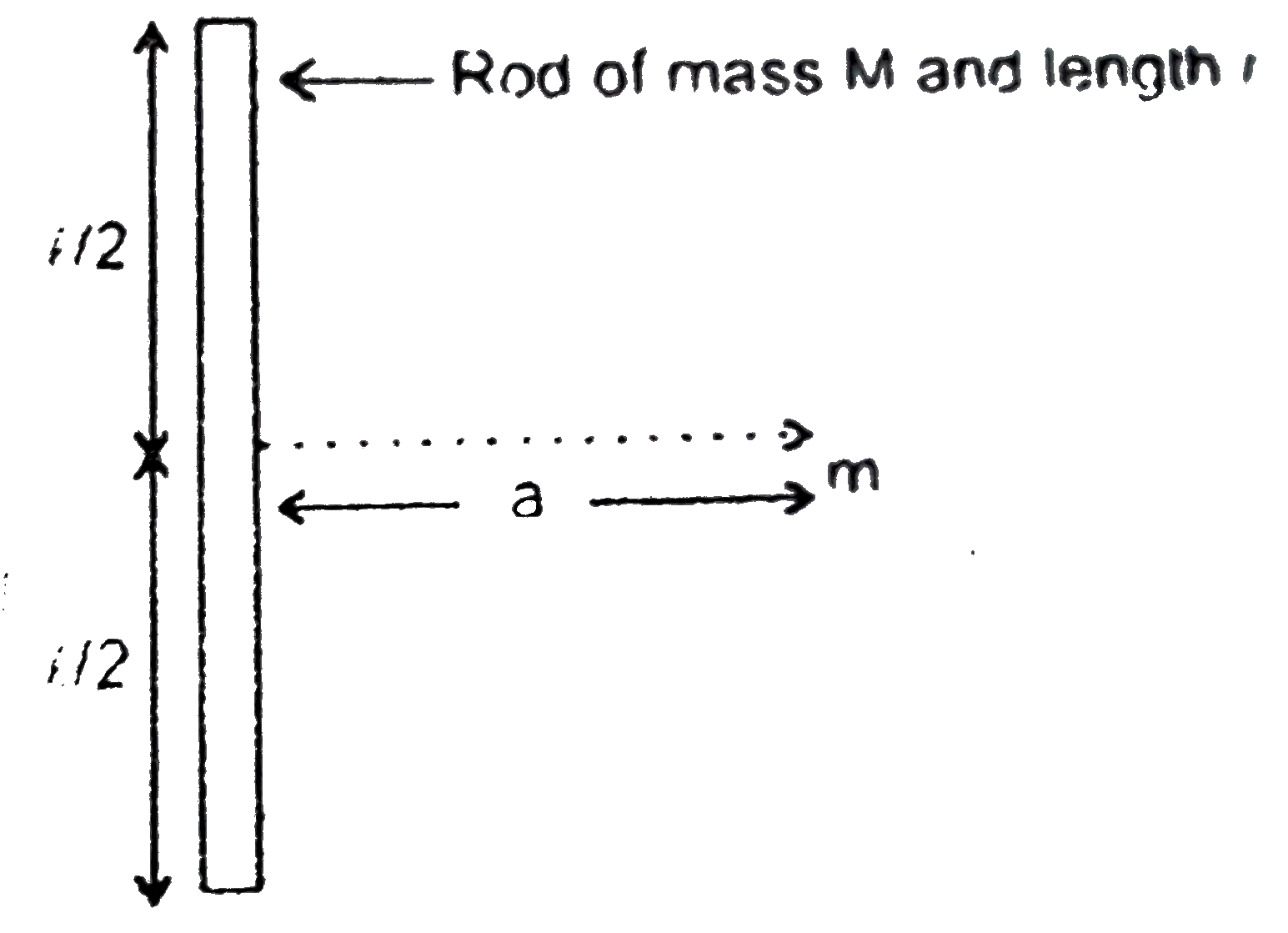 .
.
View SolutionA mass m is at a distance a from one end of a uniform rod of length l and mass M. Find the gravitational force on the mass due to the rod.

View SolutionA thin bar of length L has a mass per unit length λ, that increases linerarly with distance from one end. If its total mass is M and its mass per unit length at the lighter end is λ0, then the distance of the centre of mass from the lighter end is
View SolutionA thin of length L is bent to form a semicircle. The mass of rod is M. What will be the gravitational potential at the centre of the circle ?
View SolutionCalculate the potential due to a thin charged rod of length L at the points along and perpendicular to the length. Charge per unit length on the rod is λ.
View Solution
