Effective capacitance of parallel combination of two capacitors C1andC2 is 10μF. When the capacitors are individually connected to a voltage source of 1V, the energy stored in the capacitor C2 is 4 times of C1. If these capacitors are connected in series, their effective capacitor will be: lt
Option1 3.2μF
Option2 4.2μF
Option3 1.6μF
Option4 8.4μF
The correct Answer is:3
Step by step video & image solution for Effective capacitance of parallel combination of two capacitors C_(1) and C_(2) is 10muF. When the capacitors are individually connected to a voltage source of 1V, the energy stored in the capacitor C_(2) is 4 times of C_(1). If these capacitors are connected in series, their effective capacitor will be: lt by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
If there are n capacitors in parallel connected to V volt source, then the energy stored is equal to
View SolutionThree capacitors are connected as shwn in. Then, the charge on capacitor C1 is .
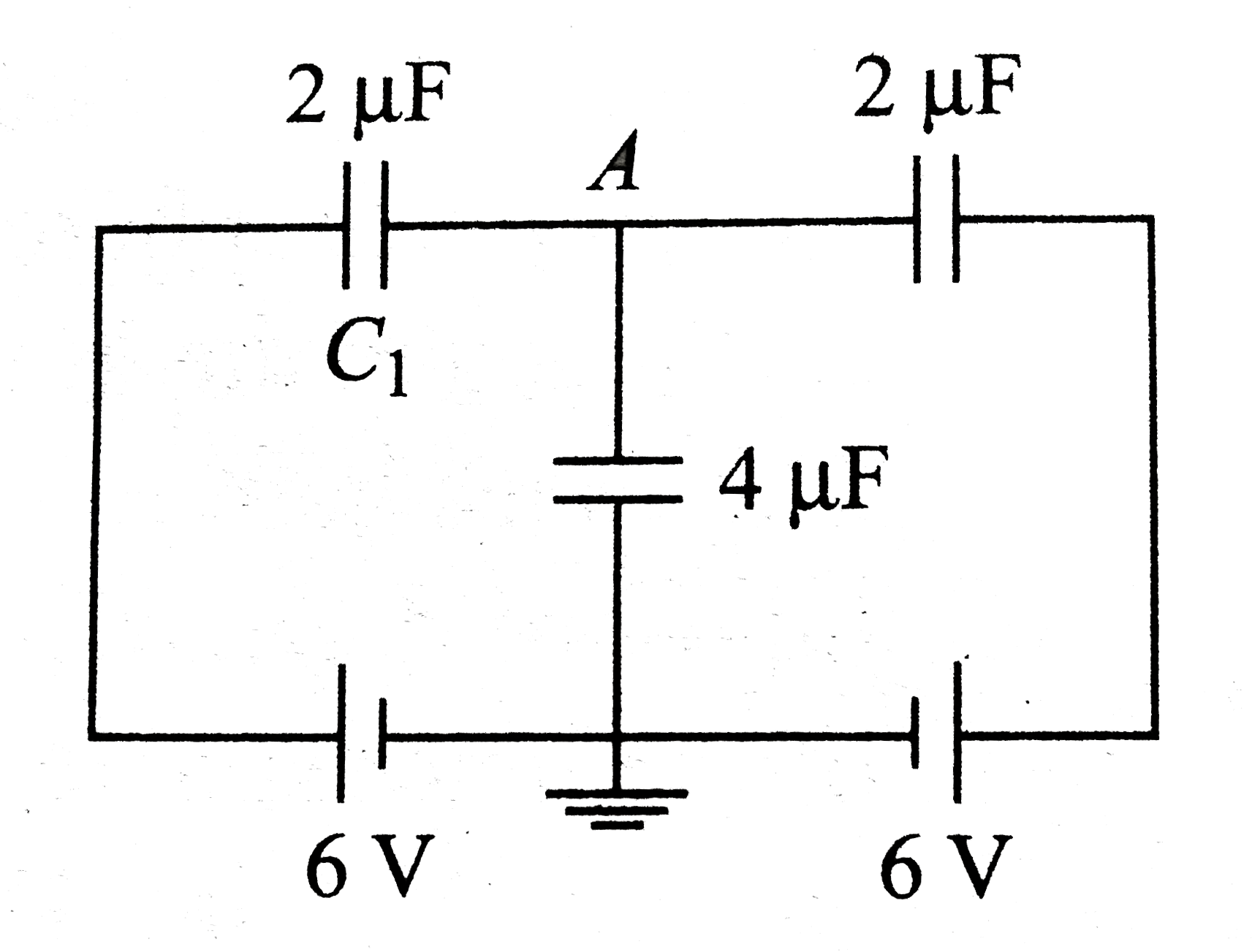. View SolutionA capacitor of capacitance 6 μF is connected with an emf sourface 3 V. Electrostaci energy stored in the capacitor is
View SolutionTwo capacitors C1 and C2 of capacitances C2 and C4 are connected to a V volt battery, as shown in figure. The ratio of energy slored in capacitors C1 and C2 is
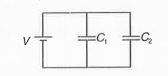
View SolutionA 10V battery is connected to two capacitors C1 and C2 and a resistor R. When the capacitors are fully charged, then the potential difference across the resistor will be
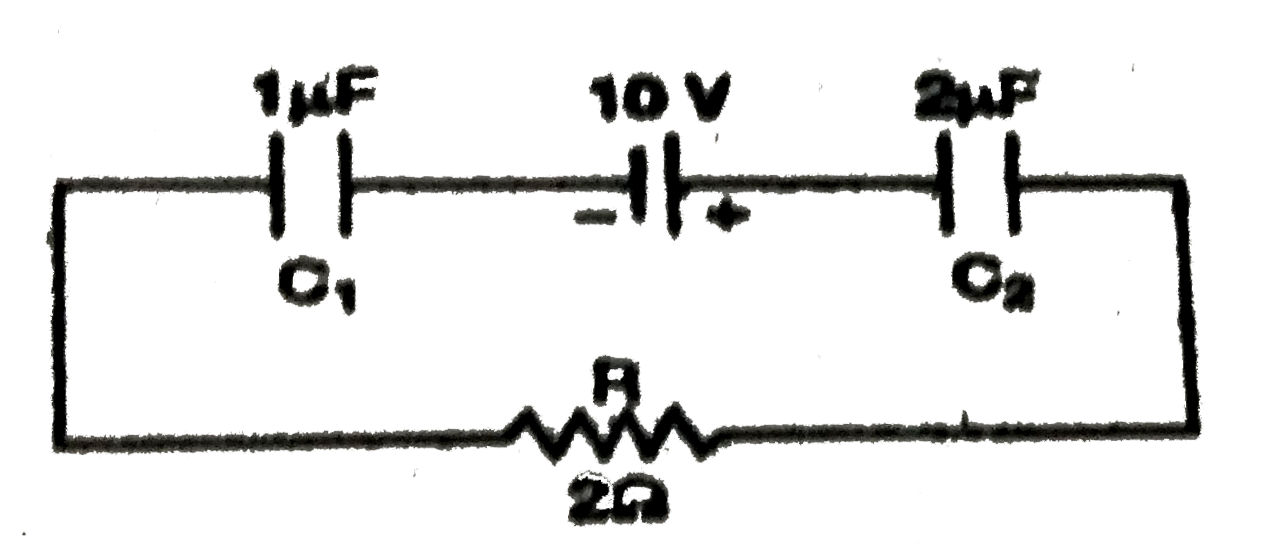
View SolutionIf two capacitors C1 & C2 are connected in parallel then equivalent capacitance is 10F. If both capacitance are connected across 1V battery then energy stored by C2 is 4 times of C1. Then the equivalent capacitance if they are connected in series is-
View SolutionObtain an expression for equivalent capacitance when three capacitors C1,C2andC3 are connected in series.
View SolutionTwo capacitors of 10μF and 20μF are connected in series with a 30V battery. The charge on the capacitors will be, respectively
View SolutionA capacitor, a resistor and a 40 mH inductor are connected in series to an a.c. source of frequency 60 Hz. Calculate the capacitance of the capacitor,if current is in phase with the voltage.
View SolutionA 2μF capacitor C1 is charge to a voltage 100 V and a 4μF capacitor C2 is charged to a voltage 50 V. The capacitors are then connected in parallel What is the loss of energy due to parallel connection?
View Solution