A star of mass m revolves in radius R in galaxy in which density varies with distance r as ρ=kr, k=constant. Find the relation between time period and radius R
T×R32 is constant
T×R−12 is constant
T×R72 is constant
T×R is constant
The correct Answer is:B
To find the relationship between the time period T and the radius R of a star revolving in a galaxy where the density varies as ρ=kr, we can follow these steps:
Step 1: Write down the gravitational force
The gravitational force acting on the star of mass m due to the mass M enclosed within radius R is given by:
Fgravity=GmMR2
where G is the gravitational constant.
Step 2: Express the centripetal force
The centripetal force required to keep the star in circular motion is given by:
Fcentripetal=mω2R
where ω is the angular velocity.
Step 3: Relate angular velocity to the time period
The angular velocity ω can be expressed in terms of the time period T:
ω=2πT
Thus, the centripetal force can also be written as:
Fcentripetal=m(2πT)2R=4π2mRT2
Step 4: Set the gravitational force equal to the centripetal force
Since the gravitational force provides the necessary centripetal force, we set them equal:
GmMR2=4π2mRT2
We can cancel m from both sides (assuming m≠0):
GMR2=4π2RT2
Step 5: Find the expression for mass M
To find M, we need to integrate the density function ρ=kr over the volume of the sphere of radius R:
M=∫R0ρdV=∫R0kr⋅4πr2dr
Calculating the volume element dV=4πr2dr:
M=4πk∫R0rdr=4πk(r22)R0=4πk⋅R22=2πkR2
Step 6: Substitute M back into the force equation
Substituting M into the equation we derived from the force balance:
G(2πkR2)R2=4π2RT2
This simplifies to:
2πGk=4π2RT2
Step 7: Rearranging for the time period T
Rearranging gives:
T2=4π2R2πGk=2πRGk
Taking the square root, we find:
T=√2πRGk
Final Relation
Thus, the relationship between the time period T and the radius R is:
T∝R1/2
The gravitational force acting on the star of mass m due to the mass M enclosed within radius R is given by:
Fgravity=GmMR2
where G is the gravitational constant.
The centripetal force required to keep the star in circular motion is given by:
Fcentripetal=mω2R
where ω is the angular velocity.
The angular velocity ω can be expressed in terms of the time period T:
ω=2πT
Thus, the centripetal force can also be written as:
Fcentripetal=m(2πT)2R=4π2mRT2
Since the gravitational force provides the necessary centripetal force, we set them equal:
GmMR2=4π2mRT2
We can cancel m from both sides (assuming m≠0):
GMR2=4π2RT2
To find M, we need to integrate the density function ρ=kr over the volume of the sphere of radius R:
M=∫R0ρdV=∫R0kr⋅4πr2dr
Calculating the volume element dV=4πr2dr:
M=4πk∫R0rdr=4πk(r22)R0=4πk⋅R22=2πkR2
Substituting M into the equation we derived from the force balance:
G(2πkR2)R2=4π2RT2
This simplifies to:
2πGk=4π2RT2
Rearranging gives:
T2=4π2R2πGk=2πRGk
Taking the square root, we find:
T=√2πRGk
Thus, the relationship between the time period T and the radius R is:
T∝R1/2
Topper's Solved these Questions
Similar Questions
An infinite cylindrical wire of radius R and having current density varying with its radius r as, J=J0[1−(r/R)]. Then answer the following questions.
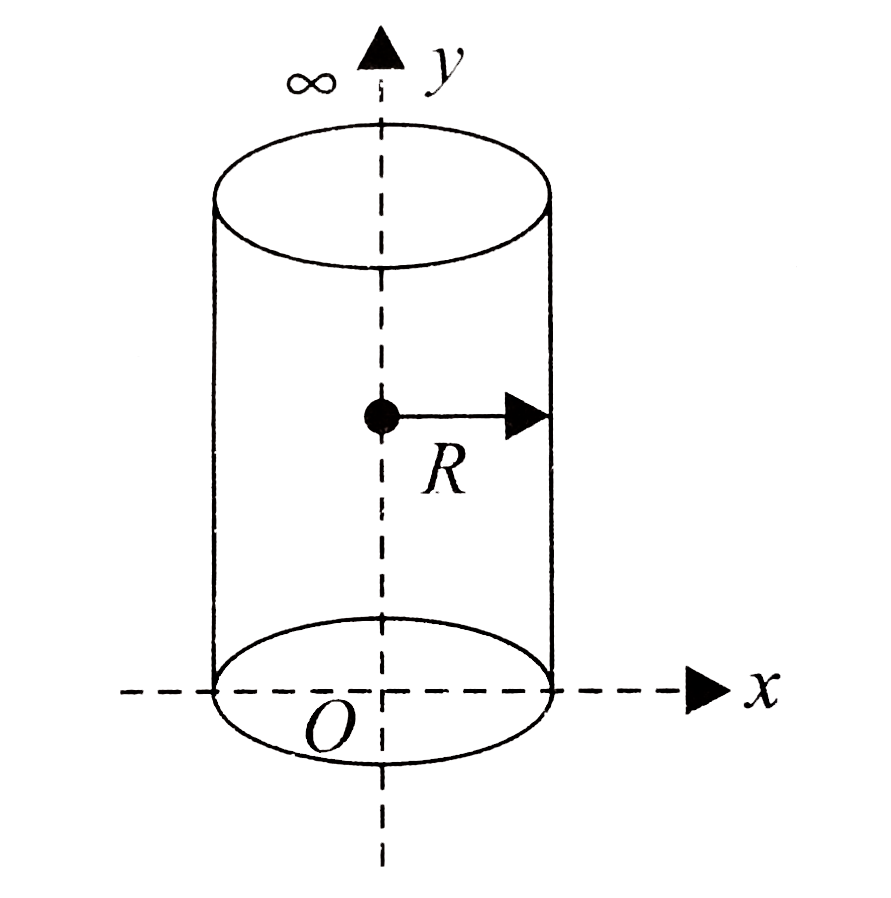
Graph between the magnetic field and radius is
View SolutionThe current density in a wire of radius a varies with radial distance r as J=J0r2a, where J0 is a constant. Choose the incorrect statement.
View SolutionA planet of mass m moves around the Sun of mass Min an elliptical orbit. The maximum and minimum distance of the planet from the Sun are r1 and r2, respectively. Find the relation between the time period of the planet in terms of r1 and r2.
View SolutionA particle of mass m is revolving in a horizontal circle of radius r under a centripetal force k/r2 where k is a constant. What is the total energy of the particle ?
View SolutionThe period T0 for a planet of mass 'm' above the sum in a circular orbit of radius R depends on m,R and G where G is the gravitational constant. Find expression for time period by dimensional methods.
View SolutionA long cylindrical conductor of radius R carries a current i as shown in the figure. The current density J varies across the cross-section as J=kr2, where, k is a constant. Find an expression for the magnetic field B at a distance r(<R) from the axis

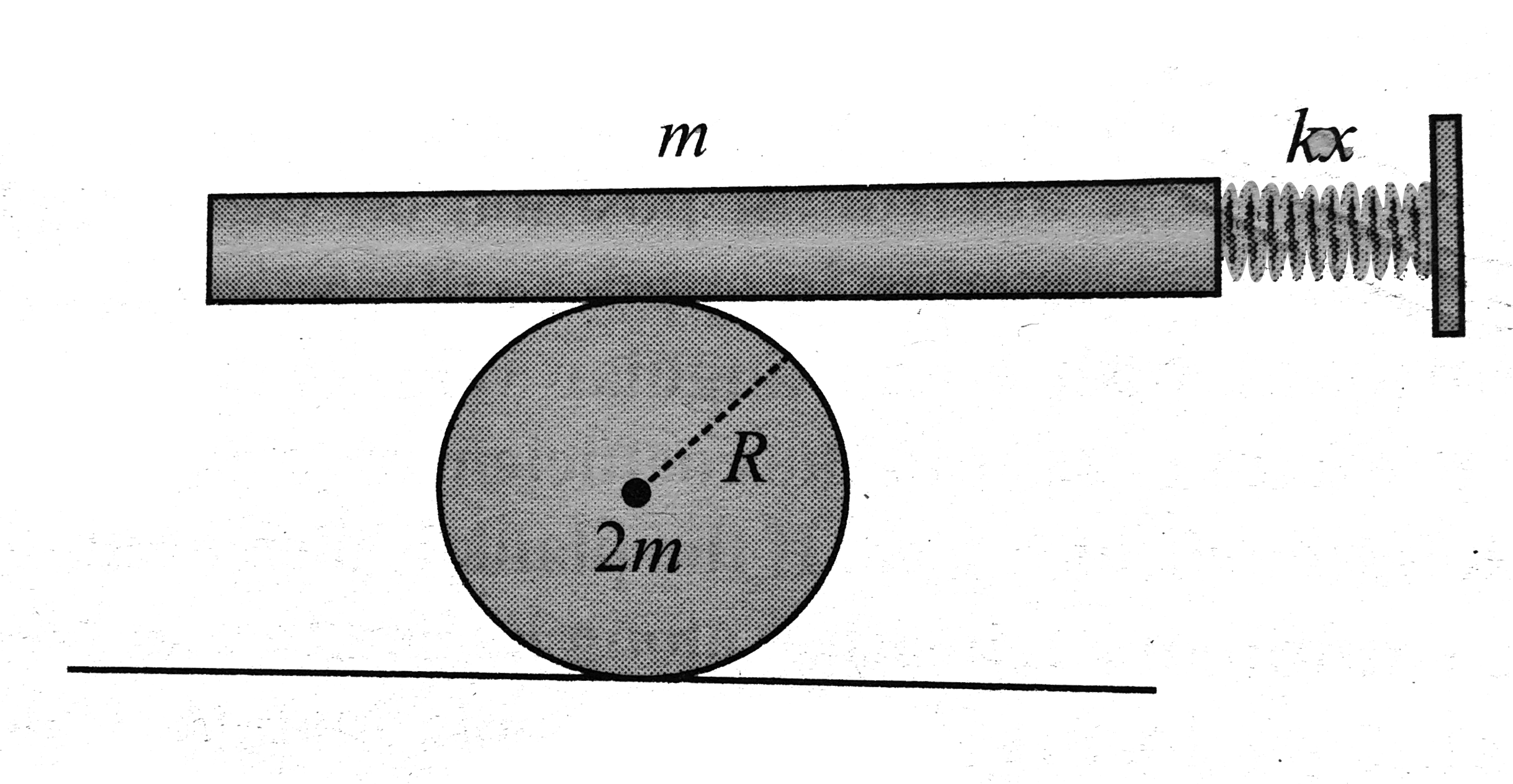
View SolutionA uniform plank of mass m, free to move in the horizontal direction only , is placed at the top of a solid cylinder of mass 2m and radius R. The plank is attached to a fixed wall by mean of a light spring constant k. There is no slipping between the cylinder and the planck , and between the cylinder and the ground. Find the time period of small oscillation of the system.
View SolutionIf the radius of a tetrahedral void is 'r' and radius of atom in close packing is 'R', derive the relation between r and R.
View SolutionIf radius of an octahedral void is r and atomic radius of atoms assuming cubical close pacting is R. Then the relation between r and R is
View SolutionThe small dense stars rotate about their common centre of mass as a binary system, each with a period of 1 year. One star has mass double than that of the other, while mass of the lighter star is one-third the mass of the Sun. The distance between the two stars is r and the distance of the earth from the Sun is R, find the relation between r and R.
View Solution