A block of mass m attached to a massless spring is performing oscillatory motion of amplitude A on a frictionless horizontal plane. If half of the mass of the block off when it is passing through its mean position, the amplitude of oscillation for the remaining system become fA. The value of f is .
√2
1√2
12
1
To solve the problem, we will follow these steps:
Step 1: Understand the initial conditions
A block of mass m is attached to a massless spring and is performing oscillatory motion with an amplitude A. At the mean position, the total energy of the system is purely kinetic.
Step 2: Write the expression for total energy
The total mechanical energy E of the system when the block is at the mean position can be expressed as:
E=12kA2
where k is the spring constant.
Step 3: Analyze the situation when half the mass is removed
When half of the mass is removed while the block is passing through the mean position, the new mass becomes m2. Let the new velocity at the mean position be v′.
Step 4: Apply conservation of momentum
Using conservation of momentum at the moment the mass is halved:
mv=m2v′
From this, we can solve for v′:
v′=2v
Step 5: Write the expression for kinetic energy after mass is halved
The kinetic energy at the mean position after the mass is halved is:
E′=12(m2)(v′)2=12(m2)(2v)2=12(m2)(4v2)=2mv22=mv2
Step 6: Relate the new kinetic energy to potential energy at the extreme position
At the extreme position, all the kinetic energy converts to potential energy:
E′=12kA′2
Equating the two expressions for energy:
mv2=12kA′2
Step 7: Relate the new kinetic energy to the initial total energy
From the initial energy expression:
12kA2=12mv2
Thus, we can express mv2 in terms of A:
mv2=12kA2
Step 8: Substitute into the energy equation
Now substituting mv2 into the equation for E′:
12kA2=12kA′2
This simplifies to:
A′2=2A2
Taking the square root gives:
A′=√2A
Step 9: Find the value of f
From the problem statement, we have A′=fA. Thus:
f=√2
Final Answer
The value of f is √2.
---
A block of mass m is attached to a massless spring and is performing oscillatory motion with an amplitude A. At the mean position, the total energy of the system is purely kinetic.
The total mechanical energy E of the system when the block is at the mean position can be expressed as:
E=12kA2
where k is the spring constant.
When half of the mass is removed while the block is passing through the mean position, the new mass becomes m2. Let the new velocity at the mean position be v′.
Using conservation of momentum at the moment the mass is halved:
mv=m2v′
From this, we can solve for v′:
v′=2v
The kinetic energy at the mean position after the mass is halved is:
E′=12(m2)(v′)2=12(m2)(2v)2=12(m2)(4v2)=2mv22=mv2
At the extreme position, all the kinetic energy converts to potential energy:
E′=12kA′2
Equating the two expressions for energy:
mv2=12kA′2
From the initial energy expression:
12kA2=12mv2
Thus, we can express mv2 in terms of A:
mv2=12kA2
Now substituting mv2 into the equation for E′:
12kA2=12kA′2
This simplifies to:
A′2=2A2
Taking the square root gives:
A′=√2A
From the problem statement, we have A′=fA. Thus:
f=√2
The value of f is √2.
Topper's Solved these Questions
Similar Questions

A block of mass m is attached to two unstretched springs of spring constant k, each as shown. The block is displaced towards right through a distance x and is released The speed of the block as it passes through the mean position will be
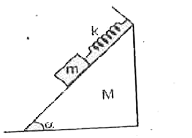
View SolutionA system consisting of a smooth movable wedge of angle α and a block A of mass m are connected together with a massless spring of spring constant k, as shown in the figure. The system is kept on a frictionless horizontal plane. If the block is displaced slighlly from equilibrium and left to oscillate, find the frequency of small oscillations.
View SolutionTwo blocks of masses m1=1kg and m2 = 2kg are connected by a spring of spring constant k = 24 N/m and placed on a frictionless horizontal surface. The block m1 is imparted an initial velocity v0 = 12cm/s to the right. The amplitude of oscillation is
View SolutionA block of mass m is attached to two unstretched springs of spring constants k1andk2 as shown in figure. The block is displaced towards right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.

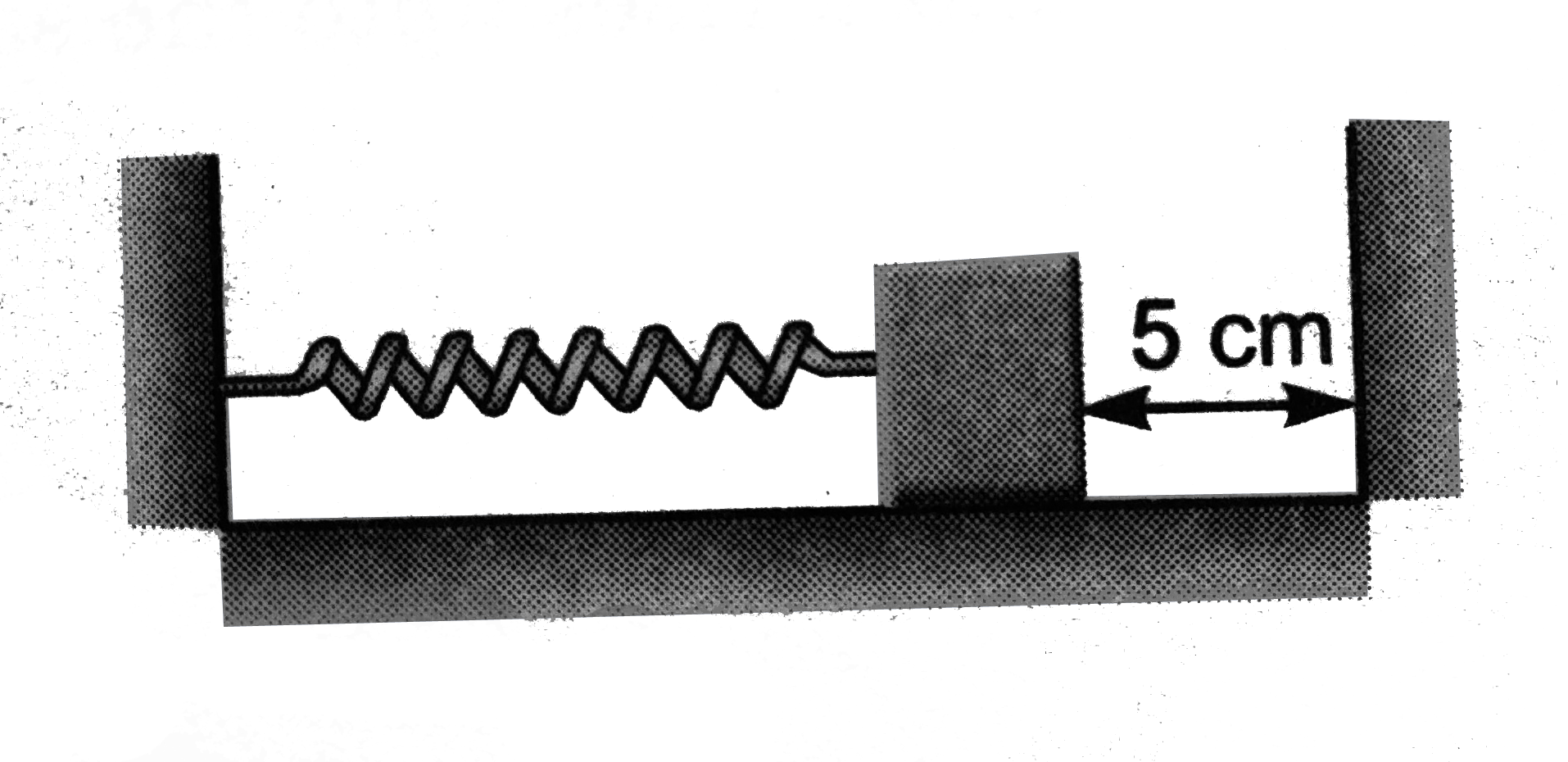
View SolutionA block of mass 100g attached to a spring of stiffness 100N/m is lying on a frictionless floor as shown. The block is moved to compress the spring by 10cm and released. If the collision with the wall is elastic then find the time period of oscillations.
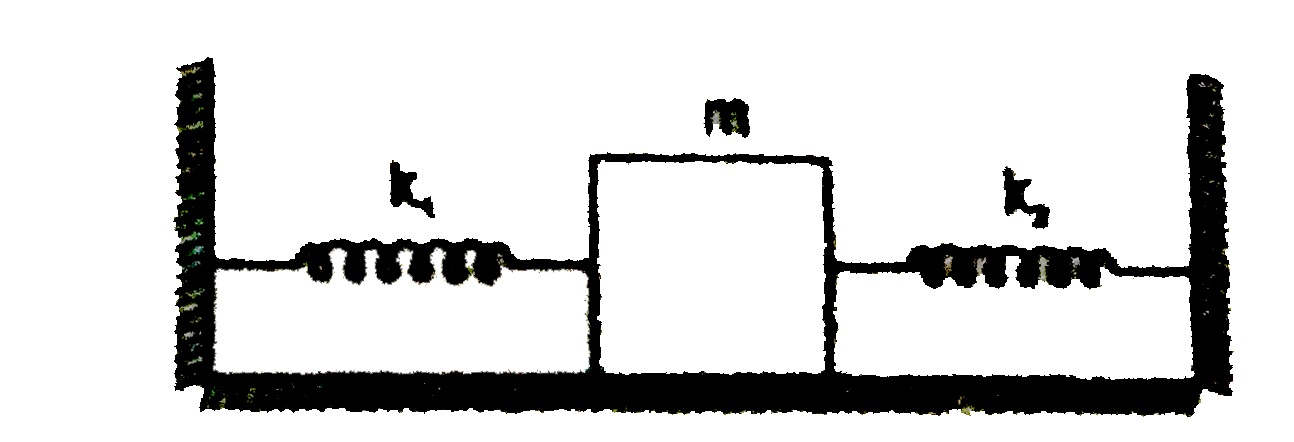
View SolutionA block of mass m is attached to two unstretched springs of springs constant k1 and k2 as shown in figure. The block is displaced towards right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.
View SolutionA block of mass m is connected to another block of mass M by a massless spring of spring constant K. Blocks are kept on a smooth horizontal plane. Initally both the blocks the at rest and spring is unstressed. Force F is applied on M to right as shownin the figure then which of the following is correct ?

View SolutionA block of mass M placed on a frictionless horizontal table is pulled by another block of mass m hanging vertically by a massless string passing over a frictionless pulley. The tension in the string is :
View SolutionA block of mass m is connected to a massless pulley and mass-less spring of stiffness k. The pulley is frictionless. The string is mass-less. Initially the spring is unstretched when the block is released. When the spring is maximum stretched, find the ratio of tenstion in the rope and weight of the block.

View SolutionA mas m is moving in SHM on a line with amplitude A and frequency f. suddenly half of the mass comes to rest just at the moment when it comes mean position then the new amplitude becomes λA, then λ will be
View Solution
