A short pˆi is placed at origin in x - y plane. The locus of equipotential surface around the dipole if a constant electric field Eˆi also exists through out the space is (k=14πε0)(Vorigin=0)

x2+y2+x2=(kpE)2/3
x2+y2=(kpE)2/3
x2+y2+z2=(kpE)1/3
none
The correct Answer is:A
Topper's Solved these Questions
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE SHEET (EXERCISE -V ELECTRIC DIPOLE) (LEVEL -II LINKED COMPREHENSION TYPE QUESTION)
2 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE SHEET (EXERCISE -V ELECTRIC DIPOLE) (LEVEL -II INTEGER TYPE QUESTION)
1 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE SHEET (EXERCISE -V ELECTRIC DIPOLE) (LEVEL - I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS)
4 Videos
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - ADDITIONAL EXERCISE
6 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
9 Videos
Similar Questions
Knowledge Check
Two short dipoles, each of diple moment p , are placed at origin. The dipole moment of one dipole is along x-axis, while that of other is along y-axis . The electric field at a point (a,0) is given by
A(14πε0)2pa3B(14πε0)pa3C(14πε0)√5pa3DZeroA dipole is placed at the origin such that its dipole moment is →p=−pˆi,0. The electric field and potential at (0,d,0) respectively are :
Ap4pε0d3ˆi,0B−p4pε0d3ˆi,0C2p4pε0d3ˆi,0D2p4pε0d3ˆi,0
Electric field function in a region of space is given as → E = y i + x j. The shape of equipotential surface is
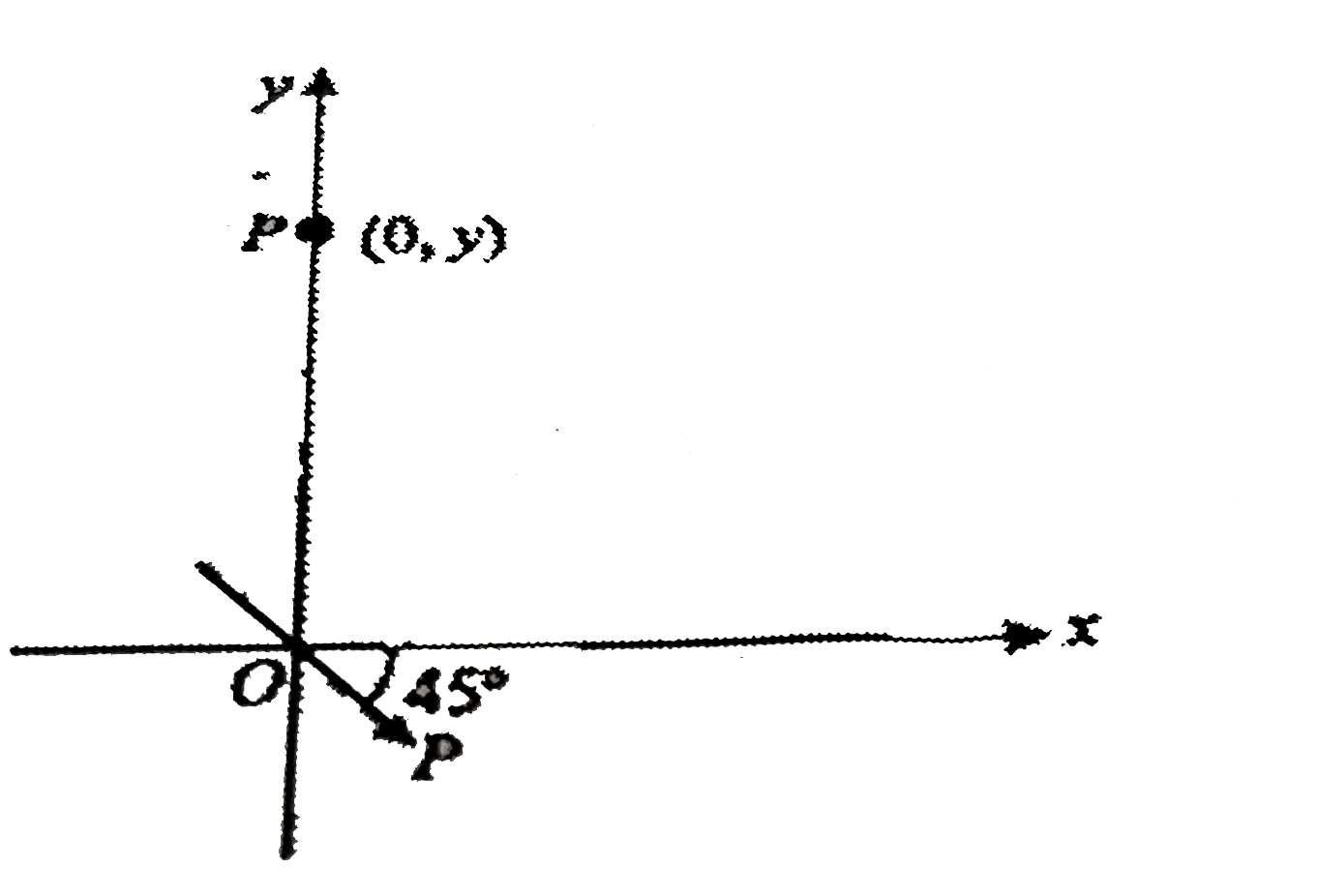
View SolutionA dipole is placed at origin of coordinate system as shown in figure, find the electric field at point P(0,y).
[KP√2y3(−ˆi−2ˆj)]
View SolutionTwo short dipoles, each of diple moment p , are placed at origin. The dipole moment of one dipole is along x-axis, while that of other is along y-axis . The electric field at a point (a,0) is given by
View SolutionA dipole of dipole moment p is placed at origin along x axis. Another dipole of dipole moment also p is kept at (0, 1, 0) along y axis. Find the resultant potential and electric field at (1, 0, 0).
View SolutionA dipole is placed at the origin such that its dipole moment is →p=−pˆi, The electric field and potential at (0,d,0) respectively are :
View SolutionTwo point dipoles pˆk and P2ˆk are located at (0, 0, 0) and (1m, 0, 2m) respectively. Find the resultant electric field due to the two dipoles at the point M(1m, 0, 0)
[−(7P32π∈0)ˆk]
View Solution

 .
.