A parallel plate capacitor has plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has a dielectric constant that varies as k(x)=K(1+ax) where ‘x’ is shown in figure. If (al)<<1 , the total capacitance of the system is best given by the expression:

AK∈0d(1+αl)
Ak∈0d(1+αl2)
A∈0Kd(1+(αl2)2)
A∈0Kd(1+α2l22)
The correct Answer is:B
dc=K∈0Aldxd
Ceq=∫(dc)=K∈0Alddx

−∈0Ald∫l0(1+αx)dx
=K∈0Ald(l+αl22) K∈0Ad(1+αl2)
Similar Questions
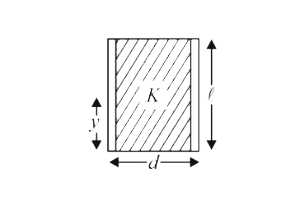
A parallel plate capacitor has square plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has a dielectric constant that varies as k(y)=K0(1+βy) where 'y' is the distance measured from bottom of capacitor. The total capacitance of the system is given by the expression:
View SolutionA parallel plate capacitor has plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has dielectric constant that varies as K(y)=k0(1+αy) where ‘y’ is the vertical distance measured from base of the plates. The total capacitance of the system is best given by: (K0 is constant)
View Solution
Knowledge Check
A parallel plate capacitor has plate area A and plate separation d. The space between the plates is filled up to a thickness x<d with a dielectric constant K. Calculate the capacitance of the system.
 A2KεAKd−x(K−1)BKεAK2d−x(K−1)CKεAK−x(K−1)DKεAKd−x(K−1)
A2KεAKd−x(K−1)BKεAK2d−x(K−1)CKεAK−x(K−1)DKεAKd−x(K−1)A parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then
 AC=C[KK+1]BC'=C[2KK+1]CC'=2CK+1DC'=C[1+K2]
AC=C[KK+1]BC'=C[2KK+1]CC'=2CK+1DC'=C[1+K2]
A parallel plate capacitor has plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has dielectric constant that varies as K(y)=k0(1+αy) where ‘y’ is the vertical distance measured from base of the plates. The total capacitance of the system is best given by: (K0 is constant)

View SolutionA parallel plate capacitor has plate area of A separated by distance between them. It is completely filled with dielectric medium, its dielectric constant increases linearly as move from one plate to other plate from k1 to k2 . Then the capacitance of system is given by :
View SolutionA parallel plate capacitor has plate area of A separated by distance between them. It is completely filled with dielectric medium, its dielectric constant increases linearly as move from one plate to other plate from k1 to k2 . Then the capacitance of system is given by :

View SolutionA parallel plate capacitor has plate area of A separated by distance between them. It is completely filled with dielectric medium, its dielectric constant increases linearly as move from one plate to other plate from k1 to k2 . Then the capacitance of system is given by :

View SolutionA parallel plate capacitor has plate area A and plate separation d. The space betwwen the plates is filled up to a thickness x (ltd) with a dielectric constant K. Calculate the capacitance of the system.

View SolutionA parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then

View Solution
